
- tvlrchess@gmail.com
- +91 9444751432
Law of Chess
FIDE ARBITERS’ COMMISSION

Dear chess friends,
It is a pleasure to follow the on-going successful work of the FIDE Arbiters' Commission in many aspects, including the online magazine for chess arbiters and the Arbiters’ Manual.
The Manual was launched several years ago as an instrument for arbiters and has proven to be of great help to National Federations, their respective Arbiters' councils and everybody in our chess community.
I am confident that this Manual will be instrumental in each Arbiter’s work and will facilitate and enrich his/her skills in order to exercise arbiter’s duties in the best way.
Commission’s daily work and brilliant organization of seminars, webinars and workshops has substantially increased the number and quality of chess arbiters throughout the world, including new Federations.
I l support and welcome the work and future plans of the Arbiters’ Commission and would like to wish all of you, first of all its Chairman and Councillors and all those who contribute to this tremendous work, lots of success, increasing audience and welltrained professional arbiters.
Gens Una Sumus
Georgios Makropoulos
FIDE Deputy President
A BRIEF HISTORY OF THE LAWS OF CHESS
FIDE was founded in Paris on 20 July 1924 and one of its main objectives was to unify the rules of the game. The first official rules for chess were published in 1929 in French.
An update of the rules was published (once more in French) in 1952 with the amendments by the FIDE General Assembly.
There was another edition in 1966, with comments to the rules. Finally, in 1974 the Permanent Rules Commission published the first English edition with new interpretations and some amendments. In the following years the FIDE Rules Commission made some more changes, based on experience from competitions.
Major change was made in 1997, when the ‘more or less’ actual Laws of Chess were split into three parts: The Basic Rules of Play, the Competition Rules and Appendices.
In 2016 the Laws of Chess were split in 5 parts: The Basic Rules of Play, the Competition Rules, the Appendices, the Guidelines and the Glossary of terms of the Laws of Chess
The first part - Articles 1 to 5 - is important for all people playing chess, including the basic rules that anyone who wants to play chess need to know.
The second part – Articles 6 to 12 - mainly applies to chess tournaments.
The third part includes some appendices for Rapid games, Blitz games, the Algebraic notation of the games and the rules for play with blind and visually disabled players
The fourth part includes guidelines for adjourned games, for Chess 960 games and for games without increment, including Quick play finishes.
The fifth part includes a glossary of terms of the laws of Chess.
Starting from 1997 the FIDE Rules Commission (RC) makes changes of the Laws of Chess only every four years, coming into force on 1st July of the year following the decision.
Let us finish the history with the prefaces of the 1958 and 1974 Rules of Chess: 1958
“GENERAL OBSERVATIONS. The Laws of Chess cannot, and should not, regulate all possible situations that may arise during a game, nor they can regulate all questions of organization. In most cases not precisely regulate by an Article of the Laws, one should be able to reach a correct judgment by applying analogous stipulations for situations of a similar character. As to the arbiters’ tasks, in most cases one must presuppose that arbiters have the competence, sound of judgment, and absolute objectivity necessary. A regulation too detailed would deprive the arbiter of his freedom of judgment and might prevent him from finding the solution dictated by fairness and compatible with the circumstances of a particular case, since one cannot foresee every possibility.”1974
“FIDE INTERPRETATIONS. During recent years the Commission has been more or less overwhelmed by a steadily growing number of proposals and questions. That, of itself, is a good thing. However, there is a marked tendency in those many questions and proposals to bring more and more refinements and details into the Laws of Chess. Clearly the intention is to get more and more detailed instructions concerning “how to act in such and such case”. This may be profitable for a certain type of arbiter, but at the same time may be a severe handicap for another, generally the best, type of arbiter. The Commission in it’s entirely takes the firm position that the laws of Chess should be as short and as clear as possible. The Commission strongly believes that minor details should be left to the discretion of the arbiter. Each arbiter should have the opportunity, in case of a conflict, to take into account all the factors of the case and should be not bound by too detailed sub‐regulations which may be not applicable to the case in question. According to the Commission, the Laws of Chess must be short and clear and leave sufficient scope to the arbiter to deal with exceptional or unusual cases. The Commissions appeals to all chess federations to accept this view, which is in the interest of the hundreds of thousands of chess players, as well as of the arbiters, generally speaking. If any chess federation wants to introduce more detailed rules, it is perfectly free to do so, provided:
-
- they do not in any way conflict with the official FIDE rules of play;
- they are limited to the territory of the federation in question; and
- they are not valid for any FIDE tournament played in the territory of the federation in question.”
ARBITERS’ MANUAL October 2022

ARBITERS’ MANUAL October 2022
FIDE President’s welcome
Dear Chess Friends,
The FIDE Arbiters' Commission represents one of the most numerous communities in the FIDE family, counting over 16,000 licensed arbiters, and plays an essential role in the development of chess all over the world.
In addition to the organization of seminars and workshops and to the regular publication on its website of guidelines and new content for all chess arbiters, an important achievement of the FIDE Arbiters' Commission is represented by the Arbiters' Manual. This document was created several years ago in the belief that it was important to have policies and procedures which could be easily accessible and of great assistance to the arbiters, the National Chess Federations, the players, and everyone who is involved in the organization of chess tournaments
The Arbiters' Manual has now been updated, and the readers will find in this book the new regulations and all necessary documents for chess arbiters to be guided in their daily activities by a team of the most experienced experts in this field. I sincerely hope that this book will be an essential tool for the training of future arbiters and will contribute to increasing the number of qualified professional chess arbiters in the world and to further enrich their skills in order to allow them to exercise their duties in the best possible way.
I am proud to express my deepest gratitude to the FIDE Arbiters' Commission and to everyone who contributed to the creation of this new issue of the Arbiters' Manual. I am also very glad that the updated Manual will be available to all arbiters of the 44th FIDE Chess Olympiad in Chennai, India.
Chess Guide In Tamil
|
“ We understand that you are having many doubts regarding the game, that’s why we are giving you an effective guide. You can find your answers from the guide, it is also available in Tamil. Let's play the game and enjoy yourself with us.” |
FIDE LAWS OF CHESS
INTRODUCTION
FIDE Laws of Chess cover over-the-board play. The Laws of Chess have two parts: 1. Basic Rules of Play and 2. Competition Rules.
The English text is the authentic version of the Laws of Chess (which were adopted at the 88th FIDE Congress at Antalya, Turkey) coming into force on 1 January 2018.
In these Laws the words ‘he’, ‘him’, and ‘his’ shall be considered to include ‘she’ and ‘her’.
PREFACE
The Laws of Chess cannot cover all possible situations that may arise during a game, nor can they regulate all administrative questions. Where cases are not precisely regulated by an Article of the Laws, it should be possible to reach a correct decision by studying analogous situations which are regulated in the Laws. The Laws assume that arbiters have the necessary competence, sound judgement and absolute objectivity. Too detailed a rule might deprive the arbiter of his freedom of judgement and thus prevent him from finding a solution to a problem dictated by fairness, logic and special factors. FIDE appeals to all chess players and federations to accept this view. A necessary condition for a game to be rated by FIDE is that it shall be played according to the FIDE Laws of Chess. It is recommended that competitive games not rated by FIDE be played according to the FIDE Laws of Chess.
Member federations may ask FIDE to give a ruling on matters relating to the Laws of Chess.
|
The Preface of the Laws is one of the most important parts. Of course, the Laws of Chess cannot cover all possible situations arising during a game. Sometimes only a small part of a situation is changed and the Arbiter can make a decision based mainly on the Laws of Chess. For cases, however that are not covered completely by the Laws, the arbiter has to make a decision based on analogous situations that have happened in the past, as well as based on common logic, fairness and probably special factors. But it is always necessary for an arbiter to make his decisions and to solve all problems during the game. Therefore, an excellent knowledge of the Laws of Chess and his experience, obtained from his working in tournaments, are the most important qualifications of an Arbiter, together with objectivity at all times. |
BASIC RULES OF PLAY
Article 1: The nature and objectives of the game of chess
-
- The game of chess is played between two opponents who move their pieces on a square board called a ‘chessboard’.
- The player with the light-coloured pieces (White) makes the first move, then the players move alternately, with the player with the dark-coloured pieces (Black) making the next move.
- A player is said to ‘have the move’ when his opponent’s move has been ‘made’.
- The objective of each player is to place the opponent’s king ‘under attack’ in such a way that the opponent has no legal move.
- The player who achieves this goal is said to have ‘checkmated’ the opponent’s king and to have won the game. Leaving one’s own king under attack, exposing one’s own king to attack and also ’capturing’ the opponent’s king is not allowed.
- The opponent whose king has been checkmated has lost the game.
- If the position is such that neither player can possibly checkmate the opponent’s king, the game is drawn (see Article 5.2.2).
|
Sometimes, neither white nor black can checkmate the opponent. In such a case, the game is drawn. The simplest example is when there are only the two kings on the chessboard. |
Article 2: The initial position of the pieces on the chessboard
-
- The chessboard is composed of an 8 x 8 grid of 64 equal squares alternately light (the ‘white’ squares) and dark (the ‘black’ squares).
The chessboard is placed between the players in such a way that the near corner square to the right of the player is white.
At the beginning of the game White has 16 light-coloured pieces (the ‘white’ pieces); Black has 16 dark-coloured pieces (the ‘black’ pieces).
These pieces are as follows:
- The chessboard is composed of an 8 x 8 grid of 64 equal squares alternately light (the ‘white’ squares) and dark (the ‘black’ squares).
A white king usually indicated by the symbol  K
K
A white queen usually indicated by the symbol  Q
Q
Two white rooks usually indicated by the symbol  R
R
Two white bishops usually indicated by the symbol  B
B
Two white knights usually indicated by the symbol  N
N
Eight white pawns usually indicated by the symbol 
A black king usually indicated by the symbol  K
K
A black queen usually indicated by the symbol  Q
Q
Two black rooks usually indicated by the symbol  R
R
Two black bishops usually indicated by the symbol  B
B
Two black knights usually indicated by the symbol  N
N
Eight black pawns usually indicated by the symbol 
Staunton Pieces

p Q K B N R
-
- The initial position of the pieces on the chessboard is as follows:

-
- The eight vertical columns of squares are called ‘files’. The eight horizontal rows of squares are called ‘ranks’. A straight line of squares of the same colour, running from one edge of the board to an adjacent edge, is called a ‘diagonal’.
|
A chessboard can be made of different materials, but the colour of the squares (dark = brown or black and light = white or cream) must be clearly different. It is useful that it is not shiny to avoid reflections and disturbance of players. The dimension of the chessboard must fit with the dimension of the pieces. (For more information see FIDE Handbook C.05 FIDE Tournament Rules). It is very important to check the orientation of the chessboard and the correct position of all the pieces before starting the game. By doing this, an arbiter can avoid a lot of possible claims about reversed Kings and Queens or Knights and Bishops. Sometimes, there is a disagreement between the players about how to place the knights. Each player has his own habits regarding this. Each player may place his own knights as he likes before the start of the game. He may only do so during the game when it is his move and after he has informed his opponent that he is going to adjust them (See Article 4: “J’adoube” – “I adjust”). |
Article 3: The moves of the pieces
-
- It is not permitted to move a piece to a square occupied by a piece of the same colour.
- If a piece moves to a square occupied by an opponent’s piece the latter is captured and removed from the chessboard as part of the same move.
- A piece is said to attack an opponent’s piece if the piece could make a capture on that square according to Articles 3.2 to 3.8.
- A piece is considered to attack a square even if this piece is constrained from moving to that square because it would then leave or place the king of its own colour under attack.
- It is not permitted to move a piece to a square occupied by a piece of the same colour.
|
Even if a piece is pinned against its own king, it attacks all the squares to which it would be able to move, if it were not pinned. |
-
- The bishop may move to any square along a diagonal on which it stands.

|
Initially, each player has two bishops, one of which moves on light squares, the other one on dark squares. If a player has two or more bishops on squares of the same colour, it must be that the second bishop is the result of a promotion (See article 3.7.5.1), or an illegal move was played. |
-
- The rook may move to any square along the file or the rank on which it stands.

-
- The queen may move to any square along the file, the rank or a diagonal on which it stands.

-
- When making these moves, the bishop, rook or queen may not move over any intervening pieces.
- The knight may move to one of the squares nearest to that on which it stands but not on the same rank, file or diagonal.

-
-
- The pawn may move forward to the square immediately in front of it on the same file, provided that this square is unoccupied, or
- on its first move the pawn may move as in 3.7.1 or alternatively it may advance two squares along the same file, provided that both squares are unoccupied, or
- the pawn may move to a square occupied by an opponent’s piece diagonally in front of it on an adjacent file, capturing that piece.
-

-
-
-
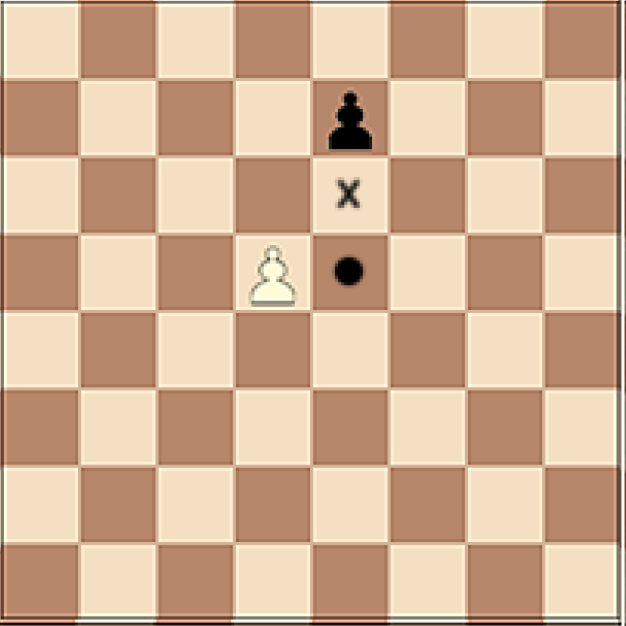
- A pawn occupying a square on the same rank as and on an adjacent file to an opponent’s pawn which has just advanced two squares in one move from its original square may capture this opponent’s pawn as though the latter had been moved only one square.
- This capture is only legal on the move following this advance and is called an ‘en passant’ capture.
-
-
-
-
-
- When a player, having the move, plays a pawn to the rank furthest from its starting position, he must exchange that pawn as part of the same move for a new queen, rook, bishop or knight of the same colour on the intended square of arrival. This is called the square of ‘promotion’.
- The player's choice is not restricted to pieces that have been captured previously.
- This exchange of a pawn for another piece is called promotion, and the effect of the new piece is immediate.
-
-
|
When a player places an inverted (upside – down) Rook on the promotion square and continues the game, the piece is considered as a Rook, even if he names it as a “Queen” or any other piece. If he moves the upside-down rook diagonally, it becomes an illegal move. In case of a promotion and if the player cannot find the required piece, he has the right to stop the game immediately and ask the Arbiter to bring him the piece he wants. Then the game continues after the promotion has been completed. |
-
- There are two different ways of moving the king:
- by moving to an adjoining square
- There are two different ways of moving the king:

-
-
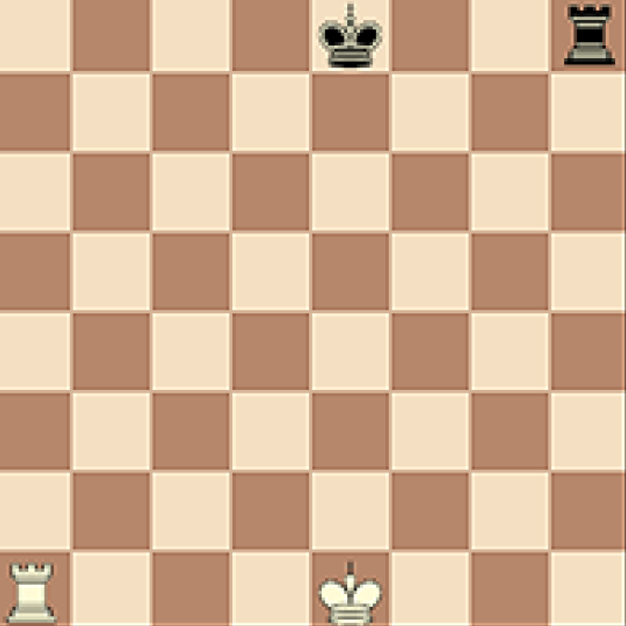
- by ‘castling’. This is a move of the king and either rook of the same colour along the player’s first rank, counting as a single move of the king and executed as follows: the king is transferred from its original square two squares towards the rook on its original square, then that rook is transferred to the square the king has just crossed.
-

Before white kingside castling / Before black queenside castling

After white kingside castling / After black queenside castling
Before white queenside castling / Before black kingside castling
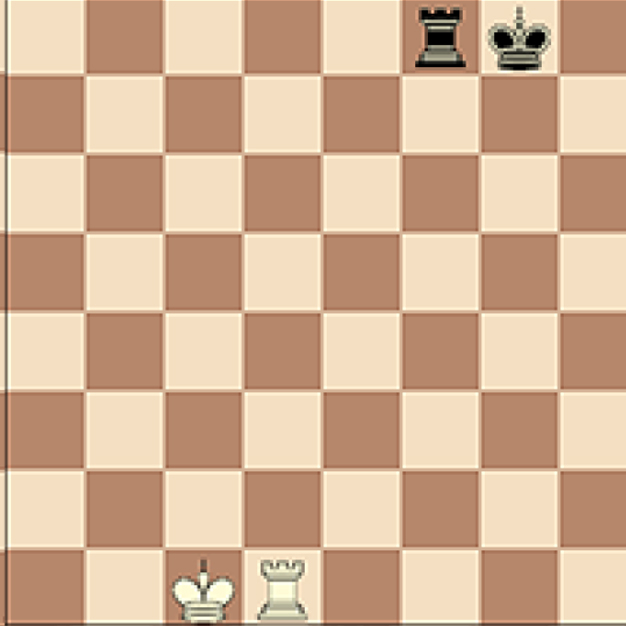
After white queenside castling / After black kingside castling
-
-
-
- The right to castle has been lost:
- if the king has already moved, or
- with a rook that has already moved.
- Castling is prevented temporarily:
- if the square on which the king stands, or the square which it must cross, or the square which it is to occupy, is attacked by one or more of the opponent's pieces, or
- if there is any piece between the king and the rook with which castling is to be effected.
- The right to castle has been lost:
- The king is said to be 'in check' if it is attacked by one or more of the opponent's pieces, even if such pieces are constrained from moving to the square occupied by the king because they would then leave or place their own king in check.
- No piece can be moved that will either expose the king of the same colour to check or leave that king in check.
-
-
-
-
- A move is legal when all the relevant requirements of Articles 3.1-3.9 have been fulfilled.
-
-
-
- A move is illegal when it fails to meet the relevant requirements of Articles 3.1-3.9
-
-
-
- A position is illegal when it cannot have been reached by any series of legal moves.
-
Article 4: The act of moving the pieces
4.1 Each move must be played with one hand only.
-
-
- Only the player having the move may adjust one or more pieces on their squares, provided that he first expresses his intention (for example by saying “j’adoube” or “I adjust”).
-
|
Article 4.2.1 may only be used to correct displaced pieces. Where the opponent is not present at the board, a player should inform the arbiter- if there is an arbiter present – before he starts to adjust the pieces on the chess board. |
-
-
- Any other physical contact with a piece, except for clearly accidental contact, shall be considered to be intent.
-
|
According to this rule, if a player has not said “I adjust” before touching a piece and touching the piece is not accidental, the touched piece must be moved. |
-
- Except as provided in Article 4.2, if the player having the move touches on the chessboard, with the intention of moving or capturing:
- one or more of his own pieces, he must move the first piece touched that can be moved
- one or more of his opponent’s pieces, he must capture the first piece touched that can be captured
- one or more pieces of each colour, he must capture the first touched opponent’s piece with his first touched piece or, if this is illegal, move or capture the first piece touched that can be moved or captured. If it is unclear whether the player’s own piece or his opponent’s was touched first, the player’s own piece shall be considered to have been touched before his opponent’s.
- If a player having the move:
- touches his king and a rook he must castle on that side if it is legal to do so
- deliberately touches a rook and then his king he is not allowed to castle on that side on that move and the situation shall be governed by Article 4.3.1
- intending to castle, touches the king and then a rook, but castling with this rook is illegal, the player must make another legal move with his king (which may include castling with the other rook). If the king has no legal move, the player is free to make any legal move.
- Except as provided in Article 4.2, if the player having the move touches on the chessboard, with the intention of moving or capturing:
-
-
- promotes a pawn, the choice of the piece is finalised when the piece has touched the square of promotion.
- If none of the pieces touched in accordance with Article 4.3 or Article 4.4 can be moved or captured, the player may make any legal move.
- The act of promotion may be performed in various ways:
- the pawn does not have to be placed on the square of arrival,
- removing the pawn and putting the new piece on the square of promotion may occur in any order.
- If an opponent’s piece stands on the square of promotion, it must be captured.
- When, as a legal move or part of a legal move, a piece has been released on a square, it cannot be moved to another square on this move. The move is considered to have been made in the case of:
- a capture, when the captured piece has been removed from the chessboard and the player, having placed his own piece on its new square, has released this capturing piece from his hand,
- castling, when the player's hand has released the rook on the square previously crossed by the king. When the player has released the king from his hand, the move is not yet made, but the player no longer has the right to make any move other than castling on that side, if this is legal. If castling on this side is illegal, the player must make another legal move with his king (which may include castling with the other rook). If the king has no legal move, the player is free to make any legal move.
- promotion, when the player's hand has released the new piece on the square of promotion and the pawn has been removed from the board.
- A player forfeits his right to claim against his opponent’s violation of Articles 4.1-4.7 once the player touches a piece with the intention of moving or capturing it.
-
-
- If a player is unable to move the pieces, an assistant, who shall be acceptable to the arbiter, may be provided by the player to perform this operation.
|
If an arbiter observes a violation of Article 4, he must always intervene immediately. He should not wait for a claim to be submitted by a player. |
Article 5: The completion of the game
-
-
- The game is won by the player who has checkmated his opponent’s king. This immediately ends the game, provided that the move producing the checkmate position was in accordance with Article 3 and Articles 4.2 – 4.7.
-
-
-
- The game is won by the player whose opponent declares he resigns. This immediately ends the game.
-
|
A player may resign in a number of different ways:
All of these possibilities are capable of being misinterpreted. Therefore the situation has to be clarified. A player who does not wish to continue a game and leaves without resigning – or notifying the arbiter – is being discourteous. He may be penalized, at the discretion of the Chief Arbiter, for poor sportsmanship. |
-
-
- The game is drawn when the player to move has no legal move and his king is not in check. The game is said to end in ‘stalemate’. This immediately ends the game, provided that the move producing the stalemate position was in accordance with Article 3 and Articles 4.2 – 4.7.
- The game is drawn when a position has arisen in which neither player can checkmate the opponent’s king with any series of legal moves. The game is said to end in a ‘dead position’. This immediately ends the game, provided that the move producing the position was in accordance with Article 3 and Articles 4.2 – 4.7.
- The game is drawn upon agreement between the two players during the game, provided both players have made at least one move. This immediately ends the game.
-
|
This rule is applicable, only if Article 9.1.1 (not to agree for a draw before a specified number of moves by each player) is not enforced. The best way to conclude a game is to write down the result on the score sheet (if there is any, see Article 8) and for both players to sign it. This then forms a legal document, but. even then, things can go wrong. |
COMPETITION RULES
Article 6: The chessclock
6.1 Chessclock’ means a clock with two time displays, connected to each other in such a way that only one of them can run at one time.
‘Clock’ in the Laws of Chess means one of the two time displays.
Each time display has a ‘flag’.
‘Flag-fall’ means the expiration of the allotted time for a player.
6.2.1 During the game each player, having made his move on the chessboard, shall stop his own clock and start his opponent’s clock (that is to say, he shall press his clock). This “completes” the move. A move is also completed if:
6.2.1.1 the move ends the game (see Articles 5.1.1, 5.2.1, 5.2.2, 9.6.1 and 9.6.2), or
6.2.1.2 the player has made his next move, when his previous move was not completed.
6.2.2 A player must be allowed to stop his clock after making his move, even after the opponent has made his next move. The time between making the move on the chessboard and pressing the clock is regarded as part of the time allotted to the player.
Some digital clocks show “ – “ instead of a flag.
Normally, when the player forgets to press his clock after making his move, the opponent has the following possibilities: (a) To wait for the player to press his clock. In this case there is a possibility to have a flag fall and the player to lose on time. Some may think that this is quite unfair, but the Arbiter cannot intervene and inform the player. (b) To remind the player to press his clock. In this case the game will continue normally. (c) To make his next move. In this case the player can also make his next move and press his clock. If the game is played with move counter, then one move has been missed by both players. In such a situation the Arbiter should, at an appropriate moment, (when he is sure it will not cause much distraction) intervene and press the lever of the clock once for each player (thus adding one move on the clock for each player). In this way the additional time of the next period (in case there is any) will be added properly (after move the first time control, usually move 40, has been completed).
6.2.3 A player must press his clock with the same hand with which he made his move. It is forbidden for a player to keep his finger on the clock or to ‘hover’ over it.
6.2.4 The players must handle the chessclock properly. It is forbidden to press it forcibly, to pick it up, to press the clock before moving or to knock it over. Improper clock handling shall be penalised in accordance with Article 12.9.
6.2.5 Only the player whose clock is running is allowed to adjust the pieces.
6.2.6 If a player is unable to use the clock, an assistant, who must be acceptable to the arbiter, may be provided by the player to perform this operation. His clock shall be adjusted by the arbiter in an equitable way. This adjustment of the clock shall not apply to the clock of a player with a disability.
6.3.1 When using a chessclock, each player must complete a minimum number of moves or all moves in an allotted period of time including any additional amount of time with each move. All these must be specified in advance.
The following situation may happen: A player makes a move, forgets to press the clock and leaves the table (for example to go to the toilet). After he returns he sees that his clock is running and believing that his opponent has completed his move he makes another move and presses the clock. In this situation the Arbiter must be summoned immediately to clarify the situation (did the opponent make a move or not?) and make the necessary corrections on the clock and the board.
A game may have more than one period. The requirements of the allotted number of moves and the additional amount of time with each move for each period must be specified in advance. These parameters should not change during a tournament. But, if there is a playoff, it is likely they will then change.
Sometimes the following happens: A player displaces some pieces. The opponent keeps his finger on the clock button to prevent the player pressing his clock. This is forbidden according to this Article.
If a player makes a move with one hand and presses the clock with the other, it is not considered as an illegal move, but it is penalized according to the article 12.9.
Where a player presses the clock without making a move, as mentioned in the article 6.2.4, it is considered as an illegal move and it is penalized according to the article 7.5.3.
It is clear that the player himself has to provide an assistant. He has to introduce this assistant in time to the arbiter, not just before the round. It is usual that 10 minutes are deducted from the time of the player who needs an assistant. No deduction should be made in the case of a disabled player. An example where a player is unable to use the clock is for religious reasons.
6.3.2 The time saved by a player during one period is added to his time available for the next period, where applicable.
In the time-delay mode both players receive an allotted ‘main thinking time’. Each player also receives a ‘fixed extra time’ with every move. The countdown of the main thinking time only commences after the fixed extra time has expired. Provided the player presses his clock before the expiration of the fixed extra time, the main thinking time does not change, irrespective of the proportion of the fixed extra time used.
6.4 Immediately after a flag falls, the requirements of Article 6.3.1 must be checked.
6.5 Before the start of the game the arbiter shall decide where the chessclock is placed.
1. Cumulative (Fischer) mode: Here each player has a main thinking time and receives a fixed extra time (increment) for each move. This increment for his first move is added before the game starts and then immediately after he has completed each of his following moves. If a player completes his move before the remaining time of this increment for the move expires, this remaining time will have been added to the main thinking time. 2. Bronstein mode: The main difference between Fisher mode and Bronstein mode is the handling of the extra time. If the player does not use the whole extra time in Bronstein mode, the remaining part is lost. 3. Time delay mode: Each player receives a main thinking time. When a player has the move the clock will not start counting for a fixed period. After this period expired the clock is counting down the main playing time.
This means that the arbiter and/or the players have to check if the minimum numbers of moves have been completed. Consider a game 90 minutes for 40 moves and 30 minutes for the rest of the game. It is normal to investigate whether 40 moves have been made by both players only after a flag has fallen. Unless a digital board is used this when the arbiter may determine the number of moves played from time.
If a move (push) counter is used in a digital clock, then it is possible to establish whether 40 moves have been made before a flag fall, as a “-“ indication appears on the clock in case that the player does not complete the 40 moves before the allotted time. In the majority of the top tournaments the move counter is used.
Where electronic clocks are used and both clocks show 0.00, the Arbiter can usually establish which flag fell first, with the help of the “-“ or any other flag indication. Where mechanical clocks are used then article III.3.1 of the Guidelines about games without increment including Quickplay Finishes are applied.
6.6 At the time determined for the start of the game White’s clock is started.
6.7.1 The regulations of an event shall specify a default time in advance. If the default time is not specified, then it is zero. Any player who arrives at the chessboard after the default time shall lose the game unless the arbiter decides otherwise.
6.7.2 If the regulations of an event specify that the default time is not zero and if neither player is present initially, White shall lose all the time that elapses until he arrives, unless the regulations of an event specify or the arbiter decides otherwise.
6.8 A flag is considered to have fallen when the arbiter observes the fact or when either player has made a valid claim to that effect.
In individual tournaments the chess clock is normally placed on the right side of the player who has the black pieces. The chess boards shall be placed so that the arbiter is able to check as many clocks as possible at the same time. In the case of a left-handed player with black pieces, the arbiter might arrange for the players to sit on the other side of the board. In team competitions the members of the same team usually sit in a row. Then the pieces are set alternate black and white and the clocks all point the same way. Be careful! It happens quite often in team competitions that a player presses the clock of his neighbour.
In smaller tournaments the arbiters start all clocks. In tournaments with many players the arbiter announces the start of the round and states that White’s clock is started. The arbiter then goes round the room checking that White’s clock has been started on all boards. Where the push counter is used to add time after the first time control (often 40 moves), it is desirable for arbiters to start all White’s clocks.
The start of the session is the moment, when the arbiter announces it. If the default time is 0, the arbiter has to declare the game lost for the players who are not present at their chessboards. It is preferable to install a large digital countdown device in the playing hall. For FIDE events with fewer than 30 players an appropriate announcement must be made five minutes before the round is due to start and again one minute before start of the game. Alternatively, a clock should be on the wall inside the playing hall and provide the official time of the tournament.
If the default time is not 0, it is advisable that the arbiter publicly announces the time of the start of the round and that he writes down the starting time. If the default time is for example 30 minutes and the round was scheduled to start at 15.00, but actually started at 15.15, then any player who hasn’t arrived by 15.45 loses.
A flag is considered to have fallen when it is noticed or claimed, not when it physically happened.
6.9 Except where one of Articles 5.1.1, 5.1.2, 5.2.1, 5.2.2, 5.2.3 applies, if a player does not complete the prescribed number of moves in the allotted time, the game is lost by that player. However, the game is drawn if the position is such that the opponent cannot checkmate the player’s king by any possible series of legal moves.
6.10.1 Every indication given by the chessclock is considered to be conclusive in the absence of any evident defect. A chessclock with an evident defect shall be replaced by the arbiter, who shall use his best judgement when determining the times to be shown on the replacement chessclock.
6.10.2 If during a game it is found that the setting of either or both clocks is incorrect, either player or the arbiter shall stop the chessclock immediately. The arbiter shall install the correct setting and adjust the times and move-counter, if necessary. He shall use his best judgement when determining the clock settings.
6.11.1 If the game needs to be interrupted, the arbiter shall stop the chessclock.
6.11.2 A player may stop the chessclock only in order to seek the arbiter’s assistance, for example when promotion has taken place and the piece required is not available.
6.11.3 The arbiter shall decide when the game restarts.
6.11.4 If a player stops the chessclock in order to seek the arbiter’s assistance, the arbiter shall determine whether the player had any valid reason for doing so. If the player had no valid reason for stopping the chessclock, the player shall be penalised in accordance with Article 12.9.
It means that a simple flag fall might not lead the Arbiter to declare the game lost for the player whose flag has fallen. The Arbiter has to check the final position on the chessboard and only if the opponent can checkmate the player’s king by any possible series of legal moves, can he declare the game won by the opponent. In case there are forced moves that lead to a checkmate by the player or to a stalemate, then the result of the game is declared as a draw.
It is desirable to check the clocks during the round, for instance every 30 minutes, and to record the times and the number of moves made, by using a time-control sheet (see at the end of the Manual). This can be particularly valuable when an increment is used. If a chess clock must be replaced, it must be done as soon as possible and it is essential to mark it as defective and to separate it from the clocks that work correctly.
It is essential to write down all the known details of the two clocks before making any adjustment.
For example, if a fire alarm goes off.
6.12.1 Screens, monitors, or demonstration boards showing the current position on the chessboard, the moves and the number of moves made/completed, and clocks which also show the number of moves, are allowed in the playing hall.
6.12.2 The player may not make a claim relying only on information shown in this manner.
Article 7: Irregularities
7.1 If an irregularity occurs and the pieces have to be restored to a previous position, the arbiter shall use his best judgement to determine the times to be shown on the chessclock. This includes the right not to change the clock times. He shall also, if necessary, adjust the clock’s move-counter.
7.2.1 If during a game it is found that the initial position of the pieces was incorrect, the game shall be cancelled and a new game shall be played.
7.2.2 If during a game it is found that the chessboard has been placed contrary to Article 2.1, the game shall continue but the position reached must be transferred to a correctly placed chessboard.
7.3 If a game has started with colours reversed then, if less than 10 moves have been made by both players, it shall be discontinued and a new game played with the correct colours. After 10 moves or more, the game shall continue.
7.4.1 If a player displaces one or more pieces, he shall re-establish the correct position in his own time.
A player may stop the clocks if he feels disturbed by his opponent or by spectators or is unwell. Going to the toilet is not necessarily a valid reason for stopping the clocks. A disabled player must be treated with due respect.
An arbiter or player must realise that the information displayed may be incorrect.
Be aware that the error was found during and not after the game. It is not mentioned how the mistake was found or who found it. If a game is played on an electronic chessboard, it can happen that the computer stops recording the moves. In such cases the operator should inform the arbiter that something has gone wrong and it is the arbiter’s duty to check what has happened.
After 10 moves or more the game shall continue, otherwise, a new game shall be played with the correct colours. It doesn’t matter what the current position on the chessboard is and how many pieces or pawns have been captured by the ninth move.
If a game with reversed colours has ended by normal means (for example checkmate, resignation or draw agreement), in less than ten (10) moves, then the result stands.
7.4.2 If necessary, either the player or his opponent shall stop the chessclock and ask for the arbiter’s assistance.
7.4.3 The arbiter may penalise the player who displaced the pieces.
7.5.1 An illegal move is completed once the player has pressed his clock. If during a game it is found that an illegal move has been completed, the position immediately before the irregularity shall be reinstated. If the position immediately before the irregularity cannot be determined, the game shall continue from the last identifiable position prior to the irregularity. Articles 4.3 and 4.7 apply to the move replacing the illegal move. The game shall then continue from this reinstated position.
7.5.2 If the player has moved a pawn to the furthest distant rank, pressed the clock, but not replaced the pawn with a new piece, the move is illegal. The pawn shall be replaced by a queen of the same colour as the pawn.
7.5.3 If the player presses the clock without making a move, it shall be considered and penalized as if an illegal move.
7.5.4 If a player uses two hands to make a single move (for example in case of castling, capturing or promotion) and pressed the clock, it shall be considered and penalized as if an illegal move.
7.5.5 After the action taken under Article 7.5.1, 7.5.2, 7.5.3 or 7.5.4 for the first completed illegal move by a player, the arbiter shall give two minutes extra time to his opponent; for the second completed illegal move by the same player the arbiter shall declare the game lost by this player. However, the game is drawn if the position is such
The Arbiter must be very careful here. Suppose player A has the move and his clock is running. Then player B displaces one of his own pieces (by accident). It is not correct that player A starts player B’s clock. A should stop both clocks and summon the arbiter. This Article should be applied flexibly.
Most problems happen in Rapidplay or Blitz. The penalty should be according to Article 12.9. A player should not be forfeited immediately for accidentally displacing a piece. If he did it deliberately, perhaps in order to gain time, or does it several times, that is different.
It is very important that the irregularity must be discovered during the game. After the players have signed the scoresheets or it is clear in another way that the game is over, corrections are not possible. The result stands. Where the irregularity is discovered during the game, it is important, that the game continues by moving the piece with which the illegal move was played or that the piece which was taken is taken by another piece, if possible.
A move cannot be declared illegal until the player completed his move by stopping his clock. So, the player can correct his move without being penalized, even if he had already released the piece on the board, provided he hasn’t press the clock. Of course, he must comply with relevant parts of article 4. that the opponent cannot checkmate the player’s king by any possible series of legal moves.
7.6 If, during a game it is found that any piece has been displaced from its correct square, the position before the irregularity shall be reinstated. If the position immediately before the irregularity cannot be determined, the game shall continue from the last identifiable position prior to the irregularity. The game shall then continue from this reinstated position.
Article 8: The recording of the moves
8.1.1 In the course of play each player is required to record his own moves and those of his opponent in the correct manner, move after move, as clearly and legibly as possible, in the algebraic notation (Appendix C), on the ‘scoresheet’ prescribed for the competition.
8.1.2 It is forbidden to write the moves in advance, unless the player is claiming a draw according to Article 9.2, or 9.3 or adjourning a game according to Guidelines I.1.1
8.1.3 A player may reply to his opponent’s move before recording it, if he so wishes. He must record his previous move before making another.
8.1.4 The scoresheet shall be used only for recording the moves, the times of the clocks, offers of a draw, matters relating to a claim and other relevant data.
8.1.5 Both players must record the offer of a draw on the scoresheet with a symbol (=).
8.1.6 If a player is unable to keep score, an assistant, who must be acceptable to the arbiter, may be provided by the player to write the moves. His clock shall be adjusted by the arbiter in an equitable way. This adjustment of the clock shall not apply to a player with a disability.
It is advisable that the investigation to determine from which position the game shall be continued takes place by the two players and under the supervision of the arbiter.
The player is forfeited in case he completes two (2) of ANY of the above illegal moves. However when there are two (2) illegal moves in one move (for example illegal castling made by two hands, illegal promotion made by two hands and illegal capturing made by two hands), they count as one (1) illegal move and the player shall not be forfeited, unless it is the second such transgression.
Capturing of the opponent’s King is illegal and is penalized accordingly.
8.2 The scoresheet shall be visible to the arbiter throughout the game.
8.3 The scoresheets are the property of the organiser of the competition.
8.4 If a player has less than five minutes left on his clock at some stage in a period and does not have additional time of 30 seconds or more added with each move, then for the remainder of the period he is not obliged to meet the requirements of Article 8.1.1.
8.5.1 If neither player keeps score under Article 8.4, the arbiter or an assistant should try to be present and keep score. In this case, immediately after a flag has fallen the arbiter shall stop the chessclock. Then both players shall update their scoresheets, using the arbiter’s or the opponent’s scoresheet.
8.5.2 If only one player has not kept score under Article 8.4, he must, as soon as either flag has fallen, update his scoresheet completely before moving a piece on the chessboard. Provided it is that player’s move, he may use his opponent’s scoresheet, but must return it before making a move.
8.5.3 If no complete scoresheet is available, the players must reconstruct the game on a second chessboard under the control of the arbiter or an assistant. He shall first record the actual game position, clock times, whose clock was running and the number of
Notice that it is forbidden to record the move in advance. Only in case of a draw claim (Article 9.2. and 9.3) and adjourning is it allowed. It is permitted to record the moves as a pair (his opponent’s move and his own), but he must have recorded his previous own move before making his next move. Even if an opponent has only one legal response, this must not be recorded by the player in advance.
Nowadays there are generally no problems with this Article. The habit of concealing the move written on the score sheet moves with a pen does not violate this article. But still the arbiter has the right to remove the pen from the score sheet, whenever he wants to check the number of the moves played by the players, provided it does not distract the opponent.
A player is not allowed to keep the original score sheet. It belongs to the Organisers. The player has to deliver it to the arbiter when the game is finished and should keep a copy (if any).
It happens quite often that in this time trouble phase the player asks the arbiter how many moves are left until the time control. The arbiter shall never give any information about the number of the moves that have been made, even after a player or both players have completed the required number of moves. The arbiter should act, only after a flag fall. He stops both clocks and orders the players to update their scoresheets. The arbiter shall start the clock of the player who has the move, only after they have done this. A player rarely takes too long over this, sometimes contemplating his next move. Then he should be warned.
Notice that, in this situation, after a flag fall, the arbiter does not stop the clocks.moves made/completed, if this information is available, before reconstruction takes place.
8.6 If the scoresheets cannot be brought up to date showing that a player has overstepped the allotted time, the next move made shall be considered as the first of the following time period, unless there is evidence that more moves have been made or completed.
8.7 At the conclusion of the game both players shall sign both scoresheets, indicating the result of the game. Even if incorrect, this result shall stand, unless the arbiter decides otherwise.
Article 9: The drawn game
9.1.1 The regulations of an event may specify that players cannot offer or agree to a draw, whether in less than a specified number of moves or at all, without the consent of the arbiter.
9.1.2 However, if the regulations of an event allow a draw agreement the following shall apply:
The reconstruction should take place after both clocks have been stopped and should be done away from the other games, so as not to disturb them.
It is very important for the Arbiter to record the correct result of the games. At the moment the Arbiter sees that a game has been finished, he should go to that board and check if the players have recorded the result of the game and signed both scoresheets. The arbiter should immediately check that both score sheets show the same result.
This article allows the Chief Arbiter to overrule decisions made by other arbiters, even after the players have signed the scoresheets or the match protocols. It has also been known for both players to record the wrong result. This permits the arbiter to correct such errors.
If a competition applies this rule, then the mentioned number of moves or the no agreement at all, should be communicated with the players in the invitation to the tournament. It is advisable for the Arbiter to repeat the rule before the start of the tournament. It is clear that the rule applies only for draw agreements. Articles 9.2, 9.3 and 9.6 still apply during the whole game and give the possibility to the players to have a draw in less that the specified number of moves, which must be accepted by the Arbiter. For example, if two players make a draw by three-fold repetition after 20 moves, in a tournament where there is a draw restriction rule before 30 moves have been completed by both players, then the Arbiter must allow the draw. If neither player claims a draw by three-fold repetition, so that the repetition takes place five times, then the arbiter must step in and declare the game drawn, see 9.6.1.
9.1.2.1 A player wishing to offer a draw shall do so after having made a move on the chessboard and before pressing his clock. An offer at any other time during play is still valid but Article 11.5 must be considered. No conditions can be attached to the offer. In both cases the offer cannot be withdrawn and remains valid until the opponent accepts it, rejects it orally, rejects it by touching a piece with the intention of moving or capturing it, or the game is concluded in some other way.
9.1.2.2 The offer of a draw shall be noted by each player on his scoresheet with the symbol (=).
9.1.2.3 A claim of a draw under Article 9.2 or 9.3 shall be considered to be an offer of a draw.
9.2.1 The game is drawn, upon a correct claim by a player having the move, when the same position for at least the third time (not necessarily by a repetition of moves):
9.2.1.1 is about to appear, if he first writes his move, which cannot be changed, on his scoresheet and declares to the arbiter his intention to make this move, or
9.2.1.2 has just appeared, and the player claiming the draw has the move.
9.2.2 Positions are considered the same if and only if the same player has the move, pieces of the same kind and colour occupy the same squares and the possible moves of all the pieces of both players are the same. Thus positions are not the same if:
9.2.2.1 at the start of the sequence a pawn could have been captured en passant
This is a valuable rule for the arbiter and its use should be encouraged.
The correct sequence of a draw offer is clear: 1. making a move 2. offering of a draw 3. pressing the clock. If a player deviates from this order, the offer still stands though it has been offered in an incorrect manner. The arbiter in this case has to penalise the player, according to the Article 12.9. No conditions can be attached to a draw offer. Some examples: The player requires the opponent to accept the offer within 2 minutes. In a team competition: a draw is offered under the condition that another game in the match shall be resigned or shall be drawn as well. In both cases the offer of a draw is valid, but not the attached condition. Regarding 9.1.2.3: If a player claims a draw, the opponent has the possibility to agree immediately to the draw. In this case the arbiter does not need to check the correctness of the claim. But be careful. If there is a draw restriction (for example: no draw offers are allowed before 30 moves have been completed by both players) and the claim has been submitted before that move (perhaps after 28 moves), then the claim has to be checked by the Arbiter, even if the opponent would agree to the draw.
9.2.2.2 a king had castling rights with a rook that has not been moved, but forfeited these after moving. The castling rights are lost only after the king or rook is moved.
9.3 The game is drawn, upon a correct claim by a player having the move, if:
9.3.1 he writes his move, which cannot be changed, on his scoresheet and declares to the arbiter his intention to make this move which will result in the last 50 moves by each player having been made without the movement of any pawn and without any capture, or
9.3.2 the last 50 moves by each player have been completed without the movement of any pawn and without any capture.
9.4 If the player touches a piece as in Article 4.3, he loses the right to claim a draw under Article 9.2 or 9.3 on that move.
9.5.1 If a player claims a draw under Article 9.2 or 9.3, he or the arbiter shall stop the chessclock (see Article 6.12.1 or 6.12.2). He is not allowed to withdraw his claim.
9.5.2 If the claim is found to be correct, the game is immediately drawn.
9.5.3 If the claim is found to be incorrect, the arbiter shall add two minutes to the opponent’s remaining thinking time. Then the game shall continue. If the claim was based on an intended move, this move must be made in accordance with Articles 3 and 4.
9.6 If one or both of the following occur(s) then the game is drawn:
9.6.1 the same position has appeared, as in 9.2.2 at least five times.
9.6.2 any series of at least 75 moves have been made by each player without the movement of any pawn and without any capture. If the last move resulted in checkmate, that shall take precedence.
Only the player whose move it is, and whose clock is running, is allowed to claim a draw in this way.
The correctness of a claim must be checked in the presence of both players. It is also advisable to replay the game and not to decide by only using the score sheets. If electronic boards are used it is possible to check it on the computer.
See comment to article 9.2.
The player loses his right to claim a draw only on that move. He has always the possibility to make a new claim in the game later, provided the circumstances haven’t changed.
This claim is not treated as an illegal move.
It is mentioned that the intended move must be played, but if the intended move is illegal, another move with this piece must be made. All the other details of Article 4 are also valid.
Article 10: Points
10.1 Unless the regulations of an event specify otherwise, a player who wins his game, or wins by forfeit, scores one point (1), a player who loses his game, or forfeits, scores no points (0), and a player who draws his game scores a half point (½).
10.2 The total score of any game can never exceed the maximum score normally given for that game. Scores given to an individual player must be those normally associated with the game, for example a score of ¾ - ¼ is not allowed.
Article 11: The conduct of the players
11.1 The players shall take no action that will bring the game of chess into disrepute.
11.2.1 The ‘playing venue’ is defined as the ‘playing area’, rest rooms, toilets, refreshment area, area set aside for smoking and other places as designated by the arbiter.
11.2.2 The playing area is defined as the place where the games of a competition are played.
11.2.3 Only with the permission of the arbiter can:
11.2.3.1 a player leave the playing venue,
11.2.3.2 the player having the move be allowed to leave the playing area.
11.2.3.3 a person who is neither a player nor arbiter be allowed access to the playing area.
In 9.6.1 case, the five times need not be consecutive. In both 9.6.1 and 9.6.2 cases the Arbiter must intervene and stop the game, declaring it as a draw.
Another scoring system from time to time used is for a win 3 points, for a draw 1 point and for a lost game 0 points. The idea is to encourage more positive play. Another is win 3 points, draw 2, loss 1 and forfeit 0. This is to discourage forfeits and may encourage children particularly as they gain a point despite losing. Yet another is win 2, draw 1, loss 0. This avoids ½ on the results sheet.
This is an Article which can be used for any infringements that are not specifically mentioned in the articles of the Laws of Chess.
If possible, spectators should not enter the playing area. It is advisable to have all other rooms (smoking areas, toilets, refreshment areas, and so on.) always to be under the control of the Arbiters or assistants.
11.2.4 The regulations of an event may specify that the opponent of the player having a move must report to the arbiter when he wishes to leave the playing area.
11.3.1 During play the players are forbidden to use any notes, sources of information or advice, or analyse any game on another chessboard.
11.3.2.1 During a game, a player is forbidden to have any electronic device not specifically approved by the arbiter in the playing venue.
However, the regulations of an event may allow such devices to be stored in a player’s bag, provided the device is completely switched off. This bag must be placed as agreed with the arbiter. Both players are forbidden to use this bag without permission of the arbiter.
11.3.2.2 If it is evident that a player has such a device on their person in the playing venue, the player shall lose the game. The opponent shall win. The regulations of an event may specify a different, less severe, penalty.
11.3.3 The arbiter may require the player to allow his clothes, bags, other items or body to be inspected, in private. The arbiter or person authorised by the arbiter shall inspect the player, and shall be of the same gender as the player. If a player refuses to cooperate with these obligations, the arbiter shall take measures in accordance with Article 12.9.
This article should not be confused with Articles 11.2.3.1 and 11.2.3.2. In 11.2.3.1 it is prohibited for any player to leave the playing venue without the permission of the arbiter and in 11.2.3.2 it is prohibited to leave the playing area for the player having the move. But in 11.2.4 it is possible to include, in the regulations, prohibition of the opponent leaving the playing area without permission of the arbiter.
The regulations about electronic devices are now very strict. No mobile phone is allowed in the playing venue and it makes no difference if it is switched on or off. If a mobile phone (even switched off) is found with a player, his game is immediately lost and the opponent shall win. The result shall be 1-0 or 0-1. It doesn’t matter if, when the mobile phone is found, the opponent cannot checkmate the offending player by any series of legal moves: he wins the game. The opponent may have cheated earlier. It is different if the game has not yet started. Suppose the following situation occurs: There is no zero‐tolerance. Player A is in the playing hall at the start of the round. His opponent, Player B is absent. Immediately after player A made his first move his mobile rings. The arbiter declares the game lost for Player A. Some minutes later, but still on time, Player B arrives. The score is “‐/+”, it is not a “played” game and it cannot be rated.
11.3.4 Smoking, including e-cigarettes, is permitted only in the section of the venue designated by the arbiter.
11.4 Players who have finished their games shall be considered to be spectators.
11.5 It is forbidden to distract or annoy the opponent in any manner whatsoever. This includes unreasonable claims, unreasonable offers of a draw or the introduction of a source of noise into the playing area.
11.6 Infraction of any part of Articles 11.1 – 11.5 shall lead to penalties in accordance with Article 12.9.
11.7 Persistent refusal by a player to comply with the Laws of Chess shall be penalised by loss of the game. The arbiter shall decide the score of the opponent.
11.8 If both players are found guilty according to Article 11.7, the game shall be declared lost by both players.
11.9 A player shall have the right to request from the arbiter an explanation of particular points in the Laws of Chess.
However, there is the possibility for an arbiter or an organizer to specify in advance (in the regulations of the event) a less severe penalty for a violation of this article (perhaps a fine) They can also include in the regulations of the event the possibility of bringing such a device to the tournament provided that certain conditions are fulfilled: that it is completely switched off and stored in a separate bag, so that it is not in contact with the player and the player does not have access to the bag during the game, without the arbiter's permission (and he cannot take the bag with him to the toilet, and so on.).
If possible, this smoking area should be close to the playing area and supervised by an Arbiter or an Assistant.
It means that the players, who finished their games, have to leave the playing area. Nevertheless, give them a few minutes to watch the other boards, making sure they do not disturb players still in play.
Even if the draw offers or claims are quite reasonable, repeating them too often can annoy the opponent. The Arbiter must always intervene when the opponent is disturbed or distracted.
It is very difficult to give a general guideline for the application of this Article, but if an arbiter has to warn the player for the third or fourth time, this is a good reason to declare the game lost. It is necessary to inform the player that Article 11.7 shall be applied at the next infringement.
11.10 Unless the regulations of an event specify otherwise, a player may appeal against any decision of the arbiter, even if the player has signed the scoresheet (see Article 8.7).
11.11 Both players must assist the arbiter in any situation requiring reconstruction of the game, including draw claims.
11.12 Checking three times occurrence of the position or 50 moves claim is a duty of a the players, under supervision of the arbiter.
Article 12: The role of the Arbiter (see Preface)
12.1 The arbiter shall see that the Laws of Chess are observed.
12.2 The arbiter shall:
12.2.1 ensure fair play,
12.2.2 act in the best interest of the competition,
12.2.3 ensure that a good playing environment is maintained,
12.2.4 ensure that the players are not disturbed,
12.2.5 supervise the progress of the competition,
12.2.6 take special measures in the interests of disabled players and those who need medical attention,
-
-
- follow the Anti-Cheating Rules or Guidelines
-
|
The Arbiter must take care to avoid any kind of cheating by the players. |
-
- The arbiter shall observe the games, especially when the players are short of time, enforce decisions he has made, and impose penalties on players where appropriate.
- The arbiter may appoint assistants to observe games, for example when several players are short of time.
- The arbiter may award either or both players additional time in the event of external disturbance of the game.
- The arbiter must not intervene in a game except in cases described by the Laws of Chess. He shall not indicate the number of moves completed, except in applying Article
8.5 when at least one flag has fallen. The arbiter shall refrain from informing a player that his opponent has completed a move or that the player has not pressed his clock.
-
- If someone observes an irregularity, he may inform only the arbiter. Players in other games must not to speak about or otherwise interfere in a game. Spectators are not allowed to interfere in a game. The arbiter may expel offenders from the playing venue.
|
This Article includes also the calling of a flag fall. |
-
- Unless authorised by the arbiter, it is forbidden for anybody to use a mobile phone or any kind of communication device in the playing venue or any contiguous area designated by the arbiter.
|
Nobody is allowed to use their mobiles in the playing hall during the games. |
-
- Options available to the arbiter concerning penalties:
- warning,
- increasing the remaining time of the opponent,
- reducing the remaining time of the offending player,
- increasing the points scored in the game by the opponent to the maximum available for that game,
- reducing the points scored in the game by the offending person,
- declaring the game to be lost by the offending player (the arbiter shall also decide the opponent’s score),
- a fine announced in advance,
- exclusion from one or more rounds,
- expulsion from the competition.
- Options available to the arbiter concerning penalties:
|
Article 12.9.9. is also be applied in cooperation with the organiser of the event. |
APPENDICES
Appendix A. Rapid chess
-
- A ‘Rapid chess’ game is one where either all the moves must be completed in a fixed time of more than 10 minutes but less than 60 minutes for each player; or the time allotted plus 60 times any increment is of more than 10 minutes but less than 60 minutes for each player.
|
Example 1: According to the Tournament Regulations of an event, the time control is 30 minutes for the whole game and 30 seconds increment for each move. That is: for 60 moves we would get 30'+ (30"x 60) = 30' +30' = 60'. So as according to the Article A1 "A Rapidplay" is a game where all moves must be completed in less than 60 minutes for each player, then such a game is considered to be standard chess. Example 2: According to the Tournament Regulations of an event, the time control is 10 minutes for the whole game and 5 seconds increment for each move. That is: for 60 moves we would get 10'+ (5” x 60) = 10' +5' = 15'. So as according to the Article A.1 such a game is considered to be Rapidplay chess |
-
- Players do not need to record the moves, but do not lose their rights to claims normally based on a scoresheet. The player can, at any time, ask the arbiter to provide him with a scoresheet, in order to write the moves.
|
Players are allowed to record the moves, but they may stop recording any time they wish. |
-
-
- The Competition Rules shall apply if:
- one arbiter supervises at most three games and
- each game is recorded by the arbiter or his assistant and, if possible, by electronic means.
- The player may at any time, when it is his move, ask the arbiter or his assistant to show him the scoresheet. This may be requested a maximum of five times in a game. More requests shall be considered as a distraction of the opponent.
- The Competition Rules shall apply if:
-
|
In case that a player asks the Arbiter to show him the score sheet, the clock should not be stopped. |
-
- Otherwise the following apply:
- From the initial position, once 10 moves have been completed by each player,
- no change can be made to the clock setting, unless the schedule of the event would be adversely affected
- no claim can be made regarding incorrect set-up or orientation of the chessboard.
- From the initial position, once 10 moves have been completed by each player,
- Otherwise the following apply:
In case of incorrect king placement, castling is not allowed. In case of incorrect rook placement, castling with this rook is not allowed.
-
-
- If the arbiter observes an action taken under Article 7.5.1, 7.5.2, 7.5.3 or 7.5.4, he shall act according to Article 7.5.5, provided the opponent has not made his next move. If the arbiter does not intervene, the opponent is entitled to claim, provided the opponent has not made his next move. If the opponent does not claim and the arbiter does not intervene, the illegal move shall stand and the game shall continue. Once the opponent has made his next move, an illegal move cannot be corrected unless this is agreed by the players without intervention of the arbiter.
-
|
It means that the player does not lose the game with the first illegal move, but only with the second, as it is in standard chess. The penalty is also the same as in standard chess. |
-
-
- To claim a win on time, the claimant may stop the chessclock and notify the arbiter. However, the game is drawn if the position is such that the claimant cannot checkmate the player’s king by any possible series of legal moves.
-
|
In case that both clocks show the indication 0.00, no claim for win on time can be submitted by the players, but the Arbiter shall decide the result of the game according the indication of “-“ that is shown on one of the clocks. The player whose clock shows this indication loses the game. |
-
-
- If the arbiter observes both kings are in check, or a pawn on the rank furthest from its starting position, he shall wait until the next move is completed. Then, if an illegal position is still on the board, he shall declare the game drawn.
-
|
If the player completes a move by giving a check and the opponent completes his next move by also giving a check (creating a position where both Kings are in check), and the player, instead of claiming the opponent’s illegal move, completes his next move with his King, avoiding the check, then the game shall be continued by the next opponent’s move, as the new position is not illegal any more. No illegal move can be claimed by the opponent. |
-
-
- The arbiter shall also call a flag fall, if he observes it.
-
|
It is an obligation of the Arbiter to call the flag fall, if he sees it. |
-
- The regulations of an event shall specify whether Article A.3 or Article A.4 shall apply for the entire event.
Appendix B. Blitz
-
- A ‘blitz’ game is one where all the moves must be completed in a fixed time of 10 minutes or less for each player; or the allotted time plus 60 times any increment is 10 minutes or less.
|
According to the Tournament Regulations of an event the time control is 5 minutes for the whole game and 5 seconds increment for each move. That is: for 60 moves we would get 5'+ (5'x60) = 5'+5' = 10'. According to Art. B.1 we have a Blitz game. |
-
- The penalties mentioned in Articles 7 and 9 of the Competition Rules shall be one minute instead of two minutes.
- The Competition Rules shall apply if:
- one arbiter supervises one game and
- each game is recorded by the arbiter or his assistant and, if possible, by electronic means.
- The player may at any time, when it is his move, ask the arbiter or his assistant to show him the scoresheet. This may be requested a maximum of five times in a game. More requests shall be considered as a distraction of the opponent.
- The Competition Rules shall apply if:
- The penalties mentioned in Articles 7 and 9 of the Competition Rules shall be one minute instead of two minutes.
-
- Otherwise, play shall be governed by the Rapid chess Laws as in Article A.2 and A.4.
- The regulations of an event shall specify whether Article B.3 or Article B.4 shall apply for the entire event.
|
In Blitz games the Arbiter SHALL CALL the flag fall, if he observes it, as in Rapid play |
|
In Blitz games, if the player asks from the Arbiter to see the score sheet, the clock should not be stopped, as in Rapidplay. |
Appendix C. Algebraic notation
FIDE recognises for its own tournaments and matches only one system of notation, the Algebraic System, and recommends the use of this uniform chess notation also for chess literature and periodicals. Score sheets using a notation system other than algebraic may not be used as evidence in cases where normally the score sheet of a player is used for that purpose. An arbiter who observes that a player is using a notation system other than the algebraic should warn the player of this requirement.
Description of the Algebraic System
-
- In this description, ‘piece’ means a piece other than a pawn.
- Each piece is indicated by an abbreviation. In the English language it is the first letter, a capital letter, of its name. Example: K=king, Q=queen, R=rook, B=bishop, N=knight. (N is used for a knight, in order to avoid ambiguity.)
- For the abbreviation of the name of the pieces, each player is free to use the name which is commonly used in his country. Examples: F = fou (French for bishop), L = loper (Dutch for bishop). In printed periodicals, the use of figurines is recommended.
- Pawns are not indicated by their first letter, but are recognised by the absence of such a letter. Examples: the moves are written e5, d4, a5, not pe5, Pd4, pa5.
- The eight files (from left to right for White and from right to left for Black) are indicated by the small letters, a, b, c, d, e, f, g and h, respectively.
- The eight ranks (from bottom to top for White and from top to bottom for Black) are numbered 1, 2, 3, 4, 5, 6, 7, 8, respectively. Consequently, in the initial position the white pieces and pawns are placed on the first and second ranks; the black pieces and pawns on the eighth and seventh ranks.
- As a consequence of the previous rules, each of the sixty-four squares is invariably indicated by a unique combination of a letter and a number.

-
- Each move of a piece is indicated by the abbreviation of the name of the piece in question and the square of arrival. There is no need for a hyphen between name and square. Examples: Be5, Nf3, Rd1. In the case of pawns, only the square of arrival is indicated. Examples: e5, d4, a5.
A longer form containing the square of departure is acceptable. Examples: Bb2e5, Ng1f3, Ra1d1, e7e5, d2d4, a6a5.
-
- When a piece makes a capture, an x may be inserted between:
- the abbreviation of the name of the piece in question and
- the square of arrival. Examples: Bxe5, Nxf3, Rxd1, see also C.10.
- When a pawn makes a capture, the file of departure must be indicated, then an x may be inserted, then the square of arrival. Examples: dxe5, gxf3, axb5. In the case of an ‘en passant’ capture, ‘e.p.’ may be appended to the notation. Example: exd6 e.p.
- If two identical pieces can move to the same square, the piece that is moved is indicated as follows:
- If both pieces are on the same rank by:
- the abbreviation of the name of the piece,
- the file of departure, and C.10.1.2 the square of arrival.
- If both pieces are on the same rank by:
- When a piece makes a capture, an x may be inserted between:
-
-
- If both pieces are on the same file by:
- the abbreviation of the name of the piece,
- the rank of the square of departure, and
- the square of arrival.
- If the pieces are on different ranks and files, method 1 is preferred. Examples:
- There are two knights, on the squares g1 and e1, and one of them moves to the square f3: either Ngf3 or Nef3, as the case may be.
- There are two knights, on the squares g5 and g1, and one of them moves to the square f3: either N5f3 or N1f3, as the case may be.
- There are two knights, on the squares h2 and d4, and one of them moves to the square f3: either Nhf3 or Ndf3, as the case may be.
- If a capture takes place on the square f3, the notation of the previous examples is still applicable, but an x may be inserted: 1) either Ngxf3 or Nexf3, 2) either N5xf3 or N1xf3, 3) either Nhxf3 or Ndxf3, as the case may be.
- If both pieces are on the same file by:
- In the case of the promotion of a pawn, the actual pawn move is indicated, followed immediately by the abbreviation of the new piece. Examples: d8Q, exf8N, b1B, g1R.
- The offer of a draw shall be marked as (=).
- Abbreviations
-
0-0 = castling with rook h1 or rook h8 (kingside castling)
0-0-0 = castling with rook a1 or rook a8 (queenside castling) x = captures
+ = check
++ or # = checkmate
e.p. = captures ‘en passant’ The last four are optional.
Sample game: 1.e4 e5 2. Nf3 Nf6 3. d4 exd4 4. e5 Ne4 5. Qxd4 d5 6. exd6 e.p. Nxd6 7. Bg5 Nc6 8. Qe3+ Be7 9. Nbd2 0-0 10. 0-0-0 Re8 11. Kb1 (=)
Or: 1. e4 e5 2. Nf3 Nf6 3. d4 ed4 4. e5 Ne4 5. Qd4 d5 6. ed6 Nd6 7. Bg5 Nc6 8. Qe3
Be7 9 Nbd2 0-0 10. 0-0-0 Re8 11. Kb1 (=)
Or: 1. e2e4 e7e5 2.Ng1f3 Ng8f6 3. d2d4 e5xd4 4. e4e5 Nf6e4 5. Qd1xd4 d7d5 6.
e5xd6 e.p. Ne4xd6 7. Bc1g5 Nb8c6 8. Qd4d3 Bf8e7 9. Nb1d2 0-0 10. 0-0-0 Rf8e8
11. Kb1 (=)
Appendix D. Rules for play with blind and visually disabled players
-
- The organiser, after consulting the arbiter, shall have the power to adapt the following rules according to local circumstances. In competitive chess between sighted and visually disabled (legally blind) players either player may demand the use of two boards, the sighted player using a normal board, the visually disabled player using one specially constructed. This board must meet the following requirements:
- measure at least 20 cm by 20 cm,
- have the black squares slightly raised,
- have a securing aperture in each square,
- The requirements for the pieces are:
- all are provided with a peg that fits into the securing aperture of the board,
- all are of Staunton design, the black pieces being specially marked.
- The following regulations shall govern play:
- The moves shall be announced clearly, repeated by the opponent and executed on his chessboard. When promoting a pawn, the player must announce which piece is chosen. To make the announcement as clear as possible, the use of the following names is suggested instead of the corresponding letters:
- The organiser, after consulting the arbiter, shall have the power to adapt the following rules according to local circumstances. In competitive chess between sighted and visually disabled (legally blind) players either player may demand the use of two boards, the sighted player using a normal board, the visually disabled player using one specially constructed. This board must meet the following requirements:
A - Anna B - Bella C - Cesar D - David E - Eva
F - Felix G - Gustav H - Hector
Unless the arbiter decides otherwise, ranks from White to Black shall be given the German numbers
- - eins
- - zwei
- - drei
- - vier
- - fuenf
- - sechs
- - sieben
- - acht
Castling is announced “Lange Rochade” (German for long castling) and “Kurze Rochade” (German for short castling).
The pieces bear the names: Koenig, Dame, Turm, Laeufer, Springer, Bauer.
-
-
- On the visually disabled player's board a piece shall be considered ‘touched’ when it has been taken out of the securing aperture.
- A move shall be considered ‘made’ when:
- in the case of a capture, the captured piece has been removed from the board of the player whose turn it is to move,
- a piece has been placed into a different securing aperture,
- the move has been announced.
- Only then shall the opponent's clock be started.
- As far as points D.2.2 and D.2.3 are concerned, the normal rules are valid for the sighted player.
-
D.2.6.1 A specially constructed chessclock for the visually disabled shall be admissible. It should be able to announce the time and number of moves to the visually disabled player.
D.6.2.2 Alternatively an analogue clock with the following features may be considered:
-
-
-
-
- a dial fitted with reinforced hands, with every five minutes marked by one raised dot, and every 15 minutes by two raised dots, and
- a flag which can be easily felt; care should be taken that the flag is so arranged as to allow the player to feel the minute hand during the last five minutes of the full hour.
-
- The visually disabled player must keep score of the game in Braille or longhand, or record the moves on a recording device.
- A slip of the tongue in the announcement of a move must be corrected immediately and before the clock of the opponent is started.
- If during a game different positions should arise on the two boards, they must be corrected with the assistance of the arbiter and by consulting both players' game scores. If the two game scores correspond with each other, the player who has written the correct move but made the wrong one must adjust his position to correspond with the move on the game scores. When the game scores are found to differ, the moves shall be retraced to the point where the two scores agree, and the arbiter shall readjust the clocks accordingly.
- The visually disabled player shall have the right to make use of an assistant who shall have any or all of the following duties:
-
-
-
-
-
- making either player's move on the board of the opponent,
- announcing the moves of both players,
- keeping the game score of the visually disabled player and starting his opponent's clock
- informing the visually disabled player, only at his request, of the number of moves completed and the time used up by both players,
- claiming the game in cases where the time limit has been exceeded and informing the arbiter when the sighted player has touched one of his pieces,
- carrying out the necessary formalities in cases where the game is adjourned.
- If the visually disabled player does not make use of an assistant, the sighted player may make use of one who shall carry out the duties mentioned in points D.2.10.1 and D.2.10.2. An assistant must be used in the case of a visually disabled player paired with a hearing impaired player.
-
-
GUIDELINES
Guidelines I. Adjourned games
-
-
- If a game is not finished at the end of the time prescribed for play, the arbiter shall require the player having the move to ‘seal’ that move. The player must write his move in unambiguous notation on his scoresheet, put his scoresheet and that of his opponent in an envelope, seal the envelope and only then stop the chessclock. Until he has stopped the chessclock the player retains the right to change his sealed move. If, after being told by the arbiter to seal his move, the player makes a move on the chessboard he must write that same move on his scoresheet as his sealed move.
- A player having the move who adjourns the game before the end of the playing session shall be considered to have sealed at the nominal time for the end of the session, and his remaining time shall so be recorded.
- The following shall be indicated upon the envelope:
- the names of the players,
- the position immediately before the sealed move,
- the time used by each player,
- the name of the player who has sealed the move,
- the number of the sealed move,
- the offer of a draw, if the proposal is current,
- the date, time and venue of resumption of play.
-
-
- The arbiter shall check the accuracy of the information on the envelope and is responsible for its safekeeping.
- If a player proposes a draw after his opponent has sealed his move, the offer is valid until the opponent has accepted it or rejected it as in Article 9.1.
- Before the game is to be resumed, the position immediately before the sealed move shall be set up on the chessboard, and the times used by each player when the game was adjourned shall be indicated on the clocks.
- If prior to the resumption the game is agreed drawn, or if one of the players notifies the arbiter that he resigns, the game is concluded.
- The envelope shall be opened only when the player who must reply to the sealed move is present.
- Except in the cases mentioned in Articles 5, 6.9, 9.6 and 9.7, the game is lost by a player whose recording of his sealed move:
- is ambiguous, or
-
-
- is recorded in such a way that its true significance is impossible to establish, or
- is illegal.
- If, at the agreed resumption time:
- the player having to reply to the sealed move is present, the envelope is opened, the sealed move is made on the chessboard and his clock is started,
- the player having to reply to the sealed move is not present, his clock shall be started; on his arrival, he may stop his clock and summon the arbiter; the envelope is then opened and the sealed move is made on the chessboard; his clock is then restarted,
- the player who sealed the move is not present, his opponent has the right to record his reply on the scoresheet, seal his scoresheet in a fresh envelope, stop his clock and start the absent player’s clock instead of making his reply in the normal manner; if so, the envelope shall be handed to the arbiter for safekeeping and opened on the absent player’s arrival.
- Any player who arrives at the chessboard after the default time shall lose the game unless the arbiter decides otherwise. However, if the sealed move resulted in the conclusion of the game, that conclusion shall still apply.
- If the regulations of an event specify that the default time is not zero, the following shall apply: If neither player is present initially, the player who has to reply to the sealed move shall lose all the time that elapses until he arrives, unless the regulations of an event specify or the arbiter decides otherwise.
- If the envelope containing the sealed move is missing, the game shall continue from the adjourned position, with the clock times recorded at the time of adjournment. If the time used by each player cannot be re-established, the arbiter shall set the clocks. The player who sealed the move shall make the move he states he sealed on the chessboard.
- If it is impossible to re-establish the position, the game shall be annulled and a new game shall be played.
-
-
- If, upon resumption of the game, either player points out before making his first move that the time used has been incorrectly indicated on either clock, the error must be corrected. If the error is not then established the game shall continue without correction unless the arbiter decides otherwise.
- The duration of each resumption session shall be controlled by the arbiter’s timepiece. The starting time shall be announced in advance.
Guidelines II. Chess960 Rules
-
- Before a Chess960 game a starting position is randomly set up, subject to certain rules. After this, the game is played in the same way as regular chess. In particular, pieces and pawns have their normal moves, and each player's objective is to checkmate the opponent's king.
- Starting-position requirements
The starting position for Chess960 must meet certain rules. White pawns are placed on the second rank as in regular chess. All remaining white pieces are placed randomly on the first rank, but with the following restrictions:
-
-
- the king is placed somewhere between the two rooks, and
- the bishops are placed on opposite-coloured squares, and
- the black pieces are placed opposite the white pieces.
-
The starting position can be generated before the game either by a computer program or using dice, coin, cards, etc.
-
- Chess960 castling rules
- Chess960 allows each player to castle once per game, a move by potentially both the king and rook in a single move. However, a few interpretations of regular chess rules are needed for castling, because the regular rules presume initial locations of the rook and king that are often not applicable in Chess960.
- How to castle. In Chess960, depending on the pre-castling position of the castling king and rook, the castling manoeuvre is performed by one of these four methods:
- double-move castling: by making a move with the king and a move with the rook, or
- transposition castling: by transposing the position of the king and the rook, or
- king-move-only castling: by making only a move with the king, or
- rook-move-only castling: by making only a move with the rook.
- Recommendations:
- When castling on a physical board with a human player, it is recommended that the king be moved outside the playing surface next to his final position, the rook then be moved from its starting position to its final position, and then the king be placed on his final square.
- After castling, the rook and king's final positions should be exactly the same positions as they would be in regular chess.
- Clarification:
- Chess960 castling rules
Thus, after c-side castling (notated as 0-0-0 and known as queen-side castling in orthodox chess), the king is on the c-square (c1 for white and c8 for black) and the rook is on the d-square (d1 for white and d8 for black). After g-side castling (notated as 0-0 and known as king-side castling in orthodox chess), the king is on the g-square (g1 for white and g8 for black) and the rook is on the f-square (f1 for white and f8 for black).
-
-
-
- Notes
- To avoid any misunderstanding, it may be useful to state "I am about to castle" before castling.
- In some starting positions, the king or rook (but not both) does not move during castling.
- In some starting positions, castling can take place as early as the first move.
- All the squares between the king's initial and final squares (including the final square) and all the squares between the rook's initial and final squares (including the final square) must be vacant except for the king and castling rook.
- In some starting positions, some squares can stay filled during castling that would have to be vacant in regular chess. For example, after c-side castling 0-0-0, it is possible to have a, b, and/or e still filled, and after g-side castling (0-0), it is possible to have e and/or h filled.
- Notes
-
-
Guidelines III. Games without increment including Quickplay Finishes
III.1 A ‘quickplay finish’ is the phase of a game when all the remaining moves must be completed in a finite time.
|
Example 1: According to the Tournament Regulations of an event, the time control is 2 hours for 40 moves and then 1 hour for the end of the game. The last 1 hour will be played according to the rules of the Quickplay finish. Example 2: According to the Tournament Regulations of an event, the time control is 2 hours for the whole game. It means that the whole game will be played according to the rules of the Quick play finish. |
-
-
- The Guidelines below concerning the final period of the game including Quickplay Finishes, shall only be used at an event if their use has been announced beforehand.
- These Guidelines shall apply only to standard chess and rapid chess games without increment and not to blitz games.
-
-
-
- If both flags have fallen and it is impossible to establish which flag fell first then:
- the game shall continue if this occurs in any period of the game except the last period.
- the game is drawn if this occurs in the period of a game in which all remaining moves must be completed.
- If both flags have fallen and it is impossible to establish which flag fell first then:
- If the player having the move has less than two minutes left on his clock, he may request that an increment extra five seconds be introduced for both players. This constitutes the offer of a draw. If the offer refused, and the arbiter agrees to the request, the clocks shall then be set with the extra time; the opponent shall be awarded two extra minutes and the game shall continue.
- If Article III.4 does not apply and the player having the move has less than two minutes left on his clock, he may claim a draw before his flag falls. He shall summon the arbiter and may stop the chessclock (see Article 6.12.2). He may claim on the basis that his opponent cannot win by normal means, and/or that his opponent has been making no effort to win by normal means:
- If the arbiter agrees that the opponent cannot win by normal means, or that the opponent has been making no effort to win the game by normal means, he shall declare the game drawn. Otherwise he shall postpone his decision or reject the claim.
- If the arbiter postpones his decision, the opponent may be awarded two extra minutes and the game shall continue, if possible, in the presence of an arbiter. The arbiter shall declare the final result later in the game or as soon as possible after the flag of either player has fallen. He shall declare the game drawn if he agrees that the opponent of the player whose flag has fallen cannot win by normal means, or that he was not making sufficient attempts to win by normal means.
-
|
It means that the Arbiter may make a decision to declare a game as a draw, even after a flag fall has occurred. As it may sometimes cause many conflicts between Arbiters and players, the games played according to Quickplay finish should be avoided. This requires use of digital clocks. Time controls WITH an increment is a much better way of concluding a game. |
-
-
- If the arbiter has rejected the claim, the opponent shall be awarded two extra minutes.
- The following shall apply when the competition is not supervised by an arbiter:
- A player may claim a draw when he has less than two minutes left on his clock and before his flag falls. This concludes the game. He may claim on the basis:
- that his opponent cannot win by normal means, and/or
- that his opponent has been making no effort to win by normal means.
- A player may claim a draw when he has less than two minutes left on his clock and before his flag falls. This concludes the game. He may claim on the basis:
-
In III.6.1.1 the player must write down the final position and his opponent must verify it.
In III.6.1.2 the player must write down the final position and submit an up-to-date scoresheet. The opponent shall verify both the scoresheet and the final position.
-
-
- The claim shall be referred to the designated arbiter.
-
GLOSSARY OF TERMS IN THE LAWS OF CHESS
The number after the term refers to the first time it appears in the Laws.
adjourn: 8.1. Instead of playing the game in one session it is temporarily halted and then continued at a later time.
algebraic notation: 8.1. Recording the moves using a-h and 1-8 on the 8x8 board.
analyse: 11.3. Where one or more players make moves on a board to try to determine what is the best continuation.
appeal: 11.10. Normally a player has the right to appeal against a decision of the arbiter or organiser.
arbiter: Preface. The person(s) responsible for ensuring that the rules of a competition are followed.
arbiter’s discretion: There are approximately 39 instances in the Laws where the arbiter must use his judgement.
assistant: 8.1. A person who may help the smooth running of the competition in various ways.
attack: 3.1.A piece is said to attack an opponent’s piece if the player’s piece can make a capture on that square.
black: 2.1. 1. There are 16 dark-coloured pieces and 32 squares called black. Or 2. When capitalised, this also refers to the player of the black pieces.
blitz: B. A game where each player’s thinking time is 10 minutes or less.
board: 2.4.Short for chessboard.
Bronstein mode: 6.3.2 See delay mode.
capture: 3.1. Where a piece is moved from its square to a square occupied by an opponent’s piece, the latter is removed from the board. See also 3.7.4.1 i 3.4.7.2.In notation x.
castling: 3.8.2 A move of the king towards a rook. See the article. In notation 0-0 kingside castling, 0-0-0 queenside castling.
cellphone: See mobile phone.
check: 3.9. Where a king is attacked by one or more of the opponent’s pieces. In notation +.
checkmate: 1.2. Where the king is attacked and cannot parry the threat. In notation ++ or #.
chessboard: 1.1. The 8x8 grid as in 2.1.
chessclock: 6.1. A clock with two time displays connected to each other.
chess set: The 32 pieces on the chessboard.
Chess960: A variant of chess where the back-row pieces are set up in one of the 960 distinguishable possible positions
claim: 6.8. The player may make a claim to the arbiter under various circumstances.
clock: 6.1. One of the two time displays.
completed move: 6.2.1 Where a player has made his move and then pressed his clock.
contiguous area: 12.8. An area touching but not actually part of the playing venue. For example, the area set aside for spectators.
cumulative (Fischer) mode: Where a player receives an extra amount of time (often 30 seconds) prior to each move.
dead position: 5.2.2 Where neither player can mate the opponent’s king with any series of legal moves.
default time: 6.7. The specified time a player may be late without being forfeited.
delay (Bronstein) mode: 6.3.2Both players receive an allotted ‘main thinking time’. Each player also receives a ‘fixed extra time’ with every move. The countdown of the main thinking time only commences after the fixed extra time has expired. Provided the player presses his clock before the expiration of the fixed extra time, the main thinking time does not change, irrespective of the proportion of the fixed extra time used.
demonstration board: 6.13. A display of the position on the board where the pieces are moved by hand.
diagonal: 2.4.A straightline of squares of the same colour, running from one edge of the board to an adjacent edge.
disability: 6.2.6 A condition, such as a physical or mental handicap, that results in partial or complete loss of a person's ability to perform certain chess activities.
displaced: 7.4.1 to put or take pieces from their usual place. For example, a pawn from a2 to a4.5; a rook partway between d1 and e1; a piece lying on its side; a piece knocked onto the floor.
draw: 5.2. Where the game is concluded with neither side winning.
draw offer: 9.1.2 Where a player may offer a draw to the opponent. This is indicated on the scoresheet with the symbol (=).
e-cigarette: device containing a liquid that is vaporised and inhaled orally to simulate the act of smoking tobacco.
en passant: 3.7.4.1See that article for an explanation. In notation e.p.
exchange: 1. 3.7.5.3 Where a pawn is promoted. Or 2.Where a player captures a piece of the same value as his own and this piece is recaptured. Or 3. Where one player has lost a rook and the other has lost a bishop or knight.
explanation: 11.9. A player is entitled to have a Law explained.
fair play: 12.2.1 Whether justice has been done has sometimes to be considered when an arbiter finds that the Laws are inadequate.
file: 2.4. A vertical column of eight squares on the chessboard.
Fischer mode: See cumulative mode.
flag: 6.1. The device that displays when a time period has expired.
flag-fall: 6.1. Where the allotted time of a player has expired.
forfeit: 4.8.1. To lose the right to make a claim or move. Or 2. To lose a game because of an infringement of the Laws.
handicap: See disability.
I adjust: See j’adoube.
illegal: 3.10.1.A position or move that is impossible because of the Laws of Chess.
impairment: See disability.
increment: 6.1. An amount of time (from 2 to 60 seconds) added from the start before each move for the player. This can be in either delay or cumulative mode.
intervene: 12.7. To involve oneself in something that is happening in order to affect the outcome.
j’adoube: 4.2. Giving notice that the player wishes to adjust a piece, but does not necessarily intend to move it.
kingside: 3.8.1.The vertical half of the board on which the king stands at the start of the game.
legal move: See Article 3.10a.
made: 1.1. A move is said to have been ‘made’ when the piece has been moved to its new square, the hand has quit the piece, and the captured piece, if any, has been removed from the board.
mate: Abbreviation of checkmate. minor piece. Bishop or knight. mobile phone: 11.3.2. Cellphone.
monitor: 6.13. An electronic display of the position on the board.
move: 1.1. 1. 40 moves in 90 minutes, refers to 40 moves by each player. Or 2. having the move refers to the player’s right to play next. Or 3. White’s best move refers to the single move by White.
move-counter: 6.10.2. A device on a chessclock which may be used to record the number of times the clock has been pressed by each player.
normal means: G.5. Playing in a positive manner to try to win; or, having a position such that there is a realistic chance of winning the game other than just flag-fall.
organiser. 8.3. The person responsible for the venue, dates, prize money, invitations, format of the competition and so on.
over-the-board: Introduction. The Laws cover only this type of chess, not internet, nor correspondence, and so on.
penalties: 12.3. The arbiter may apply penalties as listed in 12.9 in ascending order of severity.
piece: 2. 1. One of the 32 figurines on the board. Or 2. A queen, rook, bishop or knight. playing area: 11.2. The place where the games of a competition are played.
playing venue: 11.2. The only place to which the players have access during play.
points: 10. Normally a player scores 1 point for a win, ½ point for a draw, 0 for a loss. An alternative is 3 for a win, 1 for a draw, 0 for a loss.
press the clock: 6.2.1 The act of pushing the button or lever on a chess clock which stops the player’s clock and starts that of his opponent.
promotion: 3.7.5.3 Where a pawn reaches the eighth rank and is replaced by a new queen, rook, bishop or knight of the same colour.
queen: As inqueen a pawn, meaning to promote a pawn to a queen.
queenside: 3.8.1. The vertical half of the board on which the queen stands at the start of the game.
quickplay finish: G. The last part of a game where a player must complete an unlimited number of moves in a finite time.
rank: 2.4. A horizontal row of eight squares on the chessboard.
rapid chess: A. A game where each player’s thinking time is more than 10 minutes, but less than 60.
repetition: 5.3.1. 1. A player may claim a draw if the same position occurs three times.
2. A game is drawn if the same position occurs five times.
resigns: 5.1.2 Where a player gives up, rather than play on until mated.
rest rooms: 11.2. Toilets, also the room set aside in World Championships where the players can relax.
result: 8.7. Usually the result is 1-0, 0-1 or ½-½. In exceptional circumstances both players may lose (Article 11.8), or one score ½ and the other 0. For unplayed games the scores are indicated by +/- (White wins by forfeit), -/+ (Black wins by forfeit), -/- (Both players lose by forfeit).
regulations of an event: 6.7.1 At various points in the Laws there are options. The regulations of an event must state which have been chosen.
sealed move: E. Where a game is adjourned the player seals his next move in an envelope.
scoresheet: 8.1. A paper sheet with spaces for writing the moves. This can also be electronic.
screen: 6.13. An electronic display of the position on the board.
spectators: 11.4. People other than arbiters or players viewing the games. This includes players after their games have been concluded.
standard chess: G3. A game where each player’s thinking time is at least 60 minutes.
stalemate: 5.2.1Where the player has no legal move and his king is not in check.
square of promotion: 3.7.5.1 The squarea pawn lands on when it reached the eighth rank.
supervise: 12.2.5Inspect or control.
time control: 1. The regulation about the time the player is allotted. For example, 40 moves in 90 minutes, all the moves in 30 minutes, plus 30 seconds cumulatively from move 1. Or2. A player is said ‘to have reached the time control’, if, for example he has completed the 40 moves in less than 90 minutes.
time period: 8.6.A part of the game where the players must complete a number of moves or all the moves in a certain time.
touch move: 4.3. If a player touches a piece with the intention of moving it, he is obliged to move it.
vertical: 2.4. The 8th rank is often thought as the highest area on a chessboard. Thus each file is referred to as ‘vertical’.
white: 2.2. 1. There are 16 light-coloured pieces and 32 squares called white. Or 2. When capitalised, this also refers to the player of the white pieces.
zero tolerance: 6.7.1. Where a player must arrive at the chessboard before the start of the session.
50-move rule: 5.3.2 A player may claim a draw if the last 50 moves have been completed by each player without the movement of any pawn and without any capture.
75-move rule: 9.6.2The game is drawn if the last 75 moves have been completed by each player without the movement of any pawn and without any capture.
GUIDELINES FOR THE ORGANISERS
Introduction
Evolution of the FIDE Laws of Chess has given more freedom to the organisers about the regulations of a specific event. The Competition Rules enable organisers to choose options which are the best, in their opinion, for a given tournament. But greater freedom means greater responsibility.
The FIDE Laws of Chess regulate many of the specific rules, but not always. For example, in Rapid chess and Blitz, the regulations of an event shall specify if the entire event shall be played according to the Competition Rules or with some exceptions. Apart of that, is good to remind the player of such important things as the default time and the conditions when a draw can be agreed.
If the organisers forget to make these matters clear in advance, it will not be any use making an announcement at the start of a round. Players may not be present and, anyway, do not listen.
To avoid such situations, the FIDE Rules Commission has decided to prepare Guidelines for the Organisers. These are divided in three parts: what must be specified in the regulations of the event; what the RC recommends be specified; and optional rules. The RC strongly recommends to the organisers that their choice should always be exercised in conjunction with the Chief Arbiter.
According to the article 6.7.1 of the FIDE Laws of Chess, the regulations of an event shall specify a default time. If the default time is not specified, then it is zero.
-
- Using the Competition Rules in Rapid chess or Blitz
According to the article A.5/B.5 of the FIDE Laws of Chess, the regulations of an event shall specify if the entire event shall be played according to the Competition Rules (all articles from 6 to the 12 of the FIDE Laws of Chess) or with some exceptions as described in the article A.4/B.4.
-
- Standard chess and Rapid chess without an increment – less than two minutes draw claim
The regulation of the event shell specify if the game is played according to Guidelines III (Quickplay Finishes), as described in the article III.2.1. If yes, than the regulations of an event shall specify the procedure for the player having the move and less than two minutes left on his clock for a draw claim. There are two options: according to the article III.4 of the FIDE Laws of Chess, an increment extra five seconds be introduced for both players or according to the article III.5 of the FIDE Laws of Chess, a draw claim procedure shall follow. If these matters are not specified, then, for example, king and knight v king and knight can be played on until one flag falls.
According to the article 9.1.1 of the FIDE Laws of Chess, the regulations of an event may specify that players cannot offer or agree to a draw, whether in less than a specified number of moves or at all, without the consent of the arbiter. If the draw condition is not specified then, according to the article 5.3.2 of the FIDE Laws of Chess, players can offer or agree to a draw when both have made at least one move.
-
- Electronic device
According to the article 11.3.2.1 of the FIDE Laws of Chess, the regulations of an event may allow to the player to have an electronic device not specifically approved by the arbiter in the playing venue, provided that this device is stored in a player’s bag and the device is completely switched off. This bag must be placed as agreed with the arbiter. Neither player is allowed are to use this bag without permission of the arbiter. If the above permission is not specified, then is forbidden to have any electronic device in the playing venue.
According to the article 10.1 of the FIDE Laws of Chess, the regulations of an event may specify a different scoring system. For example a player who wins his game, or wins by forfeit, scores three points (3), a player who draws his game scores a two points (2), a player who loses his game scores one point (1), a player who loses by default scores zero points (0). If not specified, normal scoring is used (1, ½, 0).
-
- Leaving the playing area
According to Article 11.2.4 of the FIDE Laws of Chess, the regulations of an event may specify that the opponent of the player having a move must report to the arbiter when he wishes to leave the playing area. If this is not specified, there is no obligation for the opponent to communicate his intention to leave.
-
- Appeal procedure
According to the article 11.10 of the FIDE Laws of Chess, the regulations of an event may specify that a player cannot appeal against any decision of the arbiter, if he has signed the scoresheet. If not specified, the player may appeal even after signing the scoresheet. It is strongly recommended that an Appeal Committee should be set up in advance.
-
- Adjourned games – elapsed time before arrival of the players
According to the article I.11 of the FIDE Laws of Chess, the regulations of an event may specify the procedure regarding elapsed time before arrival of the players. If not specified, than the player who has to reply to the sealed move shall lose all the time that elapses until he arrives, even if both players are not present initially.
FIDE COMPETITION (TOURNAMENT) RULES
Approved by the 1986 General Assembly, 2007 PB
Amended by the 1989, 1992, 1993, 1994, 1998, 2006, 2010, 2014 General Assemblies and 2011 Executive Board.
Preface
All chess competitions shall be played according to the FIDE Laws of Chess (E.I.01A). The FIDE Competition Rules shall be used in conjunction with the Laws of Chess and shall apply to all official FIDE competitions. These Rules shall also be applied to all FIDE-rated competitions, amended where appropriate. The organisers, competitors and arbiters involved in any competition are expected to be acquainted with these Rules before the start of the competition. In these Rules the words ‘he’, ‘him’ and ‘his’ shall be considered to include ‘she’ and ‘her’.
National Laws take precedence over FIDE Rules.
- Scope
-
- Where an event has a situation not covered by internal rules, these Rules shall be considered to be definitive.
- These Rules apply to the following levels of competition.
L1: Official FIDE events as defined by the FIDE Events Commission (D.IV.01.1) or FIDE World Championship and Olympiad Commission (D.I, D.II)
L2: Competitions where FIDE titles and title norms can be earned L3: FIDE Rated Competitions
L4: All other competitions
Rules that apply to specific types of competitions shall have the competition level indicated. Otherwise the rules shall apply to all levels of competitions.
-
- These competition rules may contain regulations defined by other FIDE Commissions, which are listed in the FIDE Handbook. Where possible, references to these external regulations shall be shown.
- The Chief Organiser (CO)
-
- The federation or administrative body responsible for the organisation of a competition may entrust the technical organisation to a CO. He, together with the federation or organising body, may appoint an Organising Committee to be responsible for all financial, technical and organisational matters.
Other rules hereunder may apply also to the role of the CO. He and the Chief Arbiter (see 3) must work closely together in order to ensure the smooth running of an event.
- The Chief Arbiter (CA)
-
- The duties of the CA are as specified by the Laws of Chess, other FIDE Rules and the other Rules of the Competition. During the event he also has to keep the record of each round; to oversee the proper course of the competition; to ensure order in the playing venue: players’ comfort during play; to supervise the work of the technical staff of the competition.
- Prior to the start of the competition:
- he may draw up additional rules in consultation with the CO;
- he shall check all the conditions for play, including the playing venue, playing area, lighting, heating, air conditioning, ventilation, noise, security and so on.;
- he must acquire through the CO all the necessary equipment, ensure a sufficient number of arbiters, auxiliary technical staff and assistants are engaged and ensure that conditions for the arbiters are satisfactory. Whether the playing conditions meet the requirements of these FIDE Rules is his final decision.
- At the conclusion of the event the CA shall report as appropriate.
Refer to the Technical Commission Rules
Refer to the Technical Commission Rules
- Play
-
- All games must be played in the playing area at the times specified in advance by the organisers, unless otherwise decided by the CA (in consultation with the CO).
- If possible, a separate area outside the playing area shall be provided where smoking is permitted. This shall be easily accessible from the playing area. If local ordinances totally prohibit smoking on the premises, the players and officials shall be given easy access to the outside.
- If mechanical chess clocks are used, they shall be set so that each unit registers six o’clock at the first time control.
- For FIDE events (L1) with 30 players or more, at any stage, a large digital countdown device shall be installed in the playing hall. For FIDE events with fewer than 30 players an appropriate announcement shall be made five minutes before the game is due to start and again one minute before the start of the game.
- After the finish of the game, the score sheets shall be signed. Then: the arbiter or the players shall place the kings in the middle of the board to indicate the result of the game. For a win by White, the kings shall be placed on e4 and d5 (the white centre squares); for a win by Black, the kings shall be placed on d4 and e5 (the black centre squares), for a draw, the kings shall be placed on d4 and d5 or on e4 and e5.
But, if electronic boards are used, an illegal move shall be made, before placing the kings in the centre.
-
- Where it is clear results have been arranged (E.I.01A.11.1), the CA shall impose suitable penalties (E.I.01A.12).
- A glossary of common relevant terms in several languages should be available to the arbiter.
- Pairings
-
- Responsibility for the drawing of lots and the actual pairings rests with the CA.
- The drawing of lots for the first round of a round-robin competition shall be arranged by the CO, to be open to the players.
- In L1, L2: round robin competitions and preferable Swisses, the drawing of lots shall take place at least 12 hours before the start of the first round. In L1 all participants shall attend the ceremony of drawing of lots. A player who has not arrived on time for the drawing of lots may be included at the discretion of the CA. The first-round pairings shall be announced as soon as possible thereafter.
- If a player withdraws or is excluded from a competition after the drawing of lots but before the beginning of the first round, or there are additional entries, the announced pairings shall remain unaltered. Additional pairings or changes may be made at the discretion of the CA in consultation with the players directly involved, but only if these minimise amendments to pairings that have already been announced.
-
- The pairings for a round robin should be made in accordance with the Berger tables (Annex 1), adjusted where necessary for double-round events.
- If the pairings are to be restricted in any way - for example, players from the same federation shall, if possible, not meet in the last three rounds - this shall be communicated to the players as soon as possible, but not later than the start of the first round.
- For round-robin competitions this restricted drawing of lots may be done by using the Varma tables, reproduced in Annex 2, which can be used for competitions of 9 to 24 players
- For the pairings of a Swiss-system competition the pre-announced pairing system and program shall apply. (C.04)
“Player” in 8.1 -8.3.3, includes a “team” where appropriate.
-
- If a player has lost a game by default for insufficient reason, he shall be expelled unless the CA decides otherwise.
- Round robins
- Each player has entered into a contract to play throughout the tournament.
- When a player withdraws or is expelled from a tournament, the effect shall be as follows:
- If a player has completed less than 50 % of his games, the results shall remain in the tournament table (for rating and historical purposes, but they shall not be counted in the final standings. The unplayed games of the player are indicated by (-) in the tournament table and those of his opponents by (+). If neither player is present this will be indicated by two (-).
- If a player has completed at least 50 % of his games, the results shall remain in the tournament table and shall be counted in the final standings. The unplayed games of the player are shown as above.
- Swisses
- If a player withdraws, the results shall remain in the cross-table for ranking purposes. Only games that are actually played shall be rated
- If a player cannot play a particular round it is essential to inform the Pairings Controller and CA before the pairings for that round are made.
-
-
- In an L2, L3 or L4 tournament: If, after the round has started two players do not have a game, then they can be paired against each other. This is only allowed when the arbiter and both players agree and they have not already played in this tournament. The arbiter shall adjust the clock times in an equitable manner.
- In an L2, L3 or L4 tournament the rules may permit a player to take a half point bye in a given round. It is only allowed if adequate notice has been given and is agreed to by the arbiter.
-
Such permission might not be granted to a player who receives conditions, or who has been given a free entry to the tournament. It is not permitted in the last round of a tournament.
|
Normally such ½ point byes may be given to players who cannot be present in the first and in the second round of the tournament. It is advisable not to give them in later rounds, especially in the last round, because they can affect the final standings and thus the prize distribution. |
- Conduct of the Players
-
- Once a player has formally accepted an invitation, he must play except in exceptional circumstances (force majeure), such as illness or incapacity. Acceptance of another invitation is not considered to be a valid reason for not participating or for withdrawing.
- All the participants should be dressed in a suitable manner.
- A player who does not wish to continue a game and leaves without resigning or notifying the arbiter is discourteous. He may be penalised, at the discretion of the CA, for poor sportsmanship (E.I.01A.12.9)
- A player shall not speak about any game while it is in progress, except as allowed in the Laws of Chess.
- In a team competition a player must not stand behind the opposing team during play.
- All complaints concerning the behaviour of players or captains shall be made to the arbiter.
A player is not permitted to complain directly to his opponent (E.I.01A.11.5)
of Chess or the Competition Rule. Then the CA (in consultation with the CO) shall have discretionary power to impose penalties. He should seek to maintain discipline and offer other solutions which may placate the offended parties.
-
- In all competitions there shall be an Appeals Committee (AC). The CO shall ensure that the AC is elected or appointed before the start of the first round, usually at the drawing of lots, or players’ meeting. It is recommended that the AC consist of a Chairman, at least two members and two reserve members. The Chairman, the members and reserve members shall, if possible, be from different federations, if it is an international competition. No member of the AC involved in the dispute shall rule in that dispute. Such a committee should have an odd number of voting members. Members of the AC shall not be younger than 21 years old.
- A player or a registered official representing a player or team may appeal against any ruling made by the CA or CO or one of their assistants. Such an official may include the player's team captain, head of delegation or other person as defined in the rules of the event.,
- An appeal shall be accompanied by a fee and submitted in written form not later than the deadline. Both fee and deadline shall be fixed in advance. The decisions of the AC shall be final. The fee is returnable if the appeal is successful. The fee (or part of it) may also be returned if the appeal is unsuccessful but considered reasonable in the view of the committee.
- TV, Filming, Photography
-
- Television cameras that are noiseless and unobtrusive are permitted in the playing venue and contiguous areas with the approval of the CO and CA. The CA shall ensure the players are not disturbed or distracted in any way by the presence of TV, video cameras or other equipment.
- Only authorised photographers may take photographs in the playing venue. Use of flash in the playing area is restricted to the first ten minutes of the first round and the first five minutes of each subsequent round, unless the CA decides otherwise.
The Competition Rules may include other rules due to the peculiarities of the event. The authorised photographers may take photographs without flash during the rest of the round in the playing area, only with the permission of the CA
A team competition is one where the results of individual games contribute equally to the final score of a defined group of players.
-
- Depending on the rules of the specific competition, the captain shall be required to deliver at a specific time a written list naming the players in his team participating in
each round, to communicate to his players the pairings, to sign the protocol indicating the results in the match at the end of play.
-
- A team captain is allowed to leave or re-enter the playing venue only with the permission of the arbiter.
- The team captain must not stand behind the opposing team during play.
- If the team captain wishes to speak to one of his players, he shall first approach the arbiter. The team captain shall then speak to the player in the presence of an arbiter, using a language the arbiter can understand. The same procedure shall be followed if a player needs to speak to the captain.
- A team captain is entitled to advise the players of his team to make or accept an offer of a draw unless the regulations of the event stipulate otherwise. He shall not intervene in a game in any other way. He must not discuss any position on any board during play.
- The team captain may delegate his functions to another person, provided he informs the CA of this in writing in advance.
|
In the regulations of a Team Tournament, details about the Team Compositions should be included. Normally the following may be applied: A fixed board order (it might be according to the ELO rating of the players; the highest rating gets no 1. Some events allow the captain full discretion, others do not permit a player to play on a board higher than a team mate who is 100 Rating points higher. The team list must be submitted before the first round at a time stipulated by the regulations. The order shall not be changed during the whole tournament. If a team has reserve(s): then for every round, each team must submit its composition (for example, if the team consists of 4 players and one reserve, the team composition may be: 1, 2, 3, 4, or 1, 2, 4, 5, or 1, 3, 4, 5, or 1, 2, 3, 5 or 2, 3, 4, 5,), provided a given deadline before the start of the round. Where the captain does not submit any composition by the deadline, its composition shall be: 1, 2, 3, 4. No player with higher number in the fixed board order is allowed to play above a player with lower number. The reserve player must always play board 4. Other permutations are not allowed. Where players play on the wrong boards, the result of the game counts for the rating, but not for the final score in the match. They will be forfeited (+/- or -/+). |
|
A team |
- |
B team |
|
|
1A |
1-0 |
2B: correct board |
|
|
2A |
0-1 |
4B: wrong board (it should be 3B) |
+ - : corrected result |
|
3A |
½ |
3B: wrong board (it should be 4B) |
+ - : corrected result |
|
5A |
0-1 |
5B: correct board |
|
-
Example:
Initial Match Result: 1.5-2.5 (valid only for ratings)
Corrected result of the
Match: 3.0-1.0 (valid for standings and future pairings).
Each Team Captain is responsible for the submission of the compositions of their team to the appropriate officer.
The Arbiters of a Team Tournament, in cooperation with the Team Captains, MUST CHECK the Teams Compositions for every round, in order to avoid incorrect board order.
- Invitation, Registration and Functions
-
- Invitations to an official FIDE competition shall be issued as soon as feasible.
- The CO shall send, through the respective national federations, invitations to all participants qualified for the competition. The invitation letter shall first be approved by the President of FIDE for World Championship competitions, and by the Continental President for Continental Championship competitions.
- The invitation shall be as comprehensive as possible, stating clearly the expected conditions and giving all details which may be of use to the player. The following should be included in the invitation letter and/or brochure which should also be posted on the FIDE website:
- The dates and site of the Competition
- The FIDE Regulations
- The hotel(s) where the players are to stay (including e-mail, fax and telephone numbers)
- The Competition schedule: dates, times of play and places of: arrival, the opening ceremony, drawing of lots, play, special events, the closing ceremony, departure.
- The rate of play and the type of clocks to be used in the Competition.
- The pairing system for the event and the tie-break system to be used.
- The default-time (which for official FIDE events shall be the start of the round).
- The specific rules for draw agreements if there is any restriction.
- For Rapidplay and Blitz competitions, whether Article A3 or A4, or B3 or B4 applies.
- For competitions played without an increment, whether Appendix G applies.
- The financial arrangements: travel expenses; accommodation; duration for which board and lodging shall be provided, or the cost of such accommodation, including that for people accompanying the player; arrangements for meals; start money; pocket money; entry fee; full details of the prize fund, including special prizes; point money; the currency in which money shall be disbursed; tax liability; visas and how to obtain them.
- Whether the event shall be rated or not.
- The means for reaching the playing venue and arrangements for transportation.
- The likely number of participants, the names of players invited and the name of the Chief Arbiter (CA).
- The website of the event, contact details of the organisers including the name of the CO.
- The players’ responsibility towards the media, general public, sponsors, government representatives and other similar considerations.
- Dress code, if any
- Any smoking restrictions shall be mentioned in the invitation.
- Security Arrangements.
- Special medical considerations such as vaccinations recommended or required in advance.
- Arrangements for: tourism, special events, internet access, and so on.
- The date by which a player must give a definite reply to the invitation and where and when he shall report his arrival.
- In his reply a player may mention pre-existing medical conditions and special dietary and/or religious requirements.
- If the organiser has to take special measures due to a disability of the player, the player shall notify the organiser in his reply.
- Once an invitation has been issued to a player, it must not be withdrawn, provided the player accepts the invitation by the reply date. If an event is cancelled or postponed the organisers shall provide compensation.
- The CO shall guarantee medical treatment and medicines for all participants, official seconds, arbiters and officials of an official FIDE competition and shall insure said people against accidents and the need for medical services, including medicine , surgical procedures, and so on, but shall have no responsibility where there is a chronic condition. An official doctor shall be appointed for the duration of the competition.
-
- The same protocol as in 13.3-5 shall be followed for L2, L3, L4 competitions, amended where appropriate.
-
- 14.1 The CA of an official World Event shall be nominated by the President of FIDE in consultation with the CO. The CA of a Continental Championship competition shall be nominated by the Continental President, in consultation with the CO. However, if the Continental Championship is a qualification event for the World Championship, the candidacy of the CA must be agreed with FIDE.
- The CA shall have the title of International Arbiter classified “A” or “B” (B.06) and shall have adequate experience of FIDE competitions, FIDE official languages and relevant FIDE regulations. FIDE and/or the Organising Committee shall nominate the arbiters and other staff.
Berger Tables
Berger Tables for Round-Robin Tournaments
Where there is an odd number of players, the highest number counts as a bye.
3 or 4 players:
Rd 1: 1-4, 2-3.
Rd 2: 4-3, 1-2.
Rd 3: 2-4, 3-1.
5 or 6 players:
Rd 1: 1-6, 2-5, 3-4.
Rd 2: 6-4, 5-3, 1-2.
Rd 3: 2-6, 3-1, 4-5.
Rd 4: 6-5, 1-4, 2-3.
Rd 5: 3-6, 4-2, 5-1.
7 or 8 players:
Rd 1: 1-8, 2-7, 3-6, 4-5.
Rd 2: 8-5, 6-4, 7-3, 1-2.
Rd 3: 2-8, 3-1, 4-7, 5-6.
Rd 4: 8-6, 7-5, 1-4, 2-3.
Rd 5: 3-8, 4-2, 5-1, 6-7.
Rd 6: 8-7, 1-6, 2-5, 3-4.
Rd 7: 4-8, 5-3, 6-2, 7-1.
9 or 10 players:
Rd 1: 1-10, 2-9, 3-8, 4-7, 5-6.
Rd 2: 10-6, 7-5, 8-4, 9-3, 1-2.
Rd 3: 2-10, 3-1, 4-9, 5-8, 6-7.
Rd 4: 10-7, 8-6, 9-5, 1-4, 2-3.
Rd 5: 3-10, 4-2, 5-1, 6-9, 7-8.
Rd 6: 10-8, 9-7, 1-6, 2-5, 3-4.
Rd 7: 4-10, 5-3, 6-2, 7-1, 8-9.
Rd 8: 10-9, 1-8, 2-7, 3-6, 4-5.
Rd 9: 5-10, 6-4, 7-3, 8-2, 9-1.
11 or 12 players:
|
Rd 1: |
1-12, 2-11, 3-10, 4-9, 5-8, 6-7. |
|
Rd 2: |
12-7, 8-6, 9-5, 10-4, 11-3, 1-2. |
|
Rd 3: |
2-12, 3-1, 4-11, 5-10, 6-9, 7-8. |
|
Rd 4: |
12-8, 9-7, 10-6, 11-5, 1-4, 2-3. |
|
Rd 5: |
3-12, 4-2, 5-1, 6-11, 7-10, 8-9. |
|
Rd 6: |
12-9, 10-8, 11-7, 1-6, 2-5, 3-4. |
|
Rd 7: |
4-12, 5-3, 6-2, 7-1, 8-11, 9-10. |
|
Rd 8: |
12-10, 11-9, 1-8, 2-7, 3-6, 4-5. |
|
Rd 9: |
5-12, 6-4, 7-3, 8-2, 9-1, 10-11. |
Rd 10: 12-11, 1-10, 2-9, 3-8, 4-7, 5-6.
Rd 11: 6-12, 7-5, 8-4, 9-3, 10-2, 11-1.
13 or 14 players:
|
Rd 1: |
1-14, 2-13, 3-12, 4-11, 5-10, 6-9, 7-8. |
|
Rd 2: |
14-8, 9-7, 10-6, 11-5, 12-4, 13-3, 1-2. |
|
Rd 3: |
2-14, 3-1, 4-13, 5-12, 6-11, 7-10, 8-9. |
|
Rd 4: |
14-9, 10-8, 11-7, 12-6, 13-5, 1-4, 2-3. |
|
Rd 5: |
3-14, 4-2, 5-1, 6-13, 7-12, 8-11, 9-10. |
|
Rd 6: |
14-10, 11-9, 12-8, 13-7, 1-6, 2-5, 3-4. |
|
Rd 7: |
4-14, 5-3, 6-2, 7-1, 8-13, 9-12, 10-11. |
|
Rd 8: |
14-11, 12-10, 13-9, 1-8, 2-7, 3-6, 4-5. |
|
Rd 9: |
5-14, 6-4, 7-3, 8-2, 9-1, 10-13, 11-12. |
Rd 10: 14-12, 13-11, 1-10, 2-9. 3-8, 4-7, 5-6.
Rd 11: 6-14, 7-5, 8-4, 9-3, 10-2, 11-1, 12-13.
Rd 12: 14-13, 1-12, 2-11, 3-10, 4-9, 5-8, 6-7.
Rd 13: 7-14, 8-6, 9-5, 10-4, 11-3, 12-2, 13-1.
15 or 16 players:
|
Rd 1: |
1-16, 2-15, 3-14, 4-13, 5-12, 6-11, 7-10, 8-9. |
|
Rd 2: |
16-9, 10-8, 11-7, 12-6, 13-5, 14-4, 15-3, 1-2. |
|
Rd 3: |
2-16, 3-1, 4-15, 5-14, 6-13, 7-12, 8-11, 9-10. |
|
Rd 4: |
16-10, 11-9, 12-8, 13-7, 14-6, 15-5, 1-4, 2-3. |
|
Rd 5: |
3-16, 4-2, 5-1, 6-15, 7-14, 8-13, 9-12, 10-11. |
|
Rd 6: |
16-11, 12-10, 13-9, 14-8, 15-7, 1-6, 2-5, 3-4. |
|
Rd 7: |
4-16, 5-3, 6-2, 7-1, 8-15, 9-14, 10-13, 11-12. |
|
Rd 8: |
16-12, 13-11, 14-10, 15-9, 1-8, 2-7, 3-6, 4-5. |
|
Rd 9: |
5-16, 6-4, 7-3, 8-2, 9-1, 10-15, 11-14, 12-13. |
Rd 10: 16-13, 14-12, 15-11, 1-10, 2-9, 3-8, 4-7, 5-6.
Rd 11: 6-16, 7-5, 8-4, 9-3, 10-2, 11-1, 12-15, 13-14.
Rd 12: 16-14, 15-13, 1-12, 2-11, 3-10, 4-9, 5-8, 6-7.
Rd 13: 7-16, 8-6, 9-5, 10-4, 11-3, 12-2, 13-1, 14-15.
Rd 14: 16-15, 1-14, 2-13, 3-12, 4-11, 5-10, 6-9, 7-8.
Rd 15: 8-16, 9-7, 10-6, 11-5, 12-4, 13-3, 14-2, 15-1.
For a double-round tournament it is recommended to reverse the order of the last two rounds be reversed of the first cycle. This is to avoid three consecutive games with the same colour
Varma Tables
Directions for “restricted” drawing of tournament numbers:
- The arbiter should prepare, beforehand, unmarked envelopes each containing one of the sets of numbers A, B, C and D as indicated below in point 5. These envelopes are then respectively placed in larger envelopes, on each of which the quantity of player-numbers contained in the small envelopes is indicated.
- The order in which players draw lots is determined beforehand as follows: The players from the federation with the greatest number of representatives shall draw first. Where two or more federations have the same number of representatives, precedence is determined by the alphabetical order of the FIDE country code. Among players of the same federation, precedence is determined by the alphabetical order of their names.
- For example, the first player of the federation with the largest number of players shall choose one of the large envelopes containing at least enough numbers for his contingent, and then draw one of the numbers from this envelope. The other players from the same contingent shall also draw their numbers from the same envelope. The numbers that remain are available for use by other players.
- The players of the next contingent then choose an envelope, and this procedure is repeated until all players have drawn their numbers.
- The following Varma Table can be used for 9 to 24 players.
- 9/10 players: A: (3, 4, 8); B: (5, 7, 9); C: (1, 6); D: (2, 10)
- 11/12 players: A: (4, 5, 9, 10); B: (1, 2, 7); C: (6, 8, 12); D: (3, 11)
- 13/14 players: A: (4, 5, 6, 11, 12); B: (1, 2, 8, 9); C: (7, 10, 13); D: (3, 14)
- 15/16 players: A: (5, 6, 7, 12, 13, 14); B: (1, 2, 3, 9, 10); C: (8, 11, 15); D: (4,16)
- 17/18 players: A: (5, 6, 7, 8, 14, 15, 16); B: (1, 2, 3, 10, 11, 12); C: (9, 13, 17); D: (4, 18)
- 19/20 players: A: (6, 7, 8, 9, 15, 16, 17, 18); B: (1, 2, 3, 11, 12, 13, 14); C: (5,10, 19); D: (4, 20)
- 21/22 players: A: (6, 7, 8, 9, 10, 17, 18, 19, 20); B: (1, 2, 3, 4, 12, 13, 14, 15); C: (11, 16, 21); D: (5, 22)
- 23/24 players: A: (6, 7, 8, 9, 10, 11, 19, 20, 21, 22); B: (1, 2, 3, 4, 13, 14, 15,16, 17); C: (12, 18, 23); D: (5, 24)
Tie-Break Regulations
- Choice of Tie-Break System
The choice of the tie-break system to be used in a tournament shall be decided in advance and shall be announced prior to the start of the tournament. If all tie-breaks fail, the tie shall be broken by drawing of lots. A play-off is the best system, but it is not always appropriate. For example, there may not be adequate time.
-
- Play-Off
|
Fundamentally this is the fairest way to decide the final ranking of players having equal scores at the end of a tournament. The only problem is that there will not be enough time to organise tie‐break matches with similar playing time as to the main tournament. Therefore, tie‐break matches with very short playing times, mainly rapid or blitz are organised, and this is a different type of tournament. That’s one of the reasons why some players are not happy with playoffs. |
- Adequate time must be set aside for a conclusion to be reached.
- The pairing system and rate of play must be determined in advance of the start of the event.
- All eventualities must be covered in the regulations.
- It is recommended that play-offs only be arranged for disposition of the first place, a championship or qualifying places.
- Where subsidiary places are also being decided during the play-off, each position shall be determined in accordance with the play-off. For example, three players tie: number 1 wins the play-off, number 2 comes second and number 3 third. Number 2 shall receive the second prize.
- Where two players are tied after the first place has been decided, they shall split any prize money to which they are entitled. For example: four players tie, and a knockout is arranged. Players 3 and 4 knocked out in the semi-final shall share the 3rd and 4th prizes equally.
- Where time is limited before a closing ceremony, games between players potentially involved in such ties in the last round may be scheduled to commence earlier than other games in the tournament.
- If there is a play-off it shall commence after a break of at least 30 minutes after the conclusion of the last main game involving players in the play-off. Where there are further stages, there shall be a break of at least 10 minutes between each stage.
- Each game shall be supervised by an arbiter. If there is a dispute, the matter shall be referred to the Chief Arbiter. His decision shall be final.
- Initial colours shall be determined by lot in all cases below.
- The following is an example where time for play-off is somewhat limited.
-
- If two players have to play a tie-break match:
- They play a two-game mini-match at the rate of all the moves in 3 minutes with 2 seconds added on for each move from move 1. If this match is tied:
- A new drawing of lots for colours shall take place. The winner shall be the first winner of a game. After each odd-numbered game the colours shall be reversed.
- If three players have to take part in a play-off:
- They play a one-game round robin at the rate as in 1 (a). If all three players again tie:
- The next tie-break shall be used (see G.), and the lowest-placed player eliminated. The procedure is then as in (1) (a).
- If four players have to take part in a play-off they play a knockout: The pairings shall be determined by lot.
There shall be two-game elimination matches at the rate as in (1) (a).
-
- If five or more players have to take part in a play-off, they are ranked by the next tie-break (see G.) and all but the top four are eliminated.
- The right is reserved to make necessary changes.
- Where only two players are involved in the play-off, they may play at a slower rate of play, if time permits, by agreement with the CA and CO.
-
- Other Commonly Used Tie-Break Systems
In all systems the players shall be ranked in descending order of the respective system. The following list is simply in alphabetical order.
- Explanations of Tie-Break Systems
- Average Rating of Opponents
The Average Rating of Opponents (ARO) is the sum of the ratings of the opponents of a player, divided by the number of games played.
(a1) The Average Rating of Opponents Cut (AROC) is the Average Rating of Opponents, excluding one or more of the ratings of the opponents, starting from the lowest-rated opponent.
- Buchholz System
The Buchholz System is the sum of the scores of each of the opponents of a player.
(b1) The Median Buchholz is the Buchholz reduced by the highest and the lowest scores of the opponents.
(b2) The Median Buchholz 2 is the Buchholz score reduced by the two highest and the two lowest scores of the opponents.
(b3) The Buchholz Cut 1 is the Buchholz score reduced by the lowest score of the opponents.
(b4) The Buchholz Cut 2 is the Buchholz score reduced by the two lowest scores of the opponents.
- Direct Encounter
If all the tied players have met each other, the sum of points from these encounters is used. The player with the highest score is ranked number 1 and so on. If some but not all have played each other, the player with a score that could not be equalled by any other player (if all such games had been played) is ranked number 1 and so on.
- Koya System for Round-Robin Tournaments
This is the number of points achieved against all opponents who have achieved 50 % or more.
(d1) The Koya System Extended
The Koya system may be extended, step by step, to include score groups with less than 50 %, or reduced, step by step, to exclude players who scored 50 % and then higher scores.
- Number of Games played with the Black Pieces
The greater number of games played with the black pieces (unplayed games shall be counted as played with the white pieces).
- Sonneborn-Berger System
(f1) Sonneborn-Berger for Individual Tournaments is the sum of the scores of the opponents a player has defeated and half the scores of the players with whom he has drawn.
(f2) Sonneborn-Berger for Team Tournaments is the sum of the products of the scores made by each opposing team and the score made against that team.
- Team Competitions
(g1) Match points in team competitions that are decided by game points. For example:
2 points for a won match where a team has scored more points than the opposing team.
1 point for a drawn match. 0 points for a lost match.
(g2) Game points in team competitions that are decided by match points. The tie is broken by determining the total number of game points scored.
- Tie-Break Systems using both the Player’s and the Opponents’ Results
- Sonneborn-Berger
- The Koya System for Round-Robin Tournaments (b1) The Koya System Extended
- Number of games won
- Direct encounter
- Tie-Break Systems using a Team's Own Results
- Match points in team competitions
- Game points in team competitions that are decided by match points
The tie is broken by determining the total number of game points scored.
- Direct encounter
- Tie-Break Systems using the Opponent’s Results
|
Note: all these scores are determined in each case after the application of the rule concerning unplayed games. |
- Buchholz System (a1) Median Buchholz (a2) Median Buchholz 2 (a3) Buchholz Cut 1 (a4) Buchholz Cut 2
(a5) Sum of Buchholz: the sum of the Buchholz scores of the opponents
- Sonneborn-Berger System
(b1) Sonneborn-Berger for Individual Tournaments
(b2) Sonneborn-Berger for Team Tournaments A: the sum of the products of the match points made by each opposing team and the match points made against that team, or
(b3) Sonneborn-Berger for Team Tournaments B: the sum of the products of the match points made by each opposing team and the game points made against that team, or
(b4) Sonneborn-Berger for Team Tournaments C: the sum of the products of the game points made by each opposing team and the match points made against that team, or
(b5) Sonneborn-Berger for Team Tournaments D: the sum of the products of the game points made by each opposing team and the game points made against that team
(b6) Sonneborn-Berger for Team Tournaments Cut 1 A: the sum of the products of the match points made by each opposing team and the match points made against that team, excluding the opposing team who scored the lowest number of match points, or
(b7) Sonneborn-Berger for Team Tournaments Cut 1 B: the sum of the products of the match points made by each opposing team and the game points made against that team, excluding the opposing team who scored the lowest number of match points, or
(b8) Sonneborn-Berger for Team Tournaments Cut 1 C: the sum of the products of the game points made by each opposing team and the match points made against that team, excluding the opposing team who scored the lowest number of game points, or
(b9) Sonneborn-Berger for Team Tournaments Cut 1 D: the sum of the products of the game points made by each opposing team and the game points made against that team, excluding the opposing team who scored the lowest number of game points.
- Tie-Break Systems using Ratings in Individual Tournaments (where all the players are rated)
When a player has elected not to play more than two games in a tournament, his ARO or AROC shall be considered to be lower than that of any player who has completed more of the schedule.
- ARO {See 2.A.(a)}
- AROC {See 2.A.(a1)}
- Handling Unplayed Games for Calculation of Buchholz
For tie-break purposes all unplayed games in which players are indirectly involved (results by forfeit of opponents) are considered to have been drawn.
For tie-break purposes a player who has no opponent will be considered as having played against a virtual opponent who has the same number of points at the beginning of the round and who draws in all the following rounds. For the round itself the result by forfeit will be considered as a normal result.
This gives the formula:
Svon = SPR + (1 – SfPR) + 0.5 * (n – R)
where for player P who did not play in round R: n = number of completed rounds
Svon = score of virtual opponent after round n SPR = score of P before round R
SfPR = forfeit score of P in round R
Example 1: in Round 3 of a nine-round tournament Player P did not show up. Player P’s score after 2 rounds is 1.5. The score of his virtual opponent is Svon = 1.5 + (1 – 0) + 0.5 * (3 – 3) = 2.5 after round 3
Svon = 1.5 + (1 – 0) + 0.5 * (9 – 3) = 5.5 at the end of the tournament
Example 2: in Round 6 of a nine-round tournament player P’s opponent does not show up.
Player P’s score after 5 rounds is 3.5. The score of his virtual opponent is: Svon = 3.5 + (1 – 1) + 0.5 * (6 – 6) = 3.5 after round 6
Svon = 3.5 + (1 – 1) + 0.5 * (9 – 6) = 5.0 at the end of the tournament
- Recommended Tie-Break Systems
For different types of tournaments the Tie-Break Rules are as listed below and are recommended to be applied in the listed order.
- Individual Round-Robin Tournaments:
Direct encounter
The greater number of wins Sonneborn-Berger
Koya System
|
Remark: Don’t use Buchholz systems for Round Robin tournaments |
- Team Round-Robin Tournaments:
Match points (if ranking is decided by game points), or Game points (if ranking is decided by match points) Direct encounter
Sonneborn-Berger
- Individual Swiss Tournaments where not all the ratings are consistent:
Direct encounter
The greater number of wins
The greater number of games with Black (unplayed games shall be counted as played with White)
Buchholz Cut 1 Buchholz Sonneborn-Berger
- Individual Swiss Tournaments where all the ratings are consistent:
Direct encounter
The greater number of wins
The greater number of games with Black (unplayed games shall be counted as played with White)
AROC
Buchholz Cut 1 Buchholz Sonneborn-Berger
- Team Swiss Tournaments:
Match points (if ranking is decided by game points), or Game points (if ranking is decided by match points) Direct encounter
Buchholz Cut 1 Buchholz Sonneborn-Berger
Examples to explain the virtual opponent system ‐ we are in round 5 of a 11 round tournament
-
- in case of a bye
|
|
player "A" |
virtual opponent |
|
points before the round |
2 |
2 |
|
result of the round |
1 |
0 |
|
points after the round |
3 |
2 |
|
points for the subsequent rounds |
? |
3 |
|
points at the end of the tournament |
? |
5 |
-
- in case of a “+ / ‐ “
|
|
player "A" |
virtual opponent |
|
points before the round |
2 |
2 |
|
result of the round |
1 |
0 |
|
points after the round |
3 |
2 |
|
points for the subsequent rounds |
? |
3 |
|
points at the end of the tournament |
? |
5 |
-
- in case of a “ ‐ / + “
|
|
player "A" |
virtual opponent |
|
points before the round |
2 |
2 |
|
result of the round |
0 |
1 |
|
points after the round |
2 |
3 |
|
points for the subsequent rounds |
? |
3 |
|
points at the end of the tournament |
? |
6 |
|
If the tie‐break system is not fixed in the existing tournament regulations, it is up to the organiser to decide the system. The type of the tournament has to be taken in account (Round Robin, Swiss System, Team Tournament, and so on) and the structure of the expected participants (such as juniors, seniors, rated or unrated players).
Whatever system used, it must be announced by the organiser in advance or by the Chief Arbiter before the start of the first round. |
Awarding of money prizes
If two or more players finish a tournament with equal points the organizers have three possibilities to award money prizes:
-
-
- money prizes will be shared equally.
- money prizes will be given according to the tie‐break results.
- money prizes will be calculated by using the Hort system, which is a combination of „a“ and „b“.
-
In Hort system 50% of the prize money is given according the tie‐break ranking. The second half of the prize money of all the players, having the same number of points at the end, is added together and shared equally.
|
|
system a) |
system b) |
system c) |
|
|
|
A ‐ |
5.000 € |
10.000 € |
5.000 + |
2.500 = |
7.500 € |
|
B ‐ |
5.000 € |
5.000 € |
2.500 + |
2.500 = |
5.000 € |
|
C ‐ |
5.000 € |
3.000 € |
1.500 + |
2.500 = |
4.000 € |
|
D ‐ |
5.000 € |
2.000 € |
1.000 + |
2.500 = |
3.500 € |
|
Example: The prizes in the tournament are: 1st place 10.000 € 2nd place 5.000 € 3rd place 3.000€ 4th place 2.000€ Players A, B, C and D finish a tournament with 8 points each. The Buchholz points are: A has 58 Buchholz points B has 57 Buchholz points C has 56 Buchholz points D has 54 Buchholz points. The money prizes for A, B, C and D ‐ depending on the system used ‐ will be: |
|
The total is €20,000€ whatever system is used. |
Organizers have to decide in advance and to inform the players before the start of the tournament which system will be used for calculation of money prizes.
Additionally, in systems a) and c) the organizers have to decide and to inform the participants how many players will have the right to be awarded with money prizes in case of equal points after the last round.
|
If it is announced to give 10 money prizes and the final ranking is: players ranked 1 to 4 have 8 points players ranked 5 to 9 have 7.5 points players ranked 10 to 20 have 7 points. In such a case it is not wise to share the money for rank 10 between 11 players. To avoid such a problem it should be announced in advance that money prizes are equally shared or given by Hort system to the players ranked on 1 to 10. |
|
The distribution of the prize money is better done by two people working independently. This might be the Treasurer and Chief Arbiter. |
Comparison of several tie‐break criteria in an artificial round robin tournament:
| name | rtg | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | points | SB | Koya | Rp |
| Alexander | 2269 | * | 1 | 1 | ½ | 0 | 1 | 1 | ½ | 1 | ½ | ½ | 1 | 8 | 42 | 3½ | 2414 |
| Joseph | 2171 | 0 | * | ½ | 1 | 1 | 0 | ½ | 1 | 1 | 1 | 1 | 0 | 7 | 36,75 | 2½ | 2350 |
| Robert | 2276 | 0 | ½ | * | ½ | 0 | 1 | 0 | 1 | 1 | 1 | ½ | 1 | 6½ | 31,75 | 2 | 2304 |
| Walter | 2290 | ½ | 0 | ½ | * | 1 | 1 | ½ | ½ | ½ | ½ | ½ | ½ | 6 | 32,5 | 3 | 2273 |
| Peter | 2273 | 1 | 0 | 1 | 0 | * | ½ | ½ | 0 | 1 | ½ | ½ | 1 | 6 | 32 | 2½ | 2275 |
| Olaf | 2299 | 0 | 1 | 0 | 0 | ½ | * | 1 | 1 | 0 | 1 | 1 | ½ | 6 | 30,25 | 1½ | 2273 |
| Mark | 2281 | 0 | ½ | 1 | ½ | ½ | 0 | * | ½ | ½ | 0 | ½ | 1 | 5 | 25,75 | 2½ | 2202 |
| Ivan | 2333 | ½ | 0 | 0 | ½ | 1 | 0 | ½ | * | ½ | 0 | 1 | 1 | 5 | 25 | 2 | 2198 |
| Sandor | 2233 | 0 | 0 | 0 | ½ | 0 | 1 | ½ | ½ | * | ½ | 1 | 1 | 5 | 23,25 | 1½ | 2207 |
| Martin | 2227 | ½ | 0 | 0 | ½ | ½ | 0 | 1 | 1 | ½ | * | 0 | ½ | 4½ | 23,75 | 1½ | 2178 |
| Frederik | 2340 | ½ | 0 | ½ | ½ | ½ | 0 | ½ | 0 | 0 | 1 | * | 1 | 4½ | 22,75 | 2 | 2168 |
| Valery | 1910 | 0 | 1 | 0 | ½ | 0 | ½ | 0 | 0 | 0 | ½ | 0 | * | 2½ | 15,25 | 2 | 2061 |
Comparison of several tie‐break criteria in a swiss tournament
using the final results of the European Individual Championship 2011 in Aix‐les‐ Bains, France:
| Rk | title | name | rtg | fed | pt | Rp‐2 | Rp | BH | m BH | SB |
| 1 | GM | Potkin Vladimir | 2653 | RUS | 8½ | 2849 | 2822 | 78 | 63½ | 59,25 |
| 2 | GM | Wojtaszek Radoslaw | 2711 | POL | 8½ | 2826 | 2812 | 77 | 63 | 58,5 |
| 3 | GM | Polgar Judit | 2686 | HUN | 8½ | 2799 | 2781 | 77 | 63½ | 58,25 |
| 4 | GM | Moiseenko Alexander | 2673 | UKR | 8½ | 2755 | 2790 | 74½ | 62 | 56,5 |
| 5 | GM | Vallejo Pons Francisco | 2707 | ESP | 8 | 2819 | 2764 | 80 | 66½ | 57,75 |
| 6 | GM | Ragger Markus | 2614 | AUT | 8 | 2783 | 2768 | 76 | 62½ | 54,25 |
| 7 | GM | Feller Sebastien | 2657 | FRA | 8 | 2766 | 2763 | 70½ | 58½ | 49 |
| 8 | GM | Svidler Peter | 2730 | RUS | 8 | 2751 | 2757 | 76½ | 62½ | 54,75 |
| 9 | GM | Mamedov Rauf | 2667 | AZE | 8 | 2751 | 2754 | 74 | 61 | 52,25 |
| 10 | GM | Vitiugov Nikita | 2720 | RUS | 8 | 2741 | 2744 | 76½ | 63 | 54,5 |
| 11 | GM | Zhigalko Sergei | 2680 | BLR | 8 | 2732 | 2731 | 72 | 59½ | 50 |
| 12 | GM | Jakovenko Dmitry | 2718 | RUS | 8 | 2719 | 2704 | 72½ | 60 | 53 |
| 13 | GM | Korobov Anton | 2647 | UKR | 8 | 2697 | 2740 | 75 | 61½ | 53,5 |
| 14 | GM | Inarkiev Ernesto | 2674 | RUS | 8 | 2695 | 2735 | 72½ | 60 | 51,5 |
| 15 | GM | Postny Evgeny | 2585 | ISR | 8 | 2633 | 2676 | 64 | 52 | 44,75 |
| 16 | GM | Azarov Sergei | 2615 | BLR | 7½ | 2776 | 2723 | 75 | 62½ | 47,5 |
| 17 | GM | Khairullin Ildar | 2634 | RUS | 7½ | 2771 | 2720 | 74½ | 61½ | 49 |
| 18 | GM | Kobalia Mikhail | 2672 | RUS | 7½ | 2754 | 2716 | 70½ | 57 | 45,5 |
| 19 | GM | Zherebukh Yaroslav | 2560 | UKR | 7½ | 2739 | 2712 | 71½ | 59 | 45,5 |
| 20 | GM | Guliyev Namig | 2522 | AZE | 7½ | 2739 | 2652 | 71 | 59½ | 45,5 |
| 21 | GM | Riazantsev Alexander | 2679 | RUS | 7½ | 2728 | 2687 | 72½ | 60 | 48,75 |
| 22 | GM | Iordachescu Viorel | 2626 | MDA | 7½ | 2725 | 2716 | 76 | 62 | 50,25 |
| 23 | GM | Lupulescu Constantin | 2626 | ROU | 7½ | 2722 | 2677 | 71 | 58 | 46 |
| 24 | GM | Mcshane Luke J | 2683 | ENG | 7½ | 2718 | 2684 | 72½ | 59 | 47 |
| 25 | GM | Fridman Daniel | 2661 | GER | 7½ | 2717 | 2684 | 69 | 56½ | 45,25 |
| 26 | GM | Motylev Alexander | 2677 | RUS | 7½ | 2716 | 2710 | 71 | 59 | 47,5 |
| 27 | GM | Ivanisevic Ivan | 2617 | SRB | 7½ | 2712 | 2704 | 71 | 58½ | 47 |
| 28 | GM | Jobava Baadur | 2707 | GEO | 7½ | 2711 | 2656 | 71½ | 58 | 47,5 |
| 29 | GM | Parligras Mircea‐ Emilian | 2598 | ROU | 7½ | 2709 | 2735 | 78½ | 65 | 50,75 |
| 30 | GM | Romanov Evgeny | 2624 | RUS | 7½ | 2709 | 2668 | 68½ | 55½ | 43,75 |
| 31 | GM | Esen Baris | 2528 | TUR | 7½ | 2707 | 2669 | 73 | 61 | 47,25 |
| 32 | GM | Nielsen Peter Heine | 2670 | DEN | 7½ | 2703 | 2707 | 67½ | 55 | 45,5 |
| 33 | GM | Cheparinov Ivan | 2664 | BUL | 7½ | 2698 | 2693 | 75 | 62 | 49,75 |
| 34 | GM | Gustafsson Jan | 2647 | GER | 7½ | 2687 | 2687 | 67 | 55 | 45 |
| 35 | GM | Kulaots Kaido | 2601 | EST | 7½ | 2669 | 2633 | 67½ | 54½ | 44 |
| 36 | GM | Smirin Ilia | 2658 | ISR | 7½ | 2668 | 2675 | 69 | 56½ | 47,25 |
| 37 | GM | Saric Ivan | 2626 | CRO | 7½ | 2651 | 2692 | 72½ | 58½ | 47 |
| 38 | GM | Pashikian Arman | 2642 | ARM | 7½ | 2649 | 2640 | 68 | 55½ | 46 |
| 39 | GM | Edouard Romain | 2600 | FRA | 7½ | 2634 | 2602 | 66 | 52½ | 42,5 |
| 40 | GM | Bologan Viktor | 2671 | MDA | 7½ | 2629 | 2673 | 68½ | 56 | 45,75 |
|
Rating prizes are another type of prize. Perhaps the best achievement by a player rated 2300-2399 and another 2200-2299. Do not fall into the trap of writing U2400, U2300. In that case the U2300 player might get both prizes. A good way of awarding these is not just on score, or Tournament Performance Rating. Best Improvement in Rating as measured by W-We. W is the score achieved, We is the expected score against the average strength of the opponents. This has the advantage that ties are almost unknown. It is possible, in a Swiss, for a player with a lower score to get the greater achievement. |
GENERAL RULES AND RECOMMENDATIONS FOR TOURNAMENTS
STANDARDS OF CHESS EQUIPMENT FOR FIDE TOURNAMENTS
-
- Chess Equipment
- The chess equipment offered by the organisers (hosts) of a FIDE or Continental Championship, Olympiad and other FIDE registered tournaments shall conform with the standards mentioned below, and shall be approved by the Chief Organiser and the Chief Arbiter.
- It is recommended, that the chess pieces, boards and clocks, used in the World or Continental top level competitions be approved by participating players. Their approval shall be obtained for other equipment the table, chairs etc. In case either side disagrees, the equipment to be used shall be decided by the Chief Organiser or the Chief Arbiter of the event, bearing in mind the standards for its size and form as mentioned below.
- It is highly recommended that the chess equipment used in a competition is the same for all participants and all games.
-
- Chess Pieces
- Material
Chess pieces should be made of wood, plastic or an imitation of these materials.
-
- Height, weight, proportions
The size of the pieces should be proportionate to their height and form; other elements such as stability, aesthetic considerations etc., may also be taken into account. The weight of the pieces should be suitable for comfortable moving and stability.
Recommended height of the pieces is as follows: King - 9.5 cm, Queen - 8.5 cm, Bishop
- 7 cm, Knight - 6 cm, Rook - 5.5 cm and Pawn - 5 cm. The diameter of the piece's base should measure 40¬50% of its height. These dimensions may differ up to 10% from the above recommendation, but the order (e.g. King is higher than Queen etc.) must be kept.
-
- Form, style of pieces
Recommended for use in FIDE competitions are pieces of Staunton style. The pieces should be shaped so as to be clearly distinguishable from one another. In particular the top of the King should distinctly differ from that of the Queen. The top of the Bishop may bear a notch or be of a special colour clearly distinguishing it from that of the Pawn.
Examples of chess pieces:

Original Staunton chess pieces, left to right: pawn, rook, knight, bishop, queen, and king
A modern Staunton set, in wood

World Chess set approved by FIDE for the 2013 Candidate Tournament in London


-
- 2.4. Colour of the pieces
The "black" pieces should be brown or black, or of other dark shades of these colours. The "white" pieces may be white or cream, or of other light colours. The natural colour of wood (walnut, maple, etc.) may also be used for this purpose. The pieces should not be shiny and should be pleasing to the eye.

The initial position of the pieces – see FIDE Laws of Chess art.2
- Chess boards
- Material and colour
For the World or Continental top level competitions wooden boards should be used. For other FIDE registered tournaments boards made of wood, plastic or card are recommended. In all cases boards should be rigid. The board may also be of stone or marble with appropriate light and dark colours, provided the Chess Organiser and Chief Arbiter find it acceptable. Natural wood with sufficient contrast, such as birch, maple or European ash against walnut, teak, beech, etc., may also be used for boards, which must have a dull or neutral finish, never shiny. Combination of colours such as brown, green, or very light tan and white, cream, off-white ivory, buff, etc., may be used for the chess squares in addition to natural colours.
-
- Size of the square and the board
The side of the square should measure 5 to 6 cm. Referring to 2.2 the side of a square should be at least twice the diameter of a pawn's base (that means four pawns on one square). A comfortable table of suitable height may be fitted in with a chessboard. If the table and the board are separate from one another, the latter must be fastened and thus prevented from moving during play.
- Chess tables
For all official FIDE tournaments, the length of the table is 110 cm (with 15% tolerance). The width is 85 cm (for each player at least 15 cm). The height of the table is 74 cm. The chairs should be comfortable for the players. Special dispensation should be given for children's events. Any noise when moving the chairs must be avoided.
- Chess clocks
For the FIDE World or Continental Championships and Olympiads electronic chess clocks must be used. For other FIDE registered tournaments organisers are recommended to use also electronic chess clocks.
If mechanical chess clocks are used, they should have a device (a "flag") signalling precisely when the hour hand indicates the end of each hour. The flag must be arranged so that its fall can be clearly seen, helping the arbiters and players to check time. The clock should not be reflective, as that may make it difficult to see. It should run as silently as possible in order not to disturb the players during play.
The same type of clock should be used throughout the tournament.
-
- Requirements for electronic chess clocks
-
- In approved clocks, when one clock reaches zero in an increment mode time control, the other clock does not run further and retains its last display. For Rapid and Blitz tournaments, when one of the clocks reaches zero, the other clock may be set to continue to run until it also reaches zero.
- It is advantageous in Rapid and Blitz play as, when both flags have fallen, the game is drawn. If a player does not notice the flag fall of his opponent's clock, his clock will also display zero so that the game is drawn.
- When the approved clocks are used, the player whose flag falls first has a disadvantage and the other player, who has some time left on his clock, has a definite advantage. This is a disparity to the players.
- Clocks must function in full accordance with the FIDE laws of chess.
- The display at all times should show the time available to complete a player's next move (It is preferable to display seconds from beginning).
- The displays must be legible from a distance of at least 3 metres.
- A player must have a clearly visible indication which clock is running from a distance of 10 metres.
-
-
-
- For standard play games, where the time control has been passed, a sign on the display must signal clearly which player passed the time control first.
- For battery powered clocks, a low-battery indication is required.
- The clock must continue to function correctly for at least 10 hours after the low battery indication.
- Special attention should be given to the correct announcement of passing time controls.
- In case of cumulative or time delay modes, the clock should not add any additional time when a player passes the last time control.
- In case of time penalties, it should be possible for time and move counter corrections to be executed by an arbiter within 60 seconds.
- It must be impossible to erase or change the data in display with one simple action.
- Clocks must have a brief user manual for the clock.
- Electronic chess clocks used for FIDE events must be endorsed by the FIDE Technical Commission.
-
-
- The electronic chess clocks endorsed by FIDE
- DGT XL (year 2007)
- DGT 2010 (year 2010)
- Silver Timer (year 2007)
- Sistemco (year 2009)
- DGT 3000 (year 2014)
-
- Electronic score sheets
- General remarks
- An electronic score sheet is a replacement for paper versions. It makes it easier to reconstruct games for publication where no other means of move registration is used.
- It is a device where a player can notate his and his opponent's moves during a game electronically.
- Basic rules for this electronic score sheet (device):
- The device is dedicated for notating chess games (not a multipurpose computer).
- The device fully complies with FIDE rules.
- The game notation complies with FIDE Laws of Chess, whereas the use of figurines is allowed.
-
-
-
- The device can be linked to the owner or player through some unique identification of the device.
- The device logs user actions during game mode to prevent or detect foul play.
- It is foreseen that both players and tournament organizations will buy and use their own devices.
- The device should have approximately the size of A5-A6 (paper size).
-
- Game mode
- This is the mode where the player notates his game. The switch from any other mode to game mode can be made by the player himself when the game is finished or by the tournament organization or arbiter.
- The following rules apply to the electronic score sheet in game mode:
- During the game it is not possible to switch to any other mode.
- The game notation is clearly visible for the arbiter, with the restriction that not all moves need to be visible.
- The state of the device being in game mode is clearly visible for everyone.
- It is not allowed to go out of game mode by accident or deliberately, without notifying this to the player, his opponent or arbiter. This is also clearly visible to all parties.
- If the battery has low power this must be signalled. When this is signalled, the battery must hold out at least 8 hours to make it possible to notate a complete game.
- A minimum of 7 moves must be visible in a move list.
- Graphical input through a chess board with figurines is allowed.
- Scrolling through the move list is allowed, as is correcting of incorrect entered moves.
- A game finishes when a result is noted and both players signed the score sheet. The arbiter signature is optional.
- The players are obliged to submit the text of their game to the Organizer with reference to article 8.3 of the Laws of Chess.
- On entering moves:
-
- It is allowed to enter an illegal move;
- It is allowed to enter the clock time, draw offers and other abbreviation according to Laws of chess. Input of clock times should be possible using a figurine notation;
- It is allowed to enter only moves of white or black during time trouble;
- It is allowed to enter a dash for a move during time trouble;
- The device is not allowed to correct or signalling illegal moves automatically;
- If a stale mate or check mate is missed or an illegal move is made by the player, the device must be able to record following moves.
- An automatic move counter should be available
-
-
- The device must be able to restart the notation.
-
- Arbiter mode
- The arbiter mode is an optional mode for the device. This mode is created to give the arbiter some extra features supporting his job.
- If there is an arbiter mode available the following rules apply:
- Only the arbiter (or a representative of the tournament organization) is allowed to enter this mode during a game.
- In this mode legality checks may be done on the moves played in the game:
- Threefold repetition of a position (fivefold repetition)
- 50 moves rule (75 moves rule)
- Detection of stalemate or checkmate.
- The arbiter can take moves back in case an illegal move is detected.
- Owner mode
- The owner mode is an optional mode for the device. This is a mode where the producer may add some chess features for creating an attractive product for their customers.
- If there is owner mode available the following rules apply:
- The identification of the owner shall be possible in owner's mode.
- This mode is only allowed when not playing a game. Otherwise it is completely locked out.
- No chess program is allowed i.e. this is not a chess computer.
-
-
-
-
- No other then chess related activities are allowed.
- For anybody it is easy to see that the device is in owner mode.
-
-
-
- Testing Clocks and equipment
- The FIDE Technical Commission is competent to decide whether or not any piece of equipment is suitable for use in FIDE competitions. The Commission may recommend the use of other types of chess sets in addition to those mentioned above. It may make a list of equipment with satisfactory standards, the specimen of which would be kept at the FIDE Secretariat.
- If necessary FIDE will determine the general conditions for other equipment needed in chess competitions, such as score sheets, demonstration boards, etc.
- Tournament halls for the FIDE World or Continental Championships and Olympiads
- Inspection and preparation of the Playing Hall
- All areas to which players have access during play should be inspected carefully and repeatedly by the Chief Organiser and the Chief Arbiter.
- Space for spectators must be prepared. The distance between the chess boards and the spectators should be not less than one meter, for top level tournaments 1.5 meters.
- Lighting of a standard similar to that used for examinations should be about 800 lux. Lighting should not cast shadows or cause pinpoints of light to be reflected from the pieces. Beware of direct sunlight, especially if this varies during play.
- Inspection and preparation of the Playing Hall
For a high-level tournaments The organizer should have the possibility (the device) to adjust the light in the hall - quality of lighting covering a larger area to the same level of flux requires a greater number of lumens.
-
-
- It is highly recommended that the hall be carpeted. The noise made by moving chairs must be avoided.
- The extraneous noise levels close to the tournament hall must be checked too.
-
- Space for players and arbiters
- It is recommended that the minimal space of 4 square meters be available for each player in individual matches and round robin tournaments. For other tournaments 2 square meters may be adequate. (Please refer to Diagram-A)
-
Some definitions and recommendations regarding sizes L : Length of the table.
L = 110 cm, tolerances: +20 cm, -10 cm W : Width of the table.
W = 85 cm, tolerances: +5 cm, -5 cm. S : Horizontal space between table rows.
S = 3m, tolerances: +1.5 m, -0.5 m. R : Vertical space between table rows. R = 3m, tolerances: +1.5 m, -0.5 m.
-
-
- There should be a minimum of 2.5 meters between rows of players. It is best not to have long, unbroken rows. Where possible, players should play on individual tables at least for top boards or top matches in the events. (Please refer to Diagram-B)
-
Diagram B
Basic tournament hall placement styles
|
Dual Row For large events (open tournaments, youth champ. etc) (an arbiter may check two table in a same time) |
|
Single Row Preferable style for individual competitions |

|
Multi Row For team competitions (should be avoided for individual events as much as possible) |

-
-
- Special tables with the connection to the Internet for arbiters should be arranged too.
- Games should not be placed too close to doors.
- Playing conditions for all players in the event (especially for both players in a game) should be equalled as much as possible. Exceptions are mentioned in (b).
-
-
- Broadcasting
- All official FIDE events must be broadcast on the Internet
- All games from World Championship Matches, World Cup, Olympiad, World Team Championship and GP FIDE.
- At least 10 games from each age category of World Youth and Cadet Championships.
- As many games as possible from all other championships, but at least 30 games.
- Delay of broadcasting should be decided by the Chief Organiser and Chief Arbiter.
- Requirements on treatment of disabled chess players
- General remarks
- These guidelines will be used for all FIDE rated events.
- No one has the right to refuse to meet a disabled player against whom he has been correctly paired.
- All chess venues must either be accessible to all, or an acceptable alternative venue with full supervision shall be available to those who cannot access the nominated venue.
- A circular shall be sent out when all competitors are known. This circular contains an entry form with the usual points and questions, asking whether any potential competitor has an impairment that will require special circumstances. The competitor has to inform the organisers about the special circumstances at least 20 days before the start of the event.
- No disabled player shall be "penalised" in accordance with the Articles
- General remarks
-
-
- 6.7 and 8.1.6 of the Laws of Chess because of disability.
- It is recommended, that in all events there should be a tournament physician. The Chief Organiser and the Chief Arbiter shall know the phone number of the local hospital and physician.
- It is recommended that each national chess federation appoints an officer for matters regarding disabilities.
- It is strongly recommended that all organisers of chess events adopt these guidelines.
-
-
-
- Special arrangements for participants
- Any impaired competitor who reasonably requests in time the placing of their equipment in a particular seat or orientation, has the right to do so, provided that this does not disadvantage his opponent or other competitors. The event organizer has to ensure that the needs of both players are catered for.
- All relevant information shall be displayed before the start of the event, including maps of the venue showing the location of toilets, refreshments and emergency exits.
- If a competitor cannot access the refreshments, arrangements should be made for their needs to be met.
- If a competitor cannot press his own clock or move his own pieces, an assistant shall be available unless the opponent is willing to do so. If the opponent is acting as an assistant the Chief Arbiter may decide to give him extra thinking time.
- If a player has made a prior request, copies of all notices should be available in large print. If a player is unable to read large print, then the notices must be read to him.
- It is recommended that all team events have the rule that if a visiting team indicates that it has a player with an impairment coming with them, giving sufficient notice, that the home team does everything which is reasonable to ensure that that player can participate.
-
- Organisation of the tournament hall
- Only one game per table: in case an assistant is needed the tables should be larger (2 m width in order to place the assistants for the disabled) and should be placed separately.
- The corridors between rows of tables should be twice as large (wheel chairs)
- The arbiters should be clearly accessible to all players.
- Foresee additional contact points for electricity: some visually disabled players use a lamp for their chess board. This lamp should not disturb the opponent.
- Put the blind chess players at the same place as much as possible (they will know the way to the rest room and back in very short time!) and give them the same assistant during the whole tournament.
-
- Assistants
- The assistants should have a minimum knowledge of chess; the language is less important since most of the handicapped players only speak their mother tongue.
-
-
-
- Assistants for blind players should know the name of the pieces in their language
- Assistants for blind players should inform the player when they are leaving the chess board temporarily.
- The assistant should always write the moves: this is an important help for the arbiter.
-
- Tournament organisation and Chief Arbiter
- Organise a players meeting for all players before the first round, preferably in the tournament hall.
- If possible only one round per day should be played.
- After making the pairings the chief arbiter should decide manually on which board everyone should play: some players (visually handicapped) should always play at the same board whereas the largest space should be foreseen for wheelchair players.
- Draw proposals or claims can easily go via the assistant. All players push the clock themselves, except the players who are physically unable to do so.
- In the case there is a time trouble situation with visually disabled players the arbiter should bear in mind that the (not visually disabled) opponent can reply almost immediately. The tournament regulations should therefore release the visually disabled player from the obligation to record the moves during the last five minutes, even when the game is played with an increment of at least 30 seconds. The visually handicapped player should then update his score sheet after the time trouble.
-
- Requirements on treatment of school tournaments
- General remarks
- These guidelines shall be observed for all school tournaments played under FIDE auspices or that are to be FIDE rated and ideally should also be followed by national and regional school tournaments, especially those that may be nationally rated.
- General remarks
These guidelines may also be useful indications for ordinary school chess which is often described as "non-competitive" (games are usually played without clocks and not usually notated) in cases where the organizer is trying to introduce players to the world of "competitive" chess.
-
-
- Every player should have the accompanying person who will be an attendant.
- The attendant may help the player to find the table.
- During a game all attendants, parents, coaches are treating as spectators. They should stay in place for spectators and cannot interfere with a game in progress. In case of questionable situation may contact only the arbiter or the organizer.
- Attendants can't use any mobile phone or electronic device in the playing hall.
- Using cameras with flash is restricted to the first five minutes of each round.
-
-
- Organisers and their duties
- Organisers are obliged to prepare the invitation and the regulation that shall be as comprehensive as possible, stating clearly the expected conditions and giving all details which may be of use to the participants:
- name, address (including e-mail, fax and telephone numbers) of the organizers,
- date and venue of the event,
- the hotel(s) where the players are to stay (including e-mail and telephone numbers), also regarding provided the board and lodging,
- requirements for the participants (e.g. registration date),
- tournament schedule (with the annotation of players confirmation, approximate game-time and estimated time of awards ceremony),
- the rate of play and tie-break system,
- the default-time,
- the prizes, gifts, diploma and important diploma for the participation.
- Organisers are obliged to prepare the invitation and the regulation that shall be as comprehensive as possible, stating clearly the expected conditions and giving all details which may be of use to the participants:
- Organisers and their duties
-
-
- The chief organiser should be present in the playing hall during the tournament. He is responsible for preparing the playing hall, opening ceremony and awards ceremony.
- It is recommended to insure one arbiter for every 30 players.
- Before the first round the organiser is obliged to explain to players the tournament regulations and the remind them some basic rules:
- finding the table (numbered), chessboard and the proper colour of the pieces,
- announce that players who lose their game play the next round (unless the rules of a competition specify otherwise),
- touch move rule,
- castling (the first king, later rook, using one hand),
- using the chess clock (start and stop),
- illegal move and it's consequence,
- mobile phone and it's consequence,
- the way of claiming (stop the clock and ask the arbiter),
- the way of announcing the result.
-
-
-
-
- announce, that the arbiter will collect the result at the table of player. He will also check the names of the players before writing the result.
-
-
Note: Some children run to their parents very fast and forget to report the result. Sometimes they give false results when coming to the arbiters place or they change the colour. After that the arbiter has less time to intervene or check who won the game.
-
- Tournament conditions
- If it is possible, all of the games should be played in one playing hall, e.g. in the school gym. The minimal space of two square meters should be available for each player.
- In other cases each playing hall should have at least one arbiter.
- The tables and chairs should be adjusted to the children's height and to the chessboard size.
- It is highly recommended that the chess equipment used in a competition is the same for all participants and all games.
- Chess pieces should be made of wood, plastic or an imitation of these materials.
- Pieces for FIDE Tournaments should be used. If the organizer has difficulties to prepare this kind of equipment, he can use the chessboard with the minimum square size of 55 mm and king's height 90 mm (Staunton no 5). The chessboard with the square size 38 mm and king's height 75 mm (Staunton no 4) is also acceptable in the school tournaments.
- It is necessary to prepare additional chess sets, pieces and chess clocks because they not once are damaged during school tournaments.
- Each chessboard should have coordinates.
- The playing hall should be good marked with the sign indicating the playing area, the spectators’ area, arbiters and organizers tables as well as rest rooms etc.
- If players are taking part in a few groups, it is recommended to indicate the name of the group using different colours and other characters. The same colour can be used for marking the pairings, results etc. It is easier for children to remember colours and find the right group.
- Space for spectators must be prepared and clearly marked. It can be another room or the separated place in the playing hall. The distance between the chessboards and the spectators should not be less than one meter. The rope barrier is requested.
- It is not allowed for the spectators to walk between the chessboard or stay vis-a-vis a supporting player.
- Players become spectators when their game finishes. Players are not allowed to play skittles games in the playing hall.
- Tournament conditions
-
-
- The advertising board should be prepared to display the start lists, pairings, results and other tournament information.
- No food or drink, except for bottled water, will be permitted in the competition area. Bottled water cannot be placed on the table.
- Rate of play and results
- There must be no more than 5-6 hours play for all rounds in one day. Examples: one day 6 round G=15' and 5 rounds G-30' or three days with two rounds G-60'. It could be connected with the possibility of achieving the local chess category.
- Tournaments without chess clocks. After 20 minutes the arbiters give the clock to the players with e.g. 5 minutes for each player to complete the game.
- Player who wins his game, or wins by forfeit, scores one point (1), a player who loses his game, or forfeits, scores no points (0), and a player who draws his game scores a half point (½).
- Tie-break system
- The tie-break system shall be decided in advance and announced prior to the start of the tournament. The arbiter should be ready to clearly clarify the calculations rules of tie-break system to the children and spectators. If all tie-breaks fail, the tie shall be broken by drawing of lots.
- A play-off is the best system, but it is not always appropriate, because it required the additional time. However it is recommended that play-offs be arranged in the case of the first place in the championship or qualifying places.
- The tie-break in Swiss Tournaments:
- The Buchholz Cut 1 (the sum of the scores of each of the opponents of a player reduced by the lowest score of the opponent)
- The Buchholz System (the sum of the scores of each of the opponents of a player)
- The greater number of wins.
- The greater number of wins with Black (unplayed games shall be counted as played with White).
- The tie-break in Round-Robin Tournaments:
- The greater number of wins.
- Sonneborn-Berger (the sum of the scores of the opponents a player has defeated and half the scores of the players with whom he has drawn).
- Koya System (the number of points achieved against all opponents who have achieved 50 % or more)
- The greater number of wins with Black (unplayed games shall be counted as played with White)
-
TYPES OF TOURNAMENTS
To establish the pairings for a chess tournament the following systems may be used:
- Round Robin System
In a Round Robin Tournament all the players play each other. Therefore the number of rounds is the number of participants minus one, in case of an even number of players. If there is an odd number of participants, the number of rounds is equal to the number of players.
Usually the Berger Tables are used to establish the pairings and the colours of each round.
If the number of players is odd, then the player who was supposed to play against the last player has a free day in every round.
The best system for players is a Double Round Robin Tournament, because in such a system all players have to play two games against each opponent, one with white pieces and another one with black pieces. But mainly there is not time enough for it and other systems have to be used.
An example of a cross table of the final ranking of a Round Robin Tournament:
|
2009 China (Nanjing) Pearl Spring Chess Tournament |
||||||||||||||
| Final Ranking crosstable after 10 Rounds | ||||||||||||||
| Rk. |
|
Name | Rtg | FED | 1 | 2 | 3 | 4 | 5 | 6 | Pts. | TB1 | TB2 | TB3 |
| 1 | GM | CARLSEN MAGNUS | 2772 | NOR | *** | 1 ½ | ½ 1 | 1 1 | 1 ½ | 1 ½ | 8 | 6 | 0 | 35 |
| 2 | GM | TOPALOV VESELIN | 2813 | BUL | 0 ½ | *** | ½ ½ | ½ 1 | ½ ½ | ½ 1 | 6 | 2 | 0 | 24,5 |
| 3 | GM | WANG YUE | 2736 | CHN | ½ 0 | ½ ½ | *** | ½ ½ | ½ ½ | ½ ½ | 5 | 0 | 0 | 21,5 |
| 4 | GM | JAKOVENKO DMITRY | 2742 | RUS | 0 0 | ½ 0 | ½ ½ | *** | ½ 1 | ½ ½ | 4 | 1 | 0 | 17,3 |
| 5 | GM | RADJABOV TEIMOUR | 2757 | AZE | 0 ½ | ½ ½ | ½ ½ | ½ 0 | *** | ½ ½ | 4 | 0 | 1 | 20 |
| 6 | GM | LEKO PETER | 2762 | HUN | 0 ½ | ½ 0 | ½ ½ | ½ ½ | ½ ½ | *** | 4 | 0 | 1 | 19,3 |
- Swiss Systems
In FIDE, there are five different Swiss systems to be used for pairings:
-
- The Dutch System
It is the usual Swiss system for open tournaments well known by players and organizers, and will be described in detail later (see paragraph 8: “Annotated rules for the Dutch Swiss System”);
-
- The Lim System
The pairings are made from to top score group down before the middle group, then from the bottom score group to the middle group and finally the middle score group;
-
- The Dubov System
The objective of this system is to equalize the rating average (ARO) of all players. Therefore, in a score group, the white‐seeking players are sorted according to their ARO, the black‐seeking players according to their rating. Then, the white‐seeking player with the highest ARO is paired against the black‐seeking player with the lowest rating;
-
- The Burstein System,
The players in a score group are sorted according to their Sonneborn‐Berger points (then Buchholz, then Median) and then the top ranked player is paired against the last ranked player, the second ranked player against the last but one, and so on, with floaters coming from the middle.
It was used to pair teams in the Olympiad before 2006;
-
- The Olympiad Pairing System used in Olympiad since 2006
This system is similar to the Lim system for individual tournaments with only small amendments (reduced requirements for colour preference and floating) for team pairings.
An example of a cross table of the final ranking of a Swiss Tournament:
|
Final ranking |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
| Rank | SNo. |
|
Name | IRtg | FED | 1.Rd. | 2.Rd. | 3.Rd. | 4.Rd. | 5.Rd. | 6.Rd. | 7.Rd. | 8.Rd. | 9.Rd. | Pts | Res. | BH. | BH. | BL | Vict | Rtg+/- | Ra | Rp | ||||||||||||||||||
| 1 | 6 | IM | J. Thybo | 2466 | DEN | 30 | b | 1 | 6 | w | 0 | 54 | b | 1 | 18 | w | 1 | 8 | b | 1 | 24 | w | 1 | 4 | b | ½ | 7 | b | ½ | 13 | w | 1 | 7 | 0 | 46½ | 50½ | 5 | 6 | 13,6 | 2371 | 2591 |
| 2 | 9 | IM | J. Plenca | 2440 | CRO | 33 | w | 1 | 37 | b | ½ | 27 | w | ½ | 59 | b | 1 | 15 | w | ½ | 25 | b | ½ | 53 | w | 1 | 16 | b | 1 | 7 | w | 1 | 7 | 0 | 40½ | 44 | 4 | 5 | 13,0 | 2336 | 2556 |
| 3 | 2 | GM | B. Deac | 2559 | ROU | 11 | b | ½ | 38 | w | 1 | 22 | b | 1 | 16 | b | 1 | 9 | w | ½ | 12 | b | 1 | 7 | w | ½ | 5 | w | ½ | 4 | b | ½ | 6½ | 0 | 48 | 52½ | 5 | 4 | 1,2 | 2387 | 2551 |
| 4 | 7 | IM | L. Livaic | 2461 | CRO | 66 | w | 1 | 32 | b | 1 | 21 | w | 1 | 14 | b | ½ | 7 | w | 0 | 29 | b | 1 | 1 | w | ½ | 24 | b | 1 | 3 | w | ½ | 6½ | 0 | 45 | 48½ | 4 | 5 | 14,4 | 2415 | 2581 |
| 5 | 3 | IM | M. Santos Ruiz | 2505 | ESP | 46 | w | 1 | 24 | b | ½ | 17 | w | ½ | 37 | b | 1 | 10 | w | 1 | 16 | b | ½ | 14 | w | ½ | 3 | b | ½ | 20 | w | 1 | 6½ | 0 | 44 | 48½ | 4 | 4 | 3,2 | 2357 | 2523 |
| 6 | 33 |
|
J. Radovic | 2330 | SRB | 68 | w | 1 | 1 | b | 1 | 14 | w | 0 | 53 | b | 1 | 29 | w | ½ | 26 | b | ½ | 12 | w | 1 | 10 | b | ½ | 15 | w | 1 | 6½ | 0 | 44 | 47½ | 4 | 5 | 30,1 | 2377 | 2543 |
| 7 | 1 | IM | H. Martirosyan | 2570 | ARM | 25 | w | 0 | 60 | b | 1 | 46 | w | 1 | 48 | b | 1 | 4 | b | 1 | 9 | w | 1 | 3 | b | ½ | 1 | w | ½ | 2 | b | 0 | 6 | 0 | 47 | 51 | 5 | 5 | -5,1 | 2390 | 2510 |
| 8 | 39 |
|
I. Akhvlediani | 2303 | GEO | 77 | w | 1 | 12 | b | 1 | 9 | w | ½ | 15 | b | ½ | 1 | w | 0 | 23 | b | ½ | 48 | w | 1 | 31 | b | 1 | 10 | w | ½ | 6 | 0 | 45½ | 48 | 4 | 4 | 25,3 | 2343 | 2464 |
| 9 | 8 | IM | N. Morozov | 2461 | MDA | 62 | b | 1 | 56 | w | 1 | 8 | b | ½ | 20 | w | 1 | 3 | b | ½ | 7 | b | 0 | 15 | w | ½ | 18 | w | ½ | 25 | b | 1 | 6 | 0 | 44 | 48 | 5 | 4 | 5,9 | 2385 | 2510 |
| 10 | 17 | FM | B. Haldorsen | 2397 | NOR | 57 | w | 1 | 55 | b | ½ | 25 | w | ½ | 27 | b | 1 | 5 | b | 0 | 38 | w | 1 | 11 | b | 1 | 6 | w | ½ | 8 | b | ½ | 6 | 0 | 43½ | 47½ | 5 | 4 | 4,1 | 2307 | 2432 |
| 11 | 45 | FM | M. Askerov | 2281 | RUS | 3 | w | ½ | 17 | b | 0 | 73 | w | 1 | 43 | b | 1 | 21 | w | 1 | 19 | b | ½ | 10 | w | 0 | 53 | b | 1 | 26 | w | 1 | 6 | 0 | 42½ | 45½ | 4 | 5 | 32,2 | 2373 | 2498 |
| 12 | 12 | FM | I. Janik | 2418 | POL | 79 | b | 1 | 8 | w | 0 | 40 | b | 1 | 41 | w | 1 | 28 | b | 1 | 3 | w | 0 | 6 | b | 0 | 54 | w | 1 | 24 | w | 1 | 6 | 0 | 42 | 44½ | 4 | 6 | 3,9 | 2326 | 2451 |
| 13 | 11 | IM | M. Costachi | 2418 | ROU | 72 | w | ½ | 40 | b | ½ | 66 | w | 1 | 21 | b | ½ | 37 | w | 1 | 15 | b | ½ | 17 | w | 1 | 14 | b | 1 | 1 | b | 0 | 6 | 0 | 41½ | 44½ | 5 | 4 | 6,8 | 2350 | 2475 |
| 14 | 4 | FM | A. Sorokin | 2486 | RUS | 67 | b | 1 | 44 | w | 1 | 6 | b | 1 | 4 | w | ½ | 24 | b | 0 | 20 | w | 1 | 5 | b | ½ | 13 | w | 0 | 17 | b | ½ | 5½ | 0 | 46 | 49½ | 5 | 4 | -3,2 | 2375 | 2455 |
| 15 | 18 | S. Tica | 2389 | CRO | 64 | b | 1 | 54 | w | 1 | 28 | b | ½ | 8 | w | ½ | 2 | b | ½ | 13 | w | ½ | 9 | b | ½ | 39 | w | 1 | 6 | b | 0 | 5½ | 0 | 45 | 49 | 5 | 3 | 1,7 | 2318 | 2398 | |
| 16 | 63 |
|
K. Yayloyan | 2142 | ARM | 53 | w | 1 | 59 | b | 1 | 41 | b | 1 | 3 | w | 0 | 19 | b | 1 | 5 | w | ½ | 24 | b | ½ | 2 | w | 0 | 18 | b | ½ | 5½ | 0 | 44½ | 48 | 5 | 4 | 61,6 | 2410 | 2492 |
| 17 | 32 | FM | D. Tokranovs | 2334 | LAT | 49 | b | ½ | 11 | w | 1 | 5 | b | ½ | 19 | w | 0 | 79 | b | 1 | 28 | w | 1 | 13 | b | 0 | 38 | w | 1 | 14 | w | ½ | 5½ | 0 | 43½ | 46 | 4 | 4 | 10,1 | 2321 | 2401 |
| 18 | 23 | FM | J. Haug | 2379 | NOR | 60 | w | ½ | 45 | b | ½ | 39 | w | 1 | 1 | b | 0 | 66 | w | 1 | 33 | b | 1 | 26 | w | ½ | 9 | b | ½ | 16 | w | ½ | 5½ | 0 | 41½ | 45 | 4 | 3 | -0,9 | 2283 | 2363 |
| 19 | 15 | FM | M. Warmerdam | 2399 | NED | 39 | w | ½ | 72 | b | ½ | 23 | w | 1 | 17 | b | 1 | 16 | w | 0 | 11 | w | ½ | 56 | b | 1 | 25 | w | ½ | 22 | b | ½ | 5½ | 0 | 41½ | 44½ | 4 | 3 | -4,8 | 2275 | 2355 |
| 20 | 21 | IM | A. Sousa | 2386 | POR | 63 | w | 1 | 25 | b | ½ | 55 | w | 1 | 9 | b | 0 | 57 | w | 1 | 14 | b | 0 | 27 | w | 1 | 34 | w | 1 | 5 | b | 0 | 5½ | 0 | 41 | 45 | 4 | 5 | 6,2 | 2351 | 2431 |
| 21 | 30 | FM | R. Lagunow | 2357 | GER | 75 | b | 1 | 76 | w | 1 | 4 | b | 0 | 13 | w | ½ | 11 | b | 0 | 30 | w | ½ | 62 | b | 1 | 28 | w | ½ | 39 | b | 1 | 5½ | 0 | 39½ | 42 | 5 | 4 | -2,2 | 2247 | 2327 |
| 22 | 28 | IM | A. Perez Garcia | 2361 | ESP | 78 | b | 1 | 58 | w | ½ | 3 | w | 0 | 57 | b | 0 | 63 | w | 1 | 45 | b | 1 | 23 | w | 1 | 26 | b | ½ | 19 | w | ½ | 5½ | 0 | 39 | 42 | 4 | 4 | -1,1 | 2263 | 2343 |
| 23 | 56 | FM | M. Jogstad | 2259 | SWE | 31 | b | 0 | 80 | w | 1 | 19 | b | 0 | 76 | w | 1 | 35 | b | 1 | 8 | w | ½ | 22 | b | 0 | 47 | w | 1 | 42 | b | 1 | 5½ | 0 | 38½ | 40½ | 5 | 5 | 15,1 | 2262 | 2329 |
| 24 | 36 |
|
G. Kouskoutis | 2314 | GRE | 70 | b | 1 | 5 | w | ½ | 34 | b | 1 | 26 | b | 1 | 14 | w | 1 | 1 | b | 0 | 16 | w | ½ | 4 | w | 0 | 12 | b | 0 | 5 | 0 | 47 | 50½ | 5 | 4 | 14,7 | 2368 | 2409 |
| 25 | 44 | FM | T. Lazov | 2289 | MKD | 7 | b | 1 | 20 | w | ½ | 10 | b | ½ | 42 | w | ½ | 44 | b | 1 | 2 | w | ½ | 31 | w | ½ | 19 | b | ½ | 9 | w | 0 | 5 | 0 | 45½ | 50 | 4 | 2 | 25,7 | 2423 | 2466 |
| 26 | 10 | FM | J. Vykouk | 2440 | CZE | 38 | b | ½ | 49 | w | 1 | 47 | b | 1 | 24 | w | 0 | 56 | b | 1 | 6 | w | ½ | 18 | b | ½ | 22 | w | ½ | 11 | b | 0 | 5 | 0 | 42 | 46 | 5 | 3 | -12,2 | 2287 | 2330 |
| 27 | 40 | FM | I. Lopez Mulet | 2302 | ESP | 80 | b | 1 | 31 | w | ½ | 2 | b | ½ | 10 | w | 0 | 36 | b | 1 | 42 | w | ½ | 20 | b | 0 | 68 | w | 1 | 34 | b | ½ | 5 | 0 | 41½ | 43½ | 5 | 3 | 6,3 | 2294 | 2319 |
| 28 | 57 | FM | V. Sevgi | 2240 | TUR | 50 | w | 1 | 43 | b | 1 | 15 | w | ½ | 29 | b | ½ | 12 | w | 0 | 17 | b | 0 | 46 | w | 1 | 21 | b | ½ | 32 | w | ½ | 5 | 0 | 41 | 45½ | 4 | 3 | 26,6 | 2362 | 2405 |
| 29 | 16 | FM | R. Haria | 2398 | ENG | 36 | b | 1 | 47 | w | ½ | 58 | b | 1 | 28 | w | ½ | 6 | b | ½ | 4 | w | 0 | 39 | b | 0 | 40 | w | ½ | 54 | b | 1 | 5 | 0 | 40½ | 4½ | 5 | 3 | -9,4 | 2276 | 2319 |
| 30 | 49 | FM | C. Meunier | 2270 | FRA | 1 | w | 0 | 68 | b | 1 | 50 | w | 1 | 31 | b | 0 | 48 | w | ½ | 21 | b | ½ | 41 | b | 1 | 42 | w | ½35 | b | ½ | 5 | 0 | 40½ | 44 | 5 | 3 | 18,9 | 2349 | 2392 | |
| 31 | 13 |
|
S. Drygalov | 2415 | RUS | 23 | w | 1 | 27 | b | ½ | 37 | w | 0 | 30 | w | 1 | 55 | b | ½ | 62 | w | 1 | 25 | b | ½ | 8 | w | 0 | 33 | b | ½ | 5 | 0 | 40 | 44 | 4 | 3 | -10,8 | 2285 | 2328 |
| 32 | 37 |
|
K. Nowak | 2314 | POL | 86 | - | + | 4 | w | 0 | 81 | b | ½ | 36 | w | ½ | 39 | b | ½ | 58 | w | 1 | 42 | b | ½ | 35 | w | ½ | 28 | b | ½ | 5 | 0 | 38½ | 40 | 4 | 2 | -8,4 | 2244 | 2192 |
| 33 | 52 | FM | K. Karayev | 2266 | AZE | 2 | b | 0 | 74 | w | ½ | 49 | b | ½ | 75 | w | 1 | 41 | b | 1 | 18 | w | 0 | 47 | b | ½ | 56 | w | 1 | 31 | w | ½ | 5 | 0 | 37½ | 40 | 4 | 3 | 4,8 | 2244 | 2287 |
| 34 | 5 | IM | V. Dragnev | 2483 | AUT | 40 | w | ½ | 61 | b | 1 | 24 | w | 0 | 55 | b | 0 | 72 | w | 1 | 37 | b | 1 | 44 | w | 1 | 20 | b | 0 | 27 | w | ½ | 5 | 0 | 37 | 40 | 4 | 4 | -17,0 | 2287 | 2330 |
| 35 | 29 |
|
V. Lukiyanchuk | 2358 | UKR | 61 | w | 0 | 52 | b | 1 | 57 | w | ½ | 45 | b | ½ | 23 | w | 0 | 60 | b | 1 | 66 | w | 1 | 32 | b | ½ | 30 | w | ½ | 5 | 0 | 36 | 39½ | 4 | 3 | -14,7 | 2197 | 2240 |
| 36 | 59 | FM | C. Patrascu | 2227 | ROU | 29 | w | 0 | 83 | b | 1 | 43 | w | ½ | 32 | b | ½ | 27 | w | 0 | 59 | b | 1 | 50 | w | ½ | 44 | b | ½ | 53 | w | 1 | 5 | 0 | 35½ | 37 | 4 | 3 | 20,1 | 2302 | 2329 |
| 37 | 38 | FM | K. Koziol | 2313 | POL | 74 | b | 1 | 2 | w | ½ | 31 | b | 1 | 5 | w | 0 | 13 | b | 0 | 34 | w | 0 | 58 | b | ½ | 62 | w | 1 | 41 | b | ½ | 4½ | 0 | 42 | 44½ | 5 | 3 | 3,0 | 2326 | 2321 |
| 38 | 53 |
|
M. Friedland | 2264 | ISR | 26 | w | ½ | 3 | b | 0 | 69 | w | 1 | 51 | w | 1 | 42 | b | ½ | 10 | b | 0 | 55 | w | 1 | 17 | b | 0 | 43 | w | ½ | 4½ | 0 | 40½ | 44 | 4 | 3 | 14,2 | 2357 | 2357 |
| 39 | 58 |
|
J. Thorgeirsson | 2232 | ISL | 19 | b | ½ | 42 | w | ½ | 18 | b | 0 | 82 | w | 1 | 32 | w | ½ | 51 | b | 1 | 29 | w | 1 | 15 | b | 0 | 21 | w | 0 | 4½ | 0 | 40½ | 42 | 4 | 3 | 14,1 | 2314 | 2285 |
| 40 | 48 | FM | S. Tifferet | 2273 | ISR | 34 | b | ½ | 13 | w | ½ | 12 | w | 0 | 73 | b | 1 | 53 | w | 0 | 61 | b | 1 | 43 | w | ½ | 29 | b | ½ | 48 | w | ½ | 4½ | 0 | 39 | 42 | 4 | 2 | 10,3 | 2340 | 2340 |
- Scheveningen System
The Scheveningen system is mainly used for teams. In such a team competition, each player of one team meets each player of the opposing team. The number of rounds therefore is equal to the number of players in a team. Scheveningen system, the players of first half of one team meet all players of the first half of the opposing team and players of the second half of one team play against players of the second half of the other team. Example: Team A and B have eight players each. A1, A2, A3 and A4 play versus B1, B2, B3 and B4. At the same time A5, A6, A7 and A8 play versus B5, B6, B7 and B8. Finally four rounds are necessary
In a Semi‐
Standard Tables
Match on 2 Boards
Round 1 A1-B1 A2-B2
Round 2 B2-A1 B1-A2
Match on 3 Boards
Round 1 A1-B1 A2-B2 B3-A3
Round 2 B2-A1 A2-B3 B1-A3
Round 3 A1-B3 B1-A2 A3-B2
Match on 4 Boards
Round 1 A1-B1 A2-B2 B3-A3 B4-A4
Round 2 B2-A1 B1-A2 A3-B4 A4-B3
Round 3 A1-B3 A2-B4 B1-A3 B2-A4
Round 4 B4-A1 B3-A2 A3-B2 A4-B1
Match on 5 Boards
Round 1 A1-B1 A2-B2 A3-B3 B4-A4 B5-A5
Round 2 B2-A1 B3-A2 B4-A3 A4-B5 A5-B1
Round 3 A1-B3 A2-B4 B5-A3 B1-A4 A5-B2
Round 4 B4-A1 B5-A2 A3-B1 A4-B2 B3-A5
Round 5 A1-B5 B1-A2 B2-A3 A4-B3 A5-B4
Match on 6 Boards
Round 1 B1-A1 B5-A2 A3-B4 A4-B2 A5-B3 B6-A6
Round 2 B2-A1 A2-B1 B3-A3 B4-A4 A5-B6 A6-B5
Round 3 A1-B3 A2-B2 B1-A3 B6-A4 B5-A5 A6-B4
Round 4 A1-B4 B6-A2 A3-B5 A4-B1 B2-A5 B3-A6
Round 5 B5-A1 B4-A2 A3-B6 B3-A4 A5-B1 A6-B2
Round 6 A1-B6 A2-B3 B2-A3 A4-B5 B4-A5 B1-A6
Match on 7 Boards
Round 1 A1-B1 A2-B2 A3-B3 A4-B4 B5-A5 B6-A6 B7-A7
Round 2 B2-A1 B3-A2 B4-A3 A4-B5 A5-B6 A6-B7 B1-A7
Round 3 A1-B3 A2-B4 A3-B5 B6-A4 B7-A5 B1-A6 A7-B2
Round 4 B4-A1 B5-A2 A3-B6 A4-B7 A5-B1 B2-A6 B3-A7
Round 5 A1-B5 A2-B6 B7-A3 B1-A4 B2-A5 A6-B3 A7-B4
Round 6 B6-A1 A2-B7 A3-B1 A4-B2 B3-A5 B4-A6 B5-A7
Round 7 A1-B7 B1-A2 B2-A3 B3-A4 A5-B4 A6-B5 A7-B6
Match on 8 Boards
Round 1 A1-B1 A2-B2 A3-B3 A4-B4 B5-A5 B6-A6 B7-A7 B8-A8
Round 2 B2-A1 B3-A2 B4-A3 B1-A4 A5-B6 A6-B7 A7-B8 A8-B5
Round 3 A1-B3 A2-B4 A3-B1 A4-B2 B7-A5 B8-A6 B5-A7 B6-A8
Round 4 B4-A1 B1-A2 B2-A3 B3-A4 A5-B8 A6-B5 A7-B6 A8-B7
Round 5 A1-B5 A2-B6 A3-B7 A4-B8 B1-A5 B2-A6 B3-A7 B4-A8
Round 6 B6-A1 B7-A2 B8-A3 B5-A4 A5-B2 A6-B3 A7-B4 A8-B1
Round 7 A1-B7 A2-B8 A3-B5 A4-B6 B3-A5 B4-A6 B1-A7 B2-A8
Round 8 B8-A1 B5-A2 B6-A3 B7-A4 A5-B4 A6-B1 A7-B2 A8-B3
Match on 9 Boards
Round 1 A1-B1 A2-B2 A3-B3 A4-B4 A5-B5 B6-A6 B7-A7 B8-A8 B9-A9
Round 2 B2-A1 B3-A2 B4-A3 B5-A4 A5-B6 A6-B7 A7-B8 A8-B9 B1-A9
Round 3 A1-B3 A2-B4 A3-B5 A4-B6 B7-A5 B8-A6 B9-A7 B1-A8 A9-B2
Round 4 B4-A1 B5-A2 B6-A3 A4-B7 A5-B8 A6-B9 A7-B1 B2-A8 B3-A9
Round 5 A1-B5 A2-B6 A3-B7 B8-A4 B9-A5 B1-A6 B2-A7 A8-B3 A9-B4
Round 6 B6-A1 B7-A2 A3-B8 A4-B9 A5-B1 A6-B2 B3-A7 B4-A8 B5-A9
Round 7 A1-B7 A2-B8 B9-A3 B1-A4 B2-A5 B3-A6 A7-B4 A8-B5 A9-B6
Round 8 B8-A1 A2-B9 A3-B1 A4-B2 A5-B3 B4-A6 B5-A7 B6-A8 B7-A9
Round 9 A1-B9 B1-A2 B2-A3 B3-A4 B4-A5 A6-B5 A7-B6 A8-B7 A9-B8
Match on 10 Boards
Round 1 A1-B1 A2-B2 A3-B8 B9-A4 B5-A5 A6-B3 A7-B4 B6-A8 B7-A9 B10-A10
Round 2 B2-A1 B1-A2 B4-A3 A4-B7 A5-B10 B8-A6 B3-A7 A8-B5 A9-B6 A10-B9
Round 3 A1-B3 A2-B8 A3-B1 B2-A4 B6-A5 A6-B4 A7-B10 B7-A8 B9-A9 B5-A10
Round 4 B4-A1 B3-A2 A3-B9 B1-A4 A5-B7 B10-A6 A7-B6 B8-A8 A9-B5 A10-B2
Round 5 A1-B5 A2-B4 B2-A3 A4-B3 B1-A5 B9-A6 B7-A7 A8-B10 B8-A9 A10-B6
Round 6 B6-A1 A2-B7 B5-A3 B4-A4 A5-B8 A6-B1 A7-B9 A8-B2 B10-A9 B3-A10
Round 7 A1-B7 B5-A2 A3-B10 A4-B6 B4-A5 B2-A6 B1-A7 B9-A8 A9-B3 A10-B8
Round 8 B8-A1 B6-A2 B3-A3 B10-A4 A5-B9 A6-B5 A7-B2 A8-B1 A9-B4 B7-A10
Round 9 A1-B9 A2-B10 A3-B6 A4-B8 B2-A5 A6-B7 B5-A7 B3-A8 B1-A9 B4-A10
Round 10 B10-A1 B9-A2 B7-A3 A4-B5 A5-B3 B6-A6 B8-A7 A8-B4 A9-B2 A10-B1
- Skalitzka System
When using a Round Robin system for three teams it is necessary to organize three rounds and in each round one team is without an opponent. Skalitzka system gives a possibility to find a ranking for three teams by playing only two rounds and to avoid that a team has no opponent. Each team has to be composed of an even number of players, all of them ranked in a fixed board order. Before the pairing is made one team is marked by capital letters, then second one by small letters and the third one by figures. Then the pairings are:
| round 1 | round 2 |
| A ‐ a | 1 ‐ A |
| b ‐ 1 | a ‐ 2 |
| 2 ‐ B | B ‐ b |
| C ‐ c | 3 ‐ C |
| d ‐ 3 | c ‐ 4 |
| 4 ‐ D | D ‐ d |
| E ‐ e | 5 ‐ E |
| f ‐ 5 | e ‐ 6 |
| 6 ‐ F | F ‐ f |
- Other systems.
-
- Matches
Most matches between two players are played over a restricted number of games. Matches may be rated by FIDE if they are registered in advance with FIDE and if both players are rated before the match. After one player has won the match all subsequent games are not rated.
-
- Knock -out
The main advantage of a knock‐out system is to create a big final match. The whole schedule is known in advance. Mostly a knock‐out match consists of two games. As it is necessary to have a clear winner of each round another day for the tie‐break games has to be foreseen. Such tie‐ break games usually are organized with two rapid games followed by two or four blitz games. If still the tie is unbroken, one final “sudden death match” shall be played. The playing time should be 5 minutes for White and 4 minutes for Black, or a similar playing time. White has to win the game, for Black a draw is sufficient to win the match. See chapter “Tie‐break Systems”.
RESTRICTED DRAWING OF LOTS
Approved by the 1987 General Assembly
Introduction:
In certain cases, regulations state that the drawing of lots should be carried out in such a way that players of the same federation do not meet in the last three rounds, if possible.
This may be done by using the Varma tables, reproduced below, which can be modified for tournaments of from 10 to 24 players.
Directions for "restricted" drawing of tournament numbers
-
-
- In the case of 19 or 20 participants, the players of the same group (A, B, C or
-
D) as indicated below, will not meet in the last three rounds: (6, 7, 8, 9, 15, 16, 17, 18)
(1, 2, 3, 11, 12, 13, 14)
(5, 10, 19)
(4, 20)
The arbiter shall prepare beforehand, unmarked envelopes each containing one of the above numbers. The envelopes containing a group of numbers are then placed in unmarked larger envelopes.
-
-
- The order in which players draw lots is listed beforehand as follows: The players of the federation with the most number of representatives shall draw first. Where two or more federations have the same number of representatives, precedence is determined by the alphabetical order of the FIDE country code. Among players of the same federation, precedence is determined by the alphabetical order of their names.
- For example, the first player of the first contingent with the largest number of players shall choose one of the large envelopes containing at least enough numbers for his contingent, and then draw one of the numbers from this envelope. The other players from the same contingent shall also draw their numbers from the same envelope. The numbers that remain are available for use by other players.
- The players of the next contingent then draw lots and the procedure is followed until all players have drawn their numbers.
- The following Varma Tables can be used for 10 to 20 players. 9/10 players
-
1. (3, 4, 8);
2. (5, 7, 9);
3. (1, 6);
4. (2, 10)
11/12 players
1. (4, 5, 9, 10);
2. (2, 6, 7);
3. (1, 8, 12);
4. (3, 11)
13/14 players
1. (4, 5, 6, 11, 12);
2. (1, 2, 8, 9);
3. (7, 10, 13);
4. (3, 14)
15/16 players
1. (5, 6, 7, 12, 13, 14);
2. (1, 2, 3, 9, 10);
3. (8, 11, 15);
4. (4, 16)
17/18 players
1. (5, 6, 7, 8, 14, 15, 16);
2. (1, 2, 3, 10, 11, 12);
3. (9, 13, 17);
4. (4, 18)
19/20 players
1. (6, 7, 8, 9, 15, 16, 17, 18);
2. (1, 2, 3, 11, 12, 13, 14);
3. (5, 10, 19);
4. 4, 20)
21/22 players
1. (6, 7, 8, 9, 10, 17, 18, 19, 20);
2. (1, 2, 3, 4, 12, 13, 14, 15);
3. (11, 16, 21);
4. (5, 22)
23/24 players
1. (6, 7, 8, 9, 10, 11, 19, 20, 21, 22);
2. (1, 2, 3, 4, 13, 14, 15, 16, 17);
3. (12, 18, 23);
4. (5, 24)
FIDE SWISS RULES (04)
Basic rules for Swiss Systems (C.04.01)
The following rules are valid for each Swiss system unless explicitly stated otherwise.
- The number of rounds to be played is declared beforehand.
- Two players shall not play against each other more than once.
- Should the number of players to be paired be odd, one player is unpaired. This player receives a pairing-allocated bye: no opponent, no colour and as many points as are rewarded for a win, unless the rules of the tournament state otherwise.
- A player who has already received a pairing-allocated bye, or has already scored a (forfeit) win due to an opponent not appearing in time, shall not receive the pairing-allocated bye.
- In general, players are paired to others with the same score.
- For each player the difference between the number of black and the number of white games shall not be greater than 2 or less than –2.
Each system may have exceptions to this rule in the last round of a tournament.
- No player shall receive the same colour three times in a row.
Each system may have exceptions to this rule in the last round of a tournament.
- 1. In general, a player is given the colour with which he played less games.
2. If colours are already balanced, then, in general, the player is given the colour that alternates from the last one with which he played.
i. The pairing rules must be such transparent that the person who is in charge for the pairing can explain them.
General handling rules for Swiss Tournaments (C.04.2)
- Pairing Systems
- The pairing system used for a FIDE rated Swiss tournament should be one of the published FIDE Swiss Systems.
Accelerated methods are acceptable if they were announced in advance by the organizer and are published in section C.04.5.
- In derogation of the previous rule, unpublished pairing systems or accelerated methods may be permitted, provided that a detailed written description of their rules:
- be submitted in advance to the Qualification Commission (QC) and temporarily authorized by them; and
- be explicitly presented to the participants before the start of the tournament.
- While reporting a tournament to FIDE, the Arbiter shall declare which official FIDE Swiss system and acceleration method (if any) were used, or provide the temporary authorization(s) given by the QC as per the previous rule.
- The Swiss Pairing Systems defined by FIDE and not deprecated (see C.04.4) pair the players in an objective, impartial and reproducible way.
In any tournament where such systems are used, different arbiters, or different endorsed software programs, must be able to arrive at identical pairings.
- It is not allowed to alter the correct pairings in favour of any player.
Where it can be shown that modifications of the original pairings were made to help a player achieve a norm or a direct title, a report may be submitted to the QC to initiate disciplinary measures through the Ethics Commission.
- Initial Order
- Before the start of the tournament, a measure of the player’s strength is assigned to each player. The strength is usually represented by rating lists of the players. If one rating list is available for all participating players, then this rating list should be used.
It is advisable to check all ratings supplied by players. If no reliable rating is known for a player, the arbiters should make an estimation of it as accurately as possible.
- Before the first round the players are ranked in order of, respectively
- Strength (rating)
- FIDE-title (GM-IM- WGM-FM-WIM-CM-WFM-WCM-no title)
- alphabetically (unless it has been previously stated that this criterion has been replaced by another one)
- This ranking is used to determine the pairing numbers; the highest one gets #1 etc. If, for any reason, the data used to determine the rankings were not correct, they can be adjusted at any time. The pairing numbers may be reassigned accordingly to the corrections.
No modification of a pairing number is allowed after the fourth round has been paired.
- Late Entries
- According to FIDE Tournament Rules, any prospective participant who has not arrived at the venue of a FIDE competition before the time scheduled for the drawing of lots shall be excluded from the tournament unless he shows up at the venue in time before a pairing of another round.
An exception may be made in the case of a registered participant who has given written notice in advance that he will be unavoidably late.
- Where the Chief Arbiter decides to admit a latecomer,
- if the player's notified time of arrival is in time for the start of the first round, the player is given a pairing number and paired in the usual way.
-
- if the player's notified time of arrival is in time only for the start of the second (or third) round ("Late Entry"), then the player is not paired for the rounds which he cannot play. Instead, he receives no points for unplayed rounds (unless the rules of the tournament say otherwise), and is given an appropriate pairing number and paired only when he actually arrives.
- If there are late entries, the Pairing Numbers that were given at the start of the tournament are considered provisional. The definitive Pairing Numbers are given only when the List of Participants is closed, and corrections made accordingly in the results charts.
- Pairing, colour and publishing rules
-
- Adjourned games are considered draws for pairing purposes only.
- A player who is absent without notifying the arbiter will be considered as withdrawn, unless the absence is explained with acceptable arguments before the next pairing is published.
- Players who withdraw from the tournament will no longer be paired.
- Players known in advance not to play in a particular round are not paired in that round and score zero (unless the rules of the tournament say otherwise).
- Only played games count in situations where the colour sequence is meaningful. So, for instance, a player with a colour history of BWB=W (i.e. no valid game in round-4) will be treated as if his colour history was =BWBW. WB=WB will count as =WBWB, BWW=B=W as ==BWWBW and so on.
-
- Two paired players, who did not play their game, may be paired together in a future round.
- The results of a round shall be published at the usual place of communication at announced time due to the schedule of the tournament.
- If either
- a result was written down incorrectly, or
- a game was played with the wrong colours, or
- a player's rating has to be corrected (and playing numbers possibly recomputed as in C.04.2.C.3),
and a player communicates this to the arbiter within a given deadline after publication of results, the new information shall be used for the standings and the pairings of the next round. The deadline shall be fixed in advance according to the timetable of the tournament.
If the error notification is made after the pairing but before the end of the next round, it will affect the next pairing to be done.
If the error notification is made after the end of the next round, the correction will be made after the tournament for submission to rating evaluation only.
-
- After a pairing is complete, sort the pairs before publishing them.
The sorting criteria are (with descending priority)
-
-
- the score of the higher ranked player of the involved pair;
- the sum of the scores of both players of the involved pair;
- the rank according to the Initial Order (C.04.2.B) of the higher ranked player of the involved pair.
- Once published, the pairings shall not be changed unless they are found to violate C.04.1.b (Two players shall not play against each other more than once).
-
FIDE (Dutch) System (C.04.3)
Version approved at the 87th FIDE Congress in Baku 2016
A Introductory Remarks and Definitions
-
- Initial ranking list
See C.04.2.B (General Handling Rules - Initial order)
-
- Order
For pairings purposes only, the players are ranked in order of, respectively
-
-
- score
- pairing numbers assigned to the players accordingly to the initial ranking list and subsequent modifications depending on possible late entries or rating adjustments
-
-
- Scoregroups and pairing brackets
A scoregroup is normally composed of (all) the players with the same score. The only exception is the special "collapsed" scoregroup defined in A.9.
A (pairing) bracket is a group of players to be paired. It is composed of players coming from one same scoregroup (called resident players) and of players who remained unpaired after the pairing of the previous bracket.
A (pairing) bracket is homogeneous if all the players have the same score; otherwise it is heterogeneous.
A remainder (pairing bracket) is a sub-bracket of a heterogeneous bracket, containing some of its resident players (see B.3 for further details).
-
- Floaters and floats
-
-
- A downfloater is a player who remains unpaired in a bracket, and is thus moved to the next bracket. In the destination bracket, such players are called "moved- down players" (MDPs for short).
- After two players with different scores have played each other in a round, the higher ranked player receives a downfloat, the lower one an upfloat.
-
A player who, for whatever reason, does not play in a round, also receives a downfloat.
-
- Byes
See C.04.1.c (Should the number of players to be paired be odd, one player is unpaired. This player receives a pairing-allocated bye: no opponent, no colour and as many points as are rewarded for a win, unless the regulations of the tournament state otherwise).
-
- Colour differences and colour preferences
The colour difference of a player is the number of games played with white minus the number of games played with black by this player.
The colour preference is the colour that a player should ideally receive for the next game. It can be determined for each player who has played at least one game.
-
-
- An absolute colour preference occurs when a player’s colour difference is greater than +1 or less than -1, or when a player had the same colour in the two latest rounds he played. The preference is white when the colour difference is less than -1 or when the last two games were played with black. The preference is black when the colour difference is greater than +1, or when the last two games were played with white.
- A strong colour preference occurs when a player‘s colour difference is +1 (preference for black) or -1 (preference for white).
- A mild colour preference occurs when a player’s colour difference is zero, the preference being to alternate the colour with respect to the previous game he played.
- Players who did not play any games have no colour preference (the preference of their opponents is granted).
-
-
- Topscorers
Topscorers are players who have a score of over 50% of the maximum possible score when pairing the final round of the tournament.
-
- Pairing Score Difference (PSD)
The pairing of a bracket is composed of pairs and downfloaters.
Its Pairing Score Difference is a list of score-differences (SD, see below), sorted from the highest to the lowest.
For each pair in a pairing, the SD is defined as the absolute value of the difference between the scores of the two players who constitute the pair.
For each downfloater, the SD is defined as the difference between the score of the downfloater, and an artificial value that is one point less than the score of the lowest ranked player of the current bracket (even when this yields a negative value).
Note: The artificial value defined above was chosen in order to be strictly less than the lowest score of the bracket, and generic enough to work with different scoring-point systems and in presence of non-existent, empty or sparsely populated brackets that may follow the current one.
PSD(s) are compared lexicographically (i.e. their respective SD(s) are compared one by one from first to last - in the first corresponding SD(s) that are different, the smallest one defines the lower PSD).
-
- Round-Pairing Outlook
The pairing of a round (called round-pairing) is complete if all the players (except at most one, who receives the pairing-allocated bye) have been paired and the absolute criteria C1-C3 have been complied with.
If it is impossible to complete a round-pairing, the arbiter shall decide what to do. Otherwise, the pairing process starts with the top scoregroup, and continues bracket by bracket until all the scoregroups, in descending order, have been used and the round- pairing is complete.
However, if, during this process, the downfloaters (possibly none) produced by the bracket just paired, together with all the remaining players, do not allow the completion of the round-pairing, a different processing route is followed. The last paired bracket is called Penultimate Pairing Bracket (PPB). The score of its resident players is called the "collapsing" score. All the players with a score lower than the collapsing score constitute the special "collapsed" scoregroup mentioned in A.3.
The pairing process resumes with the re-pairing of the PPB. Its downfloaters, together with the players of the collapsed scoregroup, constitute the Collapsed Last Bracket (CLB), the pairing of which will complete the round-pairing.
Note: Independently from the route followed, the assignment of the pairing-allocated bye (see C.2) is part of the pairing of the last bracket.
Section B describes the pairing process of a single bracket.
Section C describes all the criteria that the pairing of a bracket has to satisfy.
Section E describes the colour allocation rules that determine which players will play with white.
B Pairing Process for a bracke
-
- Parameters definitions
- M0 is the number of MDP(s) coming from the previous bracket. It may be zero.
- MaxPairs is the maximum number of pairs that can be produced in the bracket under consideration (see C.5).
- Parameters definitions
Note: MaxPairs is usually equal to the number of players divided by two and rounded downwards. However, if, for instance, M0 is greater than the number of resident players, MaxPairs is at most equal to the number of resident players.
-
-
- M1 is the maximum number of MDP(s) that can be paired in the bracket (see C.6).
-
Note: M1 is usually equal to the number of MDPs coming from the previous bracket, which may be zero. However, if, for instance, M0 is greater than the number of resident players, M1 is at most equal to the number of resident players.
Of course, M1 can never be greater than MaxPairs.
-
- Subgroups (original composition)
To make the pairing, each bracket will be usually divided into two subgroups, called S1 and S2.
S1 initially contains the highest N1 players (sorted according to A.2), where N1 is either M1 (in a heterogeneous bracket) or MaxPairs (otherwise).
S2 initially contains all the remaining resident players.
When M1 is less than M0, some MDPs are not included in S1. The excluded MDPs (in number of M0 - M1), who are neither in S1 nor in S2, are said to be in a Limbo.
Note: the players in the Limbo cannot be paired in the bracket, and are thus bound to double-float.
-
- Preparation of the candidate
S1 players are tentatively paired with S2 players, the first one from S1 with the first one from S2, the second one from S1 with the second one from S2 and so on.
In a homogeneous bracket: the pairs formed as explained above and all the players who remain unpaired (bound to be downfloaters) constitute a candidate (pairing).
In a heterogeneous bracket: the pairs formed as explained above match M1 MDPs from S1 with M1 resident players from S2. This is called a MDP-Pairing. The remaining resident players (if any) give rise to the remainder (see A.3), which is then paired with the same rules used for a homogeneous bracket.
Note: M1 may sometimes be zero. In this case, S1 will be empty and the MDP(s) will all be in the Limbo. Hence, the pairing of the heterogeneous bracket will proceed directly to the remainder.
A candidate (pairing) for a heterogeneous bracket is composed by a MDP-Pairing and a candidate for the ensuing remainder. All players in the Limbo are bound to be downfloaters.
-
- Evaluation of the candidate
If the candidate built as shown in B.3 complies with all the absolute and completion criteria (from C.1 to C.4), and all the quality criteria from C.5 to C.19 are fulfilled, the candidate is called "perfect" and is (immediately) accepted. Otherwise, apply B.5 in order to find a perfect candidate; or, if no such candidate exists, apply B.8.
-
- Actions when the candidate is not perfect
The composition of S1, Limbo and S2 has to be altered in such a way that a different candidate can be produced.
The articles B.6 (for homogeneous brackets and remainders) and B.7 (for heterogeneous brackets) define the precise sequence in which the alterations must be applied.
After each alteration, a new candidate shall be built (see B.3) and evaluated (see B.4).
-
- Alterations in homogeneous brackets or remainders
Alter the order of the players in S2 with a transposition (see D.1). If no more transpositions of S2 are available for the current S1, alter the original S1 and S2 (see B.2) applying an exchange of resident players between S1 and S2 (see D.2) and reordering the newly formed S1 and S2 according to A.2.
-
- Alterations in heterogeneous brackets
Operate on the remainder with the same rules used for homogeneous brackets (see B.6).
Note: The original subgroups of the remainder, which will be used throughout all the remainder pairing process, are the ones formed right after the MDP-Pairing. They are called S1R and S2R (to avoid any
confusion with the subgroups S1 and S2 of the complete heterogeneous bracket).
If no more transpositions and exchanges are available for S1R and S2R, alter the order of the players in S2 with a transposition (see D.1), forming a new MDP-Pairing and possibly a new remainder (to be processed as written above).
If no more transpositions are available for the current S1, alter, if possible (i.e. if there is a Limbo), the original S1 and Limbo (see B.2), applying an exchange of MDPs between S1 and the Limbo (see D.3), reordering the newly formed S1 according to A.2 and restoring S2 to its original composition.
-
- Actions when no perfect candidate exists
Choose the best available candidate. In order to do so, consider that a candidate is better than another if it better satisfies a quality criterion (C5-C19) of higher priority; or, all quality criteria being equally satisfied, it is generated earlier than the other one in the sequence of the candidates (see B.6 or B.7).
C Pairing Criteria
Absolute Criteria
No pairing shall violate the following absolute criteria:
-
- see C.04.1.b (Two players shall not play against each other more than once)
- see C.04.1.d (A player who has already received a pairing-allocated bye, or has already scored a (forfeit) win due to an opponent not appearing in time, shall not receive the pairing-allocated bye).
- non-topscorers (see A.7) with the same absolute colour preference (see A6.a) shall not meet (see C.04.1.f and C.04.1.g).
Completion Criterion
-
- if the current bracket is the PPB (see A.9): choose the set of downfloaters in order to complete the round-pairing.
Quality Criteria
To obtain the best possible pairing for a bracket, comply as much as possible with the following criteria, given in descending priority:
-
- maximize the number of pairs (equivalent to: minimize the number of downfloaters).
-
- minimize the PSD (This basically means: maximize the number of paired MDP(s); and, as far as possible, pair the ones with the highest scores).
- if the current bracket is neither the PPB nor the CLB (see A.9): choose the set of downfloaters in order first to maximize the number of pairs and then to minimize the PSD (see C.5 and C.6) in the following bracket (just in the following bracket).
- minimize the number of topscorers or topscorers' opponents who get a colour difference higher than +2 or lower than -2.
- minimize the number of topscorers or topscorers' opponents who get the same colour three times in a row.
- minimize the number of players who do not get their colour preference.
- minimize the number of players who do not get their strong colour preference.
- minimize the number of players who receive the same downfloat as the previous round.
- minimize the number of players who receive the same upfloat as the previous round.
- minimize the number of players who receive the same downfloat as two rounds before.
- minimize the number of players who receive the same upfloat as two rounds before.
- minimize the score differences of players who receive the same downfloat as the previous round.
- minimize the score differences of players who receive the same upfloat as the previous round.
- minimize the score differences of players who receive the same downfloat as two rounds before.
- minimize the score differences of players who receive the same upfloat as two rounds before.
D Rules for the sequential generation of the pairings
Before any transposition or exchange take place, all players in the bracket shall be tagged with consecutive in-bracket sequence-numbers (BSN for short) representing their respective ranking order (according to A.2) in the bracket (i.e. 1, 2, 3, 4,...).
-
- Transpositions in S2
A transposition is a change in the order of the BSNs (all representing resident players) in S2.
All the possible transpositions are sorted depending on the lexicographic value of their first N1 BSN(s), where N1 is the number of BSN(s) in S1 (the remaining BSN(s) of S2 are ignored in this context, because they represent players bound to constitute the remainder in case of a heterogeneous bracket; or bound to downfloat in case of a homogeneous bracket - e.g. in a 11-player homogeneous bracket, it is 6-7-8-9-10, 6-7- 8-9-11, 6-7-8-10-11,..., 6-11-10-9-8, 7-6-8-9-10,..., 11-10-9-8-7 (720 transpositions); if
the bracket is heterogeneous with two MDPs, it is: 3-4, 3-5, 3-6,..., 3-11, 4-3, 4-5,...,
11-10 (72 transpositions)).
-
- Exchanges in homogeneous brackets or remainders (original S1 ↔ original S2)
An exchange in a homogeneous brackets (also called a resident-exchange) is a swap of two equally sized groups of BSN(s) (all representing resident players) between the original S1 and the original S2.
In order to sort all the possible resident-exchanges, apply the following comparison rules between two resident-exchanges in the specified order (i.e. if a rule does not discriminate between two exchanges, move to the next one).
The priority goes to the exchange having:
-
-
- the smallest number of exchanged BSN(s) (e.g exchanging just one BSN is better than exchanging two of them).
- the smallest difference between the sum of the BSN(s) moved from the original S2 to S1 and the sum of the BSN(s) moved from the original S1 to S2 (e.g. in a bracket containing eleven players, exchanging 6 with 4 is better than exchanging 8 with 5; similarly exchanging 8+6 with 4+3 is better than exchanging 9+8 with 5+4; and so on).
- the highest different BSN among those moved from the original S1 to S2 (e.g. moving 5 from S1 to S2 is better than moving 4; similarly, 5-2 is better than 4- 3; 5-4-1 is better than 5-3-2; and so on).
- the lowest different BSN among those moved from the original S2 to S1 (e.g. moving 6 from S2 to S1 is better than moving 7; similarly, 6-9 is better than 7- 8; 6-7-10 is better than 6-8-9; and so on).
-
-
- Exchanges in heterogeneous brackets (original S1 ↔ original Limbo)
An exchange in a heterogeneous bracket (also called a MDP-exchange) is a swap of two equally sized groups of BSN(s) (all representing MDP(s)) between the original S1 and the original Limbo.
In order to sort all the possible MDP-exchanges, apply the following comparison rules between two MDP-exchanges in the specified order (i.e. if a rule does not discriminate between two exchanges, move to the next one) to the players that are in the new S1 after the exchange.
The priority goes to the exchange that yields a S1 having:
-
-
- the highest different score among the players represented by their BSN (this comes automatically in complying with the C.6 criterion, which says to minimize the PSD of a bracket).
- the lowest lexicographic value of the BSN(s) (sorted in ascending order).
-
Any time a sorting has been established, any application of the corresponding D.1, D.2 or D.3 rule, will pick the next element in the sorting order.
E Colour Allocation rules
Initial-colour
It is the colour determined by drawing of lots before the pairing of the first round. For each pair apply (with descending priority):
-
- Grant both colour preferences.
- Grant the stronger colour preference. If both are absolute (topscorers, see A.7) grant the wider colour difference (see A.6).
- Taking into account C.04.2.D.5, alternate the colours to the most recent time in which one player had white and the other black.
- Grant the colour preference of the higher ranked player.
- If the higher ranked player has an odd pairing number, give him the initial-colour; otherwise give him the opposite colour.
Note: Always consider sections C.04.2.B/C (Initial Order/Late Entries) for the proper management of the pairing numbers.
Other FIDE-approved Pairing Systems
Use of these systems is deprecated unless for a system there is a FIDE endorsed program (see, in Appendix C.04.A, the Annex-3 "List of FIDE Endorsed Programs") with a free pairing-checker (see A.5 in the same appendix) able to verify tournaments run with this system.
FIDE-approved Accelerated Systems
In Swiss tournaments with a wide range of (mostly reliable) playing strengths, the results of the first round(s) are usually quite predictable. In the first round, only a few percent of the games have a result other than "win to the stronger part". The same may happen again in round two. It can be shown that, in title tournaments, this can prevent players from achieving norms.
An accelerated pairing is a variation of Swiss pairings in which the first rounds are modified in such a way as to overcome the aforementioned weaknesses of the Swiss system, without compromising the reliability of the final rankings.
It is not appropriate to design an entirely new pairing system for acceleration, but rather design a system that works together with existing FIDE-defined pairing systems. This result is normally achieved by rearranging score brackets in some way that is not only dependent on the points that the players have scored. For instance, one of the possible methods is to add so-called "virtual points" to the score of some higher rated players (who are supposedly stronger) and henceforth build the score brackets based on the total score (real score + virtual points).
The following chapters will describe the methods that were statistically proven to accomplish the aforementioned goals. The Baku Acceleration Method is presented first, because it was the first that, through statistical analysis, was proven to be good and stable (and is also easy to explain).
Other accelerated methods may be added, as long as they can be proven, through statistical analysis, to get better results than already described methods or, if their effectiveness is comparable, to be simpler.
Unless explicitly specified otherwise, each described acceleration method is applicable to any Swiss Pairing System.
Baku Acceleration
-
-
- Premise
-
In its current presentation, the Baku Acceleration Method is applicable for tournaments that last nine rounds or more, and in which the standard scoring point system (one point for a win, half point for a draw) is used.
-
-
- Initial Groups Division
-
Before the first round, the list of players to be paired (properly sorted) shall be split in two groups, GA and GB.
The first group (GA) shall contain the first half of the players, rounded up to the nearest even number. The second group (GB) shall contain all the remaining players.
Note: for instance, if there are 161 players in the tournament, the nearest even number that comprises the first half of the players (i.e. 80.5) is 82. The formula 2 * Q (2 times Q), where Q is the number of players
divided by 4 and rounded upwards, may be helpful in computing such number - that, besides being the number of GA-players, is also the pairing number of the last GA-player.
-
-
- Late entries
-
If there are entries after the first round, those players shall be accommodated in the pairing list according to C.04.2.B/C (Initial Order/Late Entries).
The last GA-player shall be the same as in the previous round.
Note 1: In such circumstances, the pairing number of the last GA-player may be different by the one set accordingly to Rule 2.
Note 2: After the first round, GA may contain an odd number of players.
-
-
- Virtual points
-
Before pairing the first three rounds, all the players in GA are assigned a number of points (called virtual points) equal to 1.
Such virtual points are reduced to 0.5 before pairing the fourth and the fifth round.
Note: Consequently, no virtual points are given to players in GB or to any player after the fifth round has been played.
-
-
- Pairing score
-
The pairing score of a player (i.e. the value used to define the scoregroups and internally sort them) is given by the sum of his standings points and the virtual points assigned to him.
Computer Swiss Pairing Programs endorsed by FIDE:
Some examples of FIDE (Dutch) Pairings
with Baku 2016 Swiss Rules
In the Baku 2016 FIDE Congress, the new Rules for the FIDE (Dutch) Swiss system were approved. The Rules were thoroughly rewritten, and are now far easier to understand and use. It is now time to begin to put them into practice – let us therefore peer together into some examples of pairings – we will start with a very simple one and proceed to situations that are a bit more difficult. Before reading the examples, however, it is strongly advisable to carefully read the new Rules, which can be found (together with more interesting material about pairings) in the Systems of Pairing and Programs Commission (SPP) webpage, http://pairings.fide.com.
All the examples here come from one same tournament, whose crosstable is this:
ID NAME Rtg Pts | 1 2 3 4 5 6 7 8 9
------------------------------------------------------------------------------
1 Alice 2600 6.5 | +W7 +B9 =W4 +B5 =W12 =W2 -B3 +B10 +W6
2 Bob 2550 6.0 | =B8 -W12 +W11 +B6 +W4 =B1 =B5 +W9 =B3
3 Charline 2500 5.0 | -W9 -B11 +W10 -B12 +W6 +B8 +W1 =B4 =W2
4 David 2450 6.0 | +B10 =W5 =B1 +W8 -B2 +W9 +B12 =W3 =B7
5 Eleanor 2400 4.5 | +W11 =B4 +W12 -W1 -B9 =B7 =W2 =B8 =W10
6 Frank 2350 4.0 | =B12 =W8 =B9 -W2 -B3 +B10 =W7 +W11 -B1
7 Gale 2300 4.0 | -B1 +W10 =B8 =W9 -B11 =W5 =B6 =W12 =W4
8 Hans 2250 4.0 | =W2 =B6 =W7 -B4 -W10 -W3 +B11 =W5 +B12
9 Isabelle 2200 4.0 | +B3 -W1 =W6 =B7 +W5 -B4 =W10 -B2 =B11
10 Jack 2150 3.0 | -W4 -B7 -B3 +W11 +B8 -W6 =B9 -W1 =B5
11 Karima 2100 3.0 | -B5 +W3 -B2 -B10 +W7 =B12 -W8 -B6 =W9
12 Leonard 2050 4.0 | =W6 +B2 -B5 +W3 =B1 =W11 -W4 =B7 -W8
------------------------------------------------------------------------------
Example 1 – The very basics
In the second round, the first scoregroup is:
Player Score Col. hist. Opp. hist.
| 1 | 1,0 | B | 7 |
| 4 | 1,0 | W | 10 |
| 5 | 1,0 | B | 11 |
| 9 | 1,0 | W | 3 |
To make the pairing, we must first determine the colour preferences of the players and divide the bracket into subgroups: S1=[1W, 4B] and S2=[5W, 9B] (B,W are strong colour preferences). Now we pair the first player of S1 to the first of S2, the second to the second, and so on, as usual, obtaining the candidate pairing 1-5, 4-9, which we must now evaluate against all the pairing criteria.
This candidate is legal (which means that it complies with all the relevant absolute criteria), and therefore we do not discard it at once; but it is nonetheless a definitely poor pairing, as we are disregarding two colour preferences, while we could – and therefore should – disregard zero. In the pairing jargon, we say that the candidate has a failure value equal to two for criterion C.10 (which tells us to minimise the number of disregarded colour preferences). Hence, we temporarily store the candidate (it might still be used, should we find nothing better), but proceed to look for a worthier one.
To look for a better pairing, the first alteration to try is always a transposition in S2; in this bracket, there is only one possible (the rules to generate transpositions, as well as exchanges, are in Section D of the FIDE Dutch rules), which lends S2=[9B, 5W]. Now, the candidate pairing is 1-9, 5-4 and, evaluating it against the pairing criteria, we promptly realize that it complies with them all – it is therefore a perfect candidate, which we immediately chose, thus terminating the pairing process for the bracket.
To complete it, however, yet another step is required: we must check that the rest of the players can actually be paired – this is informally called the “Requirement Zero”, and the test is a “completion test”. A failure in this test would mean that the pairing of the current bracket does not allow the pairing for the whole round to come to fruition. In such a case, we discard the candidate and change the pairing rules – but we do not need to worry about this just now: in early rounds, every participant may be legally paired with almost everyone else, so the probability of such an event is virtually zero – and, in fact, the completion test is passed. We will go back to the matter later on.
Example 2 – A complaining floater
In the fourth round, player #11 got the second downfloat running… we want to show him that his pairing is correct! Here is the pre-pairing situation:
Player Score Col. hist. Float hist. Opp. hist.
…
| 12 | 1,5 | WBB | 6 2 5 | |
| 3 | 1,0 | WBW | 9 11 10 | |
| 11 | 1,0 | BWB | ↓ | 5 3 2 |
| 10 | 0,0 | WBB | 4 7 3 |
Player #12 here is a “Moved-down player” (MDP) – that is, a player that downfloated from the previous bracket – so this bracket is heterogeneous. From now on, we will also need the float history of the players (which we only seldom need in early rounds). Here, we can see that player #11 just downfloated. By the way, this bracket shall of course give a downfloater, because the number of players is odd.
The pairing of heterogeneous brackets is built in two phases. In the first phase, we compose the MDP-Pairing, which is a partial pairing containing the MDP(s) (or, at least, as many of them as possible). In the second phase, we complete the candidate with the pairs containing only resident players (viz., those players that belong to the scoregroup); and then, only after the candidate is complete, we evaluate it as a whole.
The first phase is actually very simple: we start as usual by preparing the subgroups, annotating the colour preferences and the float status for each player: S1=[12W*], S2=[3B, 11W↓] (B*, W* are absolute colour preferences), then pair it in the usual way. The only difference is that in subgroup S1 we will put not half the players, as we would do in a homogeneous bracket, but all the MDPs that we are going to pair.
The first possible MDP-Pairing contains the pair 12-3. The remaining players (here, we have just one!) constitute the remainder {11W↓}, which we are going to pair in the second phase. With three players, we can build just one pair; hence, the remainder will yield no pairs at all and the candidate (which is built putting together the pairs from the
MDP-Pairing, the pairs from the pairing of the remainder and the possible downfloaters) is 12-3, 11 to float.
We must now evaluate this candidate – which means that we must verify its compliance with each of the relevant paring criteria. We readily verify that this candidate complies with all the pairing criteria but C.12 (Minimize the number of players who receive the same downfloat as the previous round). Hence, the candidate is legal but not perfect – let us call it the “temporary-best” (or “champ”). We therefore store it and proceed to look for something better – but, if nothing better is available, we will retrieve it and use it as “the” pairing.
To get the next candidate, we apply a transposition (there is only one), obtaining S2=[11W↓, 3B], which yields the candidate 12-11, 3 to float. This candidate is legal and therefore we evaluate it – but only to learn that it does not comply with criterion
C.10 (Minimize the number of players who do not get their colour preference). We must now compare the current temporary-best with this new candidate: the latter fails on C.10, while the former fails on C.12. Since C.10 is more important than C.12, we discard the new candidate and keep the old temporary-best.
Now there are no more transpositions, but we must continue to look for a perfect candidate – or use up all the possible ones. The next step would be to try an exchange between S1 and the Limbo (which means, to try and change the MDPs to be paired), but there are no more MDPs.
The last attempt is to reduce the number of MDPs to pair – this means, in practice, to make player #12 join the Limbo, from which it cannot help but float again, and pair players 11-3 between themselves. In a homogeneous bracket, a similar exchange would have been possible – but here player #12 is a MDP! This means that its score is higher than that of the residents – making it float causes a worse PSD, and thus a failure on criterion C.6 (Minimize the PSD), which is more important than both C.10 and C.12. Thus, also this candidate is discarded.
Well, we have now exhausted all the possible pairings, but found no perfect candidates: hence, we must choose the “less imperfect” one, which is of course the current temporary-best – and contains player #11 as a floater.
Example 3
The third example is rather typical, and still it is not a difficult one – but it definitely requires some patience. In round four, the two-point scoregroup contains only one player, who shall downfloat to the next bracket:
Player Score Col. hist. Float hist. Opp. hist.
| 4 | 2,0 | BWB | ↑ | 10 5 1 |
| 2 | 1,5 | BWW | ↑ | 8 12 11 |
| 6 | 1,5 | BWB | 12 8 9 | |
| 7 | 1,5 | BWB | 1 10 8 | |
| 8 | 1,5 | WBW | 2 6 7 | |
| 9 | 1,5 | BWW |
|
3 1 6 |
| 12 | 1,5 | WBB | 6 2 5 | |
| 3 | 1,0 | WBW | 9 11 10 | |
| 11 | 1,0 | BWB | ↓ | 5 3 2 |
Player #4 is a MDP. Player #2 just upfloated (by the way, the same holds true for player #4 – but we may ignore it, because that player is certainly not going to upfloat now), and we foresee a downfloater (again, because the number of players is odd). Four players expect White and three expect Black, so we should be able to form three pairs, with no disregarded colour preferences (x=0).
As always, we first compose the MDP-Pairing, then we complete the candidate with the pairs containing only resident players, and finally we evaluate it.
First, we prepare the subgroups, annotating the colour preferences and the float status for each player: S1=[4W], S2=[2B*↑, 6W, 7W, 8B, 9B*, 12W*]. The first possible MDP-Pairing contains the pair 4-2. The remaining players constitute the remainder
{6W, 7W, 8B, 9B*, 12W*}, which we are going to pair in the second phase. Once again, we divide the players (of the remainder) in two subgroups: S1R=[6W, 7W], S2R=[8B, 9B*, 12W*], thus obtaining the remaining pairs of the candidate as 6-8, 7- 9, 12 to float. Now we must evaluate the candidate, which is not legal, because players #6 and #8 already played each other in a previous round.
We discard the candidate at once, and proceed with a transposition in the remainder – that is, in the subgroup S2R. We might observe, however, that player #6 already met all the players in S2R, and therefore no transposition at all can yield a legal pairing. We may therefore be “smart” and jump ahead to try a resident exchange, viz. an exchange of players between S1R and S2R.
The first such exchange is between players #7 (the lower in S1R) and #8 (the higher in S2R), and gives the new subgroups S1R=[6W, 8B] and S2R=[7W, 9B*, 12W*]. This gives a candidate 4-2, 6-7, 8-9, 12 to float which is legal, so that we may at least evaluate it. The first thing to verify is that the chosen downfloater (#12) can be paired in the next bracket (criterion C.7), and we find no problems here. Two colour preferences are disregarded, so we have a double failure for criterion C.10. Moreover, player #2 upfloats for the second time running, so we have one failure for criterion C.13 too. In conclusion, the candidate is legal but not perfect – as usual, we store it as temporary-best and proceed to look for something better.
To get the next candidate, we apply a transposition, obtaining S2R=[7W, 12W*, 9B*]. The resulting candidate (4-2, 6-7, 12-8, 9 to float) still has one failure for C.10 and one for C.13 – however, it is better than the previous one, so we store this one and discard the latter, but we keep looking for a perfect one.
Any other transposition yields illegal candidates (let’s remember that 6-9 and 6-12 are forbidden), so we should now try the following resident exchange, which gives S1R=[6W, 9B*], S2R=[7W, 8B, 12W*]. The only legal candidate that we did not already try is (after a transposition) 4-2, 6-7, 12-9, 8 to float – but it is easy to verify that this is not better than the current champ, so we discard it and keep the latter.
We should then proceed examining all exchanges and transpositions, one by one, but we might already suspect that this can be long and tedious work… luckily, we may avoid some useless effort and save some precious time by looking into the remainder
{6W, 7W, 8B, 9B*, 12W*} in a “smarter” way. The key lies in player #6: since it has already played with #8, #9, and #12, the only legal candidates containing #6 must have the pair 6-7 in them, which gives a failure for C.10. However, if we make #6 downfloat, we may build the pairs 7-9, 12-8, thus disregarding no colour preferences at all (please note that 7-8, 12-9 would give an illegal candidate, because #7 and #8 already met eachother). This yields the candidate 4-2, 7-9, 12-8, 6 to float, which contains only one failure for criterion C.13, and therefore becomes the new temporary-best.
This is the best we may do with the current MDP-Pairing; yet, it is not a perfect candidate, so our quest is not over… The next step is looking for a better MDP-Pairing; let us therefore start again with the original subgroups: S1=[4W], S2=[2B*↑, 6W, 7W, 8B, 9B*, 12W*].
We might observe that we have one C.13 failure because #4 has been paired with #2, who was an upfloater in the previous round. To get a better pairing, we need to pair player #4 with someone else, and this requires one or more transpositions in S2 – which, in practice, means to try to pair #4 with each member of S2 in turn. However, the pairings 4-6 and 4-7, although legal, introduce (at least) one failure for C.10 – therefore, no candidate originating from those MDP-Pairings can be better than the current temporary-best, and there is no point in examining them. The first potentially interesting pairing is 4-8, which is legal and yields the remainder {2B*↑, 6W, 7W, 9B*, 12W*}.
We are now interested only in (legal) candidates better than the temporary-best (if any)
– that is, with perfect colour matching – and this is only possible after exchanging #6 for #7: S1R=[2B*↑, 7W], S2R=[6W, 9B*, 12W*]. This yields the candidate 4-8, 6-2, 7-9, 12 to float. Its evaluation shows that all criteria are complied with (in particular, the downfloater optimises the pairing in the next bracket) and therefore the candidate is, at long last, perfect! We immediately choose it (discarding the previous temporary- best, now useless) and proceed to perform the completion test for Requirement Zero – which is (luckily) successful.
Example 4
In this last example taken from round 5 (after that round, the pairings become quite challenging), player #7 got a “double-downfloat” – let us see why. Actually, this pairing involves the last three scoregroups, and is a bit (but not very much) more difficult than the previous ones.
Player Score Col. hist. Float hist. Opp. hist.
| 5 | 2,5 | WBWW | 11 4 12 1 | |
| 7 | 2,0 | BWBW | 1 10 8 9 | |
| 9 | 2,0 | BWWB |
|
3 1 6 7 |
| 6 | 1,5 | BWBW |
|
12 8 9 2 |
| 8 | 1,5 | WBWB | ↑ | 2 6 7 4 |
| 3 | 1,0 | WBWB | ↑ | 9 11 10 12 |
| 10 | 1,0 | WBBW | ↑ | 4 7 3 11 |
| 11 | 1,0 | BWBB | ↓↓ | 5 3 2 10 |
The first bracket is heterogeneous, with player #5 as a MDP: [5B*][7b, 9w] (w, b= mild colour preferences). This bracket is easily paired: after a transposition, player #5, who has an absolute preference for Black, is paired with the only White-seeker, which is #9, while player #7 downfloats. In the destination bracket, player #7 is compatible, so criterion C.7 is satisfied – actually, all the relevant criteria are complied with, so the candidate is perfect.
However, before proceeding to the next scoregroup, we must prove that the remaining players, together with the floater, allow at least one legal pairing (in early rounds we may usually omit this check, as it is practically always satisfied – but not now!). It is important to appreciate that, in performing this test, we are not looking for “the pairing”. All we want is to show that at least one pairing exists for the remaining players and the quality of this pairing is not important in the least: its mere existence is proof that the round-pairing can be completed, and therefore we will not need to go back to this bracket again. Actually, for example we might pair 7-11, 6-10, 8-3.
Now we proceed to the next bracket, which is [7b][6b, 8w↑]. It is easy to proof that this bracket gives no perfect candidate; in the end, we settle for the (final) temporary-best: 6-7, 8 to float. However, here comes the twist: the remaining players, who are now {8, 3, 10, 11}, cannot be paired, and therefore we have a Requirement Zero failure.
We must restart from the current bracket [7b][6b, 8w↑], which becomes the Penultimate Pairing Bracket (PPB). This bracket is now subject to a new and special rule: it must provide the downfloaters needed to pair the rest of the players. All those remaining players are put together in a big melting pot, which is called the Special Collapsed Scoregroup (SCS). This special scoregroup may therefore contain players with different scores (and indeed this is usually the case, although this does not happen here) and, together with the downfloaters from the PPB, forms the Collapsed Last Bracket (CLB).
Actually, players #3, #10, and #11 are all incompatible with each other – and therefore, to complete the pairing, we apparently need three MDPs, one for each of them. Well, the PPB contains just three players – hence, we obtain zero pairs from the PPB (which is, sometimes, a perfectly legitimate pairing) and send the three required floaters in the CLB.
We must now pair the CLB. It contains players with different scores, so, in general, we must pay attention to the Pairing Score Difference (PSD). Because of this, in the bracket we want to annotate also the scores of the players: {(2.0)7b, (1.5)6b, (1.5)8w↑, (1.0)3w↑, (1.0)10b↑, (1.0)11W*↓↓}.
For this bracket, we must find the best possible pairing. This may seem a tough task, but actually most possible candidates are illegal, because many players already played with each other (of course, this is more or less usual in a CLB). The legal pairings are therefore comparatively few, and usually the best strategy is simply to evaluate them all and apply the “Sieve pairing method”. In the present case, player #7 has already played with #8 and #10. Moreover, it cannot be paired with #6 because the rest would not be paired. Hence, we have only four legal candidates complying with criteria C.1-
C.5. The first criterion to check is now C.6 to minimise the PSD (see C.04.3.A.8 in the FIDE Swiss Rules):
7-3, 6-10, 8-11 PSD={1.0, 0.5, 0.5}
7-3, 6-11, 8-10 PSD={1.0, 0.5, 0.5}
7-11, 6-10, 8-3 PSD={1.0, 0.5, 0.5}
7-11, 6-3, 8-10 PSD={1.0, 0.5, 0.5}
Actually, all the candidates have the same PSD – and of course, this is no coincidence: since the SCS contained no different scores, all pairings with its resident must end up with the same score differences. Now, criteria C.7, C.8 and C.9 do not apply, so we proceed to C.10 to check for colour matching.
1. 7b-3w, 6b-10b, 8w-11W*
2. 7b-3w, 6b-11W*, 8w-10b
3. 7b-11W*, 6b-10b, 8w-3w
4. 7b-11W*, 6b-3w, 8w-10b
The first and third candidates contain each two disregarded colour preferences, so we discard them and keep the second and fourth, which are perfectly colour matched.
1. 7b-3w↑, 6b-11W*, 8w↑-10b↑
2. 7b-11W*, 6b-3w↑, 8w↑-10b↑
We have no strong colour preferences, so criterion C.11 does not apply. Since we have no prospective downfloaters, also criterion C.12 does not apply. We proceed to criterion C.13, regarding the repetition of upfloats: the first candidate fails once on pair 3-7, whilst the second one fails once on pair 3-6. As the failure values are equal, the candidates are still “tied” and we must proceed. We skip criteria C.14, C15, and C.16 (they are not relevant) and reach criterion C.17 (Minimize the score differences of players who receive the same upfloat as the previous round). Here, at last, we have a difference: in the first candidate, the score difference of the player who receives the second upfloat in a row, which is #3, is 1.0; in the second candidate, the score difference of the player who receives the second upfloat in a row, which incidentally is again #3, is 0.5. Hence, the failure value for this criterion is greater for the first candidate. The latter is therefore discarded too, and we finally remain with the only surviving candidate, which is also the required pairing: 11-7, 3-6, 8-10.
Conclusion
I hope that these examples may be of help in studying the new FIDE (Dutch) Swiss Rules. I strongly suggest to all those interested in this matter to pay a visit the FIDE Systems of Pairings and Programs Commission (SPP) webpage (http://pairings.fide.com).
Tournament development with the FIDE (Dutch) System
An example of a six-round tournament (With Baku 2016 FIDE C.04 Swiss Rules) By Mario Held (Member of FIDE Systems of Pairings and Programs Commission)
PART ONE – THE RULES
ANNOTATED RULES FOR THE FIDE (DUTCH) SWISS SYSTEM
Hereafter, we present the general rules for Swiss Systems (FIDE Handbook C.04.1 and C.04.2) and the Rules for the FIDE (Dutch) System (FIDE Handbook C.04.3), together with some notes to explain them.
The first part contains rules that define the technical requirements any Swiss pairing system must obey, whilst the second part targets a set of various aspects relating to the handling of tournaments, from the fairness of the systems to the management of late entrants, and several rules that are common to all the FIDE approved systems.
The third part contains the Rules for the FIDE (Dutch) Swiss System, which in its turn is comprised of the following sections:
- Introductory Remarks and Definitions: containing the basic concepts about the system and its control variables; namely, the last paragraph (A.9) is an essential description of the pairing process that will be described and regulated in detail by section (B).
- Pairing process for a bracket: this section explains how to build a candidate pairing, determine its quality (checking it against the pairing criteria), and, when necessary, improve the quality of the pairing (by looking for better candidates).
- Pairing Criteria: defining limitations to the possible pairings of the players. Some of those limitations are common to all Swiss pairing systems, while others are specific to the FIDE (Dutch) system and give origin to some of its peculiarities.
- Rules for the sequential generation of the pairings: this section defines the transposition and exchange procedures, showing how to “stir” the players list when natural pairing is not possible (because two players have already played against each other, or because of colours incompatibility, and so on)
- Colour Allocation Rules: after the completion of the pairing, each player receives its colour according to these rules.
With reference to previous versions, the FIDE (Dutch) rules have been almost completely reworded, in order to make them simpler and more intuitive. The algorithm, which used to occupy the whole section C, has now been completely evicted from the rules, together with the whole old section B. Instead of the latter, a new section C contains a revised list of the pairing quality criteria, which is both more detailed and clearer than the previous one.
For all this rewording, the real changes in the pairings address only a few cases, while a vast majority of the pairings remain just the same as they were with the previous rules 1.
We would like to suggest you to carefully study the Rules until you feel you master their principles and meanings, before starting to study the tournament example.
- Readers may find detailed information about those changes in the documents relating Abu Dhabi 2015 and Baku 2016 FIDE Congresses, in the FIDE Swiss Pairings Program Commission website http://www.pairings.fide.com.
-
- FIDE SWISS RULES
- Basic rules for Swiss Systems
- FIDE SWISS RULES
The following rules are valid for each Swiss system unless explicitly stated otherwise.
- The number of rounds to be played is declared beforehand.
- Two players shall not play each other more than once.
- Should the number of players to be paired be odd, one player is unpaired. This player receives a pairing-allocated bye: no opponent, no colour and as many points as are rewarded for a win, unless the rules of the tournament state otherwise.
- A player who has already received a pairing- allocated bye, or has already scored a (forfeit) win due to an opponent not appearing in time, shall not receive the pairing-allocated bye.
- In general, players are paired to others with the same score.
After the start of the tournament, we are not allowed to change the number of rounds (however, this may become inevitable by force of circumstances).
This is the only principle of Swiss Systems we cannot dispense with (unless doing differently is absolutely inevitable...)!
Please note that this rule allows event organizers to establish a different value for byes (e.g. half a point) instead of the usual whole point.
However, and whatever its value is, a pairing allocated bye (“PAB”) cannot be assigned to any player who has already received a previous one, or a forfeit win. The allocation of a PAB, though, is not prevented by a previous bye “on request”1 (when such a provision is permitted by the tournament rules).
The location of this principle before colour balancing rules highlights its greater importance with respect to the latter. It is because of this rule that we cannot make players float to suit colour
1 In the previous versions of the Swiss Rules, any number of points got without playing, like e.g. a requested “Half Point Bye”, did prevent the allocation of a PAB.
- For each player the difference of the number of black and the number of white games shall not be greater than 2 or less than –2.
Each system may have exceptions to this rule in the last round of a tournament.
- No player will receive the same colour three times in a row.
Each system may have exceptions to this rule in the last round of a tournament.
- 1. In general, a player is given the colour with which he played less games.
- If colours are already balanced, then, in general, the player is given the colour that alternates from the last one with which he played.
- The pairing rules must be such transparent that the person who is in charge for the pairing can explain them.
preferences that are not absolute (see C.04.3:A.6.a).
We should emphasize that the exceptions to rules f and g for the last round are possible, but not compulsory. The FIDE (Dutch) system adopts them, tough in practice only when there are very good reasons to do so. Other systems do not do the same - e.g., the Dubov Swiss System definitely refuses to make such exceptions, which seem not to be consistent with the basic principles of that system.
This rule warrants the good colour balancing typical of all FIDE approved Swiss Systems.
Sometimes, players ask the Arbiter to justify, or explain, the pairings, which, nowadays, are most usually prepared with the help of a software program (which should be a FIDE endorsed one, if only possible). However, we want to remember that, even if the pairings are made by means of a computer, it is always the arbiter who takes responsibility for the pairing, not the software.
-
-
- General handling rules for Swiss Tournaments
-
- Pairing Systems
- The pairing system used for a FIDE rated tournament shall be either one of the published FIDE Swiss Systems or a detailed written description of the rules shall be explicitly presented to the participants.
- While reporting a tournament to FIDE, the Arbiter shall declare which of the official FIDE Swiss systems was used. If another system was used, the Arbiter shall submit the rules of this system for checking by the Systems of Pairings and Programs Commission (SPPC).
- Accelerated methods are acceptable if they were announced in advance by the organizer and are not biased in favour of any player.
- The FIDE Swiss Rules pair the players in an objective and impartial way, and different arbiters or software programs following the
All the rules in this section tend to the same aim: to prevent any possible tampering with the pairings in favour of one or more participants (such as helping a player to obtain a norm). To this effect, the pairing rules must be well specified, transparent, and unambiguous in the first place.
pairing rules should arrive at identical pairings.
- It is not allowed to alter the correct pairings in favour of any player.
Where it can be shown that modifications of the original pairings were made to help a player achieve a norm or a direct title, a report may be submitted to the Qualification Commission to initiate disciplinary measures through the Ethics Commission.
- Initial Order
- Before the start of the tournament a measure of the player’s strength is assigned to each player. The strength is usually represented by rating lists of the players. If one rating list is available for all participating players, then this rating list should be used.
- It is advisable to check all ratings supplied by players. If no reliable rating is known for a player the arbiters should make an estimation of it as accurately as possible.
- Before the first round the players are ranked in order of, respectively:
- Strength (rating)
- FIDE title (GM - IM - WGM - FM -WIM
- CM - WFM - WCM - no title)
-
- alphabetically (unless it has been previously stated that this criterion has been replaced by another one)
- This ranking is used to determine the pairing numbers; the highest one gets #1 etc.
If, for any reason, the data used to determine the rankings were not correct, they can be adjusted at any time. The pairing numbers may
The fundamental principle of all Swiss systems is to pair tied players (i.e. players with the same number of points) so that, in the top echelon, the number of ties is halved at every round. Thus, in a tournament with T rounds, if the number N of players is less than 2T [i.e. T ≥ log2 (N)], we should (theoretically) have no ties for the first place.
However, practice shows that, to reach this goal in a real environment (which includes draws and unexpected results), a precise evaluation of the strength of players is essential.
When no better information is available, the estimated rating of an unknown player can be determined based on a national rating (if available) using the appropriate conversion formulas; or other rating lists, tranches, tournament results and so on may be used, if reliable. In conclusion, the Arbiter shall have to use sound judgment and reasoning, to obtain the best possible evaluation with what data is available.
FIDE titles are ordered by descending nominal rating; when ratings are equal, titles obtained through norms take precedence with respect to automatic ones.
Alphabetical sorting is unessential, its only rationale being that of ensuring an unambiguous order. Thus, this criterion can be substituted for by any other sorting method capable of giving an unambiguous order, provided this method has been previously declared in the tournament regulations.
Please notice that a lower numeric value corresponds to a higher ranking; this choice may not seem “natural”, but it is deeply rooted in common language by now.
Pairing numbers are used by all Swiss pairing systems except Dubov. Thus, a change in be reassigned accordingly to the corrections, but only for the first three rounds. No modification of a pairing number is allowed after the fourth round.
- Late Entries
- According to FIDE Tournament Rules, any prospective participant who has not arrived at the venue of a FIDE competition before the time scheduled for the drawing of lots shall be excluded from the tournament unless he shows up at the venue in time before a pairing of another round.
An exception may be made in the case of a registered participant who has given written notice in advance that he will be unavoidably late.
- Where the Chief Arbiter decides to admit a latecomer,
- if the player's notified time of arrival is in time for the start of the first round, the player is given a pairing number and paired in the usual way.
- if the player's notified time of arrival is in time only for the start of the second (or third) round (“Late Entry”), then the player is not paired for the rounds which he cannot play. Instead, he receives no points for unplayed rounds (unless the rules of the tournament say otherwise), and is given an appropriate pairing number and paired only when he actually arrives.
- If there are late entries, the Pairing Numbers that were given at the start of the tournament are considered provisional. The definitive Pairing Numbers are given only when the List of Participants is closed, and corrections made accordingly in the results charts.
pairing numbers changes the pairings too. We would expect this to happen, if at all, in the first round of a tournament - in some (rare) instances even in the second or in the third round - and, when such changes happen, they make the checking of the pairings rather difficult. Hence, in order to make it easier to perform such checks on advanced stages of a tournament, the rule prohibits late changes of the pairing numbers.
As correct ratings, titles and so on are needed to correctly rate the tournament, such data may always be corrected, even in late rounds (and even after the tournament is finished!), but without changing the pairing numbers.
It seems appropriate to point out that the declaration of delay must be given in advance, in writing, and stating reasons for it. Verbal communications (telephone, etc.) do not suffice. Since exceptions may be made, it is the Arbiter’s responsibility to grant or decline such requests.
We want to take notice that the admission of a latecomer is a choice of the Chief Arbiter, who takes the final decision – and must take the responsibility too, especially if during the round there are empty seats... Thus, before accepting a latecomer and making the actual pairing, we want to be very sure that the player will actually be there in time to play. If we are not that sure, it is probably better to let the player enter the tournament, and be paired, only for a subsequent (second, third) round.
Entering a late player in the tournament causes the pairing numbers to change according to the new ranking list; some of the players will thus play the following rounds with a different pairing number, and this may cause some perplexity among the players. For example, consider a player, correctly registered from the beginning, but entering a tournament (say, with 100 players) on the second round, as #31. In the first round that player had no pairing number – hence, the
- Pairing, colour and publishing rules
- Adjourned games are considered draws for pairing purposes only.
- A player who is absent without notifying the arbiter will be considered as withdrawn unless the absence is explained with acceptable arguments before the next pairing is published.
- Players who withdraw from the tournament will no longer be paired.
- Players known in advance not to play in a particular round are not paired in that round and score zero (unless the rules of the tournament say otherwise).
- Only played games count in situations where the colour sequence is meaningful. So, for instance, a player with a colour history of BWB=W (i.e. no valid game in round-4) will be treated as if his colour history was
=BWBW. WB=WB will count as =WBWB, BWW=B=W as = =BWWBW and so on.
- Two paired players, who did not play their game, may be paired together in a future round.
- The results of a round shall be published at the usual place of communication at announced time due to the schedule of the tournament.
players who (now) have numbers 33, 35, 37 and so on, in the first round had even pairing numbers and thus the colour opposite to that of player #1.
By the way, we should also observe that the limit imposed in C.04.2.B.4 on the regeneration of pairing numbers does not extend to the case of a newly added late player.
Basically, we look only at actually played games, skipping “holes”, which float to the top of the list. Thus, for example, in the comparison between the colours histories of two players, the sequence
== WB is equivalent to =W=B and WB== (and the latter two are equivalent to each other!).
The application of this rule and the next requires us to set (and post!) a timetable for the publication of pairings. Above all, these rules put
- If either
- a result was written down incorrectly, or
- a game was played with the wrong colours, or
- a player's rating has to be corrected (and playing numbers possibly recomputed as in C.04.2.C.3),
and a player communicates this to the arbiter within a given deadline after publication of results, the new information shall be used for the standings and the pairings of the next round. The deadline shall be fixed in advance according to the timetable of the tournament.
If the error notification is made after the pairing but before the end of the next round, it will affect the next pairing to be done.
If the error notification is made after the end of the next round, the correction will be made after the tournament for submission to rating evaluation only.
a constraint on the possible revision of the pairings: if an error is not reported within the specified deadline, all subsequent pairings, as well as the final standings, shall be prepared making use of the wrong result as if it were correct.
- After a pairing is complete, sort the pairs before publishing them.
The sorting criteria are (with descending priority):
-
- the score of the higher ranked player of the involved pair;
- the sum of the scores of both players of the involved pair;
- the rank according to the Initial Order (C.04.2.B) of the higher ranked player of the involved pair.
- Once published, the pairings shall not be changed unless they are found to violate
C.04.1.b (Two players shall not play against each other more than once).
Even when using a pairing software program, it is mostly advisable to check boards order before publishing the pairing, because many players interpret even an incorrect board order as a “pairing error”.
C.04.3 FIDE (Dutch) System
Version approved at the 87th FIDE Congress in Baku 2016.
- Introductory Remarks and Definitions
-
- Initial ranking list
See C.04.2.B (General Handling Rules - Initial order)
-
- Order
For pairings purposes only, the players are ranked in order of, respectively:
-
-
- score
- pairing numbers assigned to the players accordingly to the initial ranking list and subsequent modifications depending on possible late entries or rating adjustments
-
-
- Scoregroups and pairing brackets
A scoregroup is normally composed of (all) the players with the same score. The only exception is the special “collapsed” scoregroup defined in A.9.
A (pairing) bracket is a group of players to be paired. It is composed of players coming from one same scoregroup (called resident players) and of players who remained unpaired after the pairing of the previous bracket.
A (pairing) bracket is homogeneous if all the players have the same score; otherwise it is heterogeneous.
A remainder (pairing bracket) is a sub-bracket of a heterogeneous bracket, containing some of its resident players (see B.3 for further details).
-
- Floaters and floats
- A downfloater is a player who remains unpaired in a bracket, and is thus moved to the next bracket.
In the destination bracket, such players are called “moved-down players” (MDPs for short)
Players are ordered in such a way that their presumable strengths are likely to decrease from top to bottom of the list (see also C.04.2:B).
Please notice that when we include a late entry, the list should be sorted again, thus assigning new pairing numbers to the players (C.04.2:C.2,3). The same may be done when some wrongly entered rating had to be corrected. When this happens, some participants may play subsequent rounds with new, different numbers; and, of course, this change may, if not adequately advertised, muddle players who, in reading the pairings, still look for their old numbers.
This definition solves any ambiguity between scoregroups and pairing brackets, stating that the scoregroup is the “backbone” of a pairing bracket, which is made of a scoregroup together with the players remaining from the pairing of the previous bracket. The players from the scoregroup are called “resident”, and usually have all the same score, which is called resident score and is the “nominal score” of the bracket. Only when the scoregroup is the “Special collapsed” one, the resident players may have different scores.
The difference is that in a homogeneous bracket there are no score differences between players to be taken care of (to be homogeneous, a bracket must be made of just a (normal) scoregroup and nothing more).
Article B.3 illustrates how to build a candidate pairing for a bracket and explains how and when a remainder is built and used.
A player may become a downfloater because of several reasons; first, the bracket may contain an odd number of players, so that one shall unavoidably remain unpaired. Then, the player may have no possible opponent (and hence no legal pairing) in the bracket. Sometimes, two or more players share between them a number of possible opponents in such a way that no player is incompatible, but we cannot pair all of them (e.g., two players with only one possible opponent, three players with only two possible opponents, and so on)2. Last, but not least, in some instances the player may have to float down, in order to allow the pairing of the following bracket.

2 This situation is sometimes (unofficially) called semi-incompatibility or island-(in)compatibility.
- After two players with different scores have played each other in a round, the higher ranked player receives a downfloat, the lower one an upfloat.
A player who, for whatever reason, does not play in a round, also receives a downfloat.
-
- Byes
See C.04.1.c (Should the number of players to be paired be odd, one player is unpaired. This player receives a pairing-allocated bye: no opponent, no colour and as many points as are rewarded for a win, unless the regulations of the tournament state otherwise).
-
- Colour differences and colour preferences
The colour difference of a player is the number of games played with white minus the number of games played with black by this player.
The colour preference is the colour that a player should ideally receive for the next game. It can be determined for each player who has played at least one game.
-
-
- An absolute colour preference occurs when a player’s colour difference is greater than +1 or less than -1, or when a player had the same colour in the two latest rounds he played. The preference is white when the colour difference is less
-
In analogy to “downfloater”, we will use the term “upfloater” to indicate a player paired to another one having a higher score3 (usually, the opponent of a downfloater).
Downfloats and upfloats are a sort of markers, used to record previous unequal pairings of the player. The reason to keep track of such pairings is that, in general, we want to minimise, and, as far as possible, avoid, their occurrence for the same players. Actually, a pairing between floaters constitutes a disturbance to the general principle of Swiss systems that the players in a pair should have the same score, and therefore the rule try to limit the repetition of such events4.
We want to notice that any player who did not play a round receives a downfloat. This is important because it affects the following two pairings for that player. For example, it becomes unlikely that such a player may receive a downfloat or get the PAB [A.5] in the next round5.
In other Swiss systems (e.g. Dubov) the player, whom the PAB will be assigned to, is selected before starting the pairing for the round.
In the FIDE (Dutch) system, on the contrary, the round-pairing (see A.9) ends up with an unpaired player, who will receive the pairing-allocated bye (PAB).
During pairing, we will try to accommodate (as much as possible) the colour preferences of the players – and this is the reason for the good balance of colours of Swiss modern systems.
Participants, who have not played any games yet, just have no preference, and shall therefore accept any colour (see A.6.d).
In general, the colour difference should not become greater than 2 or less than -2 – with the possible exception of high ranked players in the last round, which can receive, if necessary, the third colour in a row or a colour three times more than the opposite (but this is still a relatively rare event).
3 Please notice that in other Swiss pairing systems (e.g. Dubov), the same term “upfloater” may indicate a player transferred to a higher bracket.
4 We may also note that the FIDE (Dutch) system uses a “local” approach to this problem, which looks only to the last two rounds. On the contrary, the Dubov system adopts also a “global” approach, putting also a limit on the total number of floats in the whole tournament (three floats for tournaments up to nine rounds, four for longer tournaments).
5 On the contrary, the previous rules did not assign a downfloat to a player who forfeited a game, so such players had no protection against getting a PAB or a downfloat in the following round. Because of this, a weak player absent in the first round could get a PAB in the second round.
than -1 or when the last two games were played with black. The preference is black when the colour difference is greater than +1, or when the last two games were played with white.
-
-
- A strong colour preference occurs when a player’s colour difference is +1 (preference for black) or -1 (preference for white).
- A mild colour preference occurs when a player’s colour difference is zero, the preference being to alternate the colour with respect to the previous game he played.
- Players who did not play any games have no colour preference (the preference of their opponents is granted).
-
-
- Topscorers
Topscorers are players who have a score of over 50% of the maximum possible score when pairing the final round of the tournament.
-
- Pairing Score Difference (PSD)
The pairing of a bracket is composed of pairs and downfloaters.
To determine an absolute colour preference, we examine only the actually played rounds, skipping any unplayed games6 (whatever the reason may be) in compliance with [C.04.2:D.5] (e.g., the sequence WBBW=W gives an absolute colour preference).
Notice that any disregarded colour preference, be it strong or mild, will give origin to an absolute colour preference on the subsequent round.
If neither player has a colour preference (as is normal when pairing the first round, but may sometimes happen also in subsequent rounds), we resort to the colour allocation rules in section E. There, by means of the initial-colour (decided by drawing of lots before the pairing of the first round) and of rule E.5, we will be able to assign the correct colour to both players.
Such high-scoring players are especially important in the determination of the winner and of the top ranking7. Hence, we may apply some special treatment criteria to their pairings - e.g., a player may receive a same colour three times more than the other one, or three times in a row, if this is needed to make it meet an opponent better suited to the strength the player demonstrated.
This is an important idea: the pairing of a bracket is not made only of pairs: the downfloaters are part of it too – and a very important part, at that! In fact, as we shall see, the choice of the downfloaters may determine if it will be possible to pair the remaining players – and therefore if the pairing is a valid one.
6 Please note the difference with floats, for which we look at the last two rounds of the tournament schedule (but remember that an unplayed game gives a downfloat).
7 Not all the “topscorers” are really competing for top ranking places; nonetheless, they are more likely to be of importance in the formation of the top standings than low-ranked players, in several collateral ways – e.g. they may be opponents to prospective prize winners, or their score may give a determinant contribute in tiebreak calculations, and so on.
Its Pairing Score Difference is a list of score- differences (SD, see below), sorted from the highest to the lowest.
For each pair in a pairing, the SD is defined as the absolute value of the difference between the scores of the two players who constitute the pair.
For each downfloater, the SD is defined as the difference between the score of the downfloater, and an artificial value that is one point less than the score of the lowest ranked player of the current bracket (even when this yields a negative value).
Note: The artificial value defined above was chosen in order to be strictly less than the lowest score of the bracket, and generic enough to work with different scoring-point systems and in presence of non-existent, empty or sparsely populated brackets that may follow the current one.
PSD(s) are compared lexicographically (i.e. their respective SD(s) are compared one by one from first to last - in the first corresponding SD(s) that are different, the smallest one defines the lower PSD).
The Pairing Score Difference allows the best management of the overall difference in scores between the paired players. In practice, it is a list of the score differences, built as follows: we calculate the score differences (SD) in each pair and for each downfloater, then sort them from higher to lower, thus obtaining a string of numbers. Each single difference is taken in absolute value (so that it is always positive), because it’s irrelevant which one of the players have a higher score.
While the meaning of the SD is obvious for pairs, it is far less obvious for downfloaters, who have no opponent yet. Nonetheless, we need to account, somehow, for the perspective score difference relative to the player when it will finally be paired - in such a way that giving a float, or a PAB, to a higher scored player should be worse than giving it to a lower scored one. So we go for a “presumptive” score difference, establishing a hypothetical score for the residents of the (yet undefined!) next bracket.
In order to be sure that we can accommodate a wide variety of possible next brackets, we choose a value lower enough than that of the current bracket, namely one point less than the minimum score of its (resident) players. In the last two brackets, this may yield a negative value – e.g., in the 0.5 points bracket this value is -0.5 points. This is not a problem, as we will simply take the difference between a positive value and this one, so the result will always be positive.
Please note that in the last bracket the only possible downfloater is the player who is going to get the PAB. Thus, this calculation provides an easy and uniform way to minimise the score of the players who get the PAB.
PSDs are compared following the lexicographical order (the “order of the dictionary”). We start by comparing the first number of the first PSD with the first number of the second PSD: if one of those two is smaller than the other one, the PSD it belongs to is the “smaller”. If they are equal, we proceed to the second element of each PSD, and repeat the comparison. Then, if needed, we go on to the third, the fourth, and so on - until we reach the end of the strings8.
An alternative (but fully equivalent) method of comparison is the following: substitute a letter for each number of each PSD, following the correspondence A=0, B=0.5, C=1, D=1.5, E=2 and so on. Doing so, we transform the PSDs in
8 Of course, this method only has significance if the two PSD have the same length; but this is always the case, because the PSD comparison is used only when pairings with the same number of pairs are involved. Were the number of pairs different, we would never get to a PSD comparison.
-
- Round-Pairing Outlook
alphabetical words, which can be compared using the simple alphabetical order. The word that comes first (alphabetically) corresponds to the “smaller” PSD.
This article is essentially a guideline giving a panoramic vision of the pairing process, both in the more common case in which the pairing can be completed by normal means, and in the special case in which this is not possible. This is a very important thing to do, as the new Rules do not any more contain an algorithm to dictate a step-by-step procedure.
The pairing of a round (called round-pairing) is complete if all the players (except at most one, who receives the pairing-allocated bye) have been paired and the absolute criteria C1-C3 have been complied with.
If it is impossible to complete a round-pairing, the arbiter shall decide what to do.
Otherwise, the pairing process starts with the top scoregroup, and continues bracket by bracket until all the scoregroups, in descending order, have been used and the round-pairing is complete.
However, if, during this process, the downfloaters (possibly none) produced by the bracket just paired, together with all the remaining players, do not allow the completion of the round-pairing, a different processing route is followed.
We want to notice that that this definition refers not to a bracket but to the complete round. Thus, we cannot accept unpaired players (apart from a possible PAB) - all players must be paired. On the other hand, the constraints for such a pairing are very loose, not to say minimal – we are only asking for it to comply with the absolute criteria. This does not mean that we may feel free to make a poor pairing: in general, several complete pairings will be possible for each round, and “the” pairing – the correct one - shall simply be the one among them that best satisfies all the pairing criteria.
This is something really brand-new: for the first time ever, the case in which no pairing at all can be done is referred to by the rules. From a practical point of view, this is not a very helpful rule - in fact, in these (luckily rare) cases, the arbiters must act according to their best judgment
– but, at least, the possibility has been accounted for.
The pairing process starts with the topmost scoregroup; with it, we build the first bracket and try to pair it. This pairing may possibly leave some downfloaters that, together with the next scoregroup, will form the next bracket, and so forth – until all players have been paired.
Before starting the pairing of a bracket, we must verify that at least one legal pairing (i.e. a pairing that complies with the absolute criteria) exists for all the players as yet unpaired, together with the downfloaters (of course, possibly none) left from the bracket just paired9. This requirement is informally called the “Requirement Zero”, and its check is called a “Completion test”.
If this check fails before pairing the first bracket, there is no way at all to complete the round- pairing, so we have an impossible pairing - which is bad news.
When, on the contrary, this happens after the pairing of the first bracket, we already know that at least one legal pairing exists for the entire round (we checked this before pairing the first
9 Of course, this check is far simpler than the actual complete pairing, because (for the moment) we are not interested in finding the best (correct) pairing, but only in showing that at least a legal one exists.
The last paired bracket is called Penultimate Pairing Bracket (PPB). The score of its resident players is called the “collapsing” score. All the players with a score lower than the collapsing score constitute the special “collapsed” scoregroup mentioned in A.3.
The pairing process resumes with the re-pairing of the PPB. Its downfloaters, together with the players of the collapsed scoregroup, constitute the Collapsed Last Bracket (CLB), the pairing of which will complete the round-pairing.
Note: Independently from the route followed, the assignment of the pairing-allocated bye (see C.2) is part of the pairing of the last bracket.
bracket!). Nevertheless, if the set formed by the downfloaters together with all of the remaining players cannot be paired, it means that, given those downfloaters, we cannot complete the pairing without infringing the absolute criteria.
In this situation, the pairing produced by the last (in fact, still current!) paired bracket is not adequate, and we need to modify it before proceeding. We must restart with this same bracket, while changing the pairing conditions, in order to be able to find the pairing (which, as we already know, must undoubtedly exist). This change of conditions may have two effects: the first, and less invasive, is a different choice of downfloaters10, while the second is an increase in the number itself of downfloaters. (The latter is of course the only option available when the original pairing did not produce any floater.)
First, we pool together all the players, whose score is lower than the collapsing score. Then, with those players, we build the “special collapsed scoregroup” (SCS) - whose players are all resident, regardless of their score.
The bracket just tentatively paired, and which we are now going to pair again, is now called PPB11.
The primary goal in pairing the PPB is to have it produce a set of downfloaters that allows a complete pairing of the SCS [C.4]. With those downfloaters, together with the SCS, we build the CLB, which is by definition the last bracket. The pairing of those two brackets requires some special attentions12.
By stating that the assignment of the PAB is always part of the pairing of the last bracket, this note is telling us that criterion C.2, which regulates the assignment of the PAB, is only significant when the last bracket is in some way involved in the pairing – that is to say:
- when pairing the last bracket (be it a normal bracket or the CLB)
- when evaluating the optimisation of the next bracket (see C.7), in pairing the last-but-one (normal) bracket
- when re-pairing the PPB (after a completion failure), during the evaluation of C.4 (see)
10 Please note that we check (and, if necessary, change) the selected downfloaters in two completely different situations: the first is when we try to optimise the number of pairings and PSD in the next bracket (see C.7). The second is when the rest of the players cannot be paired and the PPB must give the correct floaters to allow a complete pairing. Here we are referring to the latter situation.
11 Actually, the name for this bracket comes from the previous version of the Swiss rules, which included a bracket with a similar function.
12 For further details, see [B.7].
Section B describes the pairing process of a single bracket.
Section C describes all the criteria that the pairing of a bracket has to satisfy.
Section E describes the colour allocation rules that determine which players will play with white.
- when checking that the floaters give a legal pairing for the remaining players (completion test).
Without this note, we might think the allocation of the PAB to be something to be done after having paired the last bracket – in fact, just as if that bracket had produced a floater - to be paired with a fictitious player in a virtual after-the-last bracket. Hence, if that player could not receive the PAB, we would have to consider the last bracket as the PPB, and subsequently restart the pairing process from this point of view... This note is specifically meant to avoid any possible ambiguity, explicitly excluding such an interpretation.
Moreover, the note also states that, even when it is readily apparent that from the current bracket a downfloater will result, who is bound to get the PAB (e.g., in the next bracket(s) there is no player who can get it), the choice of the floater shall not keep in mind the allocation of the PAB.
We should also notice that pairs are made based also on expected colours, but actual colour assignment is only done at the end of the pairing.
For those who knew the old version of the Dutch rules, it may be useful to spend some words about the new structure. The new Sections B and C contain all the rules that were previously detailed in the algorithmic section with the support from (previous) Section B. Nonetheless, in the previous version of the Rules, the pairing route was different. When the pairing of a bracket was completed, it was accepted (for the moment), and the pairing went forward to the next bracket. If the next bracket was satisfactorily paired (and, sometimes, not even satisfactorily, since a downfloater could create a situation in which a resident player of the new bracket was made incompatible), the pairing for the previous one became (almost) final. If, on the contrary, a better pairing was possible for the next bracket (i.e., one that produced more pairs, or a smaller PSD), we went back to the previous bracket (backtracking) to pair it again, looking for better downfloaters. This is of course equivalent to verify that the floaters produced are the best possible choice before starting the pairing of the next bracket.
For the last bracket, where an unsatisfactory pairing means the impossibility to complete the pairing, the backtracking could be more complicate. First, when pairing the last bracket, a simple backtracking to the previous one was not always enough. Sometimes we had to join (“collapse”) those two brackets, in order to be able to gain access to the preceding bracket and change its floaters
- and sometimes this process had to be repeated until an acceptable pairing was found.
It is readily evident that this backwards course had to go up, starting from the last bracket, until the point was reached, in which the produced downfloaters did actually allow the pairing of the rest of the players. Hence, the backtracking did necessarily extend until it reached the bracket that, with the look-ahead methodology, is at once defined as the PPB - thus bringing us back to the same conditions. The new look-ahead method is then equivalent to the backtracking - with the advantage of a fairly simpler logic.
Anyway, the new wording of the Rules does not specify any particular method to enforce compliance with the pairing criteria. Hence, both the arbiter and the programmer enjoy complete freedom in choosing their preferred method to implement the system (look-ahead, backtracking, weighted matching or other), as long as the rules are fully complied with.
- Pairing process for a bracket
This section’s goal, from the Rules standpoint, is to univocally define the sequence of generation for the candidate pairings - and, to this aim, it precisely defines the constraints inside which the pairing must be built. From the arbiter’s point of view, however, this section may also be used as a roadmap to actually build the pairing and evaluate its quality. In fact, it can be readily adopted as a guideline to make - or, far more often, prove - a pairing.
- MaxPairs is the maximum number of pairs that can be produced in the bracket under consideration (see C.5).
Note: MaxPairs is usually equal to the number of players divided by two and rounded downwards. However, if, for instance, M0 is greater than the number of resident players, MaxPairs is at most equal to the number of resident players.
- M1 is the maximum number of MDP(s) that can be paired in the bracket (see C.6).
Note: M1 is usually equal to the number of MDPs coming from the previous bracket, which may be zero. However, if, for instance, M0 is greater than the number of resident players, M1 is at most equal to the number of resident players. Of course, M1 can never be greater than MaxPairs
-
- Subgroups (original composition)
To make the pairing, each bracket will be usually divided into two subgroups, called S1 and S2.
S1 initially contains the highest N1 players (sorted according to A.2), where N1 is either M1 (in a heterogeneous bracket) or MaxPairs (otherwise).
S2 initially contains all the remaining resident players.
When M1 is less than M0, some MDPs are not included in S1. The excluded MDPs (in number of M0 - M1), who are neither in S1 nor in S2, are said to be in a Limbo.
Note: the players in the Limbo cannot be paired in the bracket, and are thus bound to double- float.
In a given bracket we have a given number M0 of MDPs13 (possibly none), but we have no certainty that all those MDPs can be paired14.
Thus, we define a second parameter M1, representing the number of MDPs that can actually be paired - where, of course, M1 is less than or equal to M0. In summary, the bracket will contain MaxPairs pairs, at most M1 of which contain a downfloater.
We want also to observe that, while M0 is a well- known constant, we usually do not know precisely how many players, and especially MDPs, can be paired, until the actual pairing is made – actually, we need to “divine” M1 and MaxPairs out of sound reasoning, assuming a tentative value, which might initially be wrong. Nonetheless, those numbers, however identified, are considered constants - and that is why there is no rule to change them.
The composition of the original subgroups is different when we have MDPs, because those players, having already floated, need now some “special protection”.
In setting the number of pairs to be done to M1 for heterogeneous brackets, we focus only on MDPs, who (or, at least, the maximum possible number of them) actually are to be paired first15. On the contrary, setting the number of pairs to MaxPairs says that we are trying to pair the entire bracket all at once (so it must be homogeneous).
After M1 moved-down players have been selected for pairing, the remaining MDPs, in number M0- M1, cannot be paired in the bracket16. Those players form a subgroup called “Limbo”. During the pairing proceedings, it may happen that some players need to be swapped between S1 and the Limbo - but, at the end of the pairing, the players still in the Limbo will be bound to float again.
13 We want to remember that the “Moved-down players” (MDPs) are the downfloaters of the previous bracket.
14 For example, the number of MDPs may be greater than MaxPairs; or some among them may be incompatible; or we may have a semi-incompatibility, in which a group of players ‘compete’ for too few possible opponents, just like the situation described in the comment to A.4.
15 To avoid any misunderstanding, please take notice that this is only a procedural indication that has nothing to do with the order of generation of candidates. In fact, independent of the method and algorithm used to generate them, each candidate is regarded as a whole; and, when we choose the ‘earlier’ candidate from a pool of equivalent ones, we only consider the order of generation of the complete candidates.
16 Those players are not necessarily incompatible in the bracket – there may just be no place to pair them. E.g., if two MDPs share the same one possible opponent, neither of the two is incompatible - but nonetheless one of the two MDPs cannot be paired!
-
- Preparation of the candidate
S1 players are tentatively paired with S2 players, the first one from S1 with the first one from S2, the second one from S1 with the second one from S2 and so on.
In a homogeneous bracket: the pairs formed as explained above and all the players who remain unpaired (bound to be downfloaters) constitute a candidate (pairing).
In a heterogeneous bracket: the pairs formed as explained above match M1 MDPs from S1 with M1 resident players from S2. This is called a MDP-Pairing. The remaining resident players (if any) give rise to the remainder (see A.3), which is then paired with the same rules used for a homogeneous bracket.
Note: M1 may sometimes be zero. In this case, S1 will be empty and the MDP(s) will all be in the Limbo. Hence, the pairing of the heterogeneous bracket will proceed directly to the remainder.
A candidate (pairing) for a heterogeneous bracket is composed by an MDP-Pairing and a candidate for the ensuing remainder. All players in the Limbo are bound to be downfloaters.
-
- Evaluation of the candidate
If the candidate built as shown in B.3 complies with all the absolute and completion criteria (from C.1 to C.4), and all the quality criteria from
C.5 to C.19 are fulfilled, the candidate is called “perfect” and is (immediately) accepted. Otherwise, apply B.5 in order to find a perfect candidate; or, if no such candidate exists, apply B.8.
-
- Actions when the candidate is not perfect
Here is where we build the candidate pairing. In the most general case, this is done in two steps:
- first, we build M1 pairs, each of them containing an MDP,
- then, we pair the remaining resident players.
Of course, if the bracket is homogeneous, or if none of the MDPs is pairable (i.e. if M1 is zero), the first step is omitted.
Thus, in general, the candidate comprises three parts:
- an MDP-Pairing (heterogeneous brackets only), made of M1 pairs (maybe none) containing an MDP and a resident player each;
- a set of pairs of resident players, coming from the pairing of the homogeneous bracket; or from the pairing of the remainder of a heterogeneous bracket;
- a set of unpaired players, coming both from the Limbo and from the resident players that cannot be paired - and hence can’t help but get a downfloat.
Having prepared a candidate, we must evaluate its quality; that is, we must check the compliance of the candidate with the pairing criteria given in Section C.
If we are very lucky, it may be “perfect”: in this case, we accept it straight away.
Otherwise, we must apply some changes to try and make it perfect (B.5). If this proves impossible, the last resource is accepting a candidate that, although it is not perfect, is nonetheless the best we can have (B.8). Of course, a candidate that does not comply with the absolute criteria is not even acceptable.
After the pairing is made, and before accepting it and proceeding to the next bracket, we will have to perform a completion test, to check that all the remaining players, including the downfloaters from the bracket just paired, allow the round- pairing to be completed (see A.9). If this completion test fails, we define the Collapsed Last Bracket and proceed as explained in A.9.
The composition of S1, Limbo and S2 has to be altered in such a way that a different candidate can be produced.
The articles B.6 (for homogeneous brackets and remainders) and B.7 (for heterogeneous brackets) define the precise sequence in which the alterations must be applied.
After each alteration, a new candidate shall be built (see B.3) and evaluated (see B.4).
The process of pairing is an iterative one: if the pairing is not perfect, we try (one by one) a precise sequence of alterations in the subgroups S1, Limbo, and S2, and each time we repeat the preparation and evaluation of the candidate. There are, in fact, two different sequences:
- one for homogeneous brackets (B.6), which contain no MDPs; this sequence also applies to remainders
- one for heterogeneous brackets (B.7); those contain MDPs, some of which (in number M0-M1, which may be zero) are in a Limbo, so the alterations must keep into account not only the usual possible alterations in S1 and S2, but also the possibility to change the composition of the Limbo.
The first perfect candidate found in this process is the required pairing. If there is no perfect candidate, we shall have to use the best available one; since we are scrutinizing all candidates, we can find this best candidate as we proceed. To do that, when we find the first legal (but not perfect) candidate, we mark it as a “provisional-best”. Each time we find another legal candidate, we shall compare17 it with the current provisional- best candidate. If the former is better than the latter, we store it as the new provisional-best; otherwise, we keep the old one. In the end, all candidates have been examined; hence, the surviving provisional-best is actually the best possible (although imperfect) candidate, which will be accepted as pairing, because of rule B.8.
The main guideline to carry out this task is the “minimum disturbance”: every alteration must be the minimum possible, so that the resulting pairing can be as similar as possible to a “perfect” one.
For more detail about the iterative pairing process, see B.6 and B.7.
-
- Alterations in homogeneous brackets or remainders
Alter the order of the players in S2 with a transposition (see D.1). If no more transpositions of S2 are available for the current S1, alter the original S1 and S2 (see B.2) applying an exchange of resident players between S1 and S2
Since we are now managing only homogeneous brackets, we do not need to worry about pairing MDPs.
The possible actions to be tried here are:
17 Two candidates are compared based on the compliance with the pairing criteria, which are defined in order of priority in section C. The first check is on the priority of the higher infringed criterion: the higher it is, the lower is the quality of the candidate. Then the second check is on a “failure value” which is peculiar to that criterion – this will often be the number of times the criterion is infringed (e.g., the numbers of disregarded colour preferences) but it may also be of a completely different nature (e.g., the PSDs of two candidates to be compared). Then we go to the second higher infringed criterion; then to the latter’s failure value - and so on until we find a difference. When there is no difference at all, the first generated candidate takes precedence.
(see D.2) and reordering the newly formed S1 and S2 according to A.2.
- a transposition, consisting in applying a different order to the players in S2. In simple words, a transposition “shuffles” the players in S2 according to specific rules (see D.1), but keeping them separate from the players of S1. This leads to a change in the second player in some pairs. The basic idea is to alter the pairing by modifying players’ order in as low as possible rankings.
- an exchange, consisting in swapping one or more players from subgroup S1 with the same number of players from subgroup S2. As above, the basic idea is to try to alter the pairing as little as possible. To this aim, we swap players in as low as possible rankings of S1 with players in as high as possible rankings of S2 - assuming that, being near in ranking, they have more or less equivalent playing strength. After any exchange, both the subgroups S1 and S2 must be put in order again with the usual rules. An exchange makes the pairing between players of the same original subgroup possible.
After we made transpositions in a bracket, alterations in the order are desired; hence, players in the S2 subgroup should not be sorted again (while S1 does not need to be sorted, as it has not been changed).
On the contrary, after exchanges, which swap one or more players between subgroups S1 and S2, we must sort both subgroups S1 and S2 according to A.2, to re-establish a correct order before beginning a new sequence of pairing attempts. If the first attempt of the new exchange fails to give a valid result, we will try transpositions too, thus changing the natural order in the modified S2.
Both transpositions and exchanges should not be applied at random: to comply with the general principle of minimal disturbance of the pairing, section D dictates a precise sequence of possible transpositions and exchanges. This sequence begins with alterations that give only mild disturbances to the pairing (with respect to the “natural” one), moving gradually towards those changes that cause definitely important effects.
The order of actions is as follows: first, we try, one by one, all the possible transpositions (see D.1). If we find one that allows a perfect pairing, the process is completed. Otherwise, we try the first exchange (see D.2): with this, we proceed
-
- Alterations in heterogeneous brackets
Operate on the remainder with the same rules used for homogeneous brackets (see B.6).
Note: The original subgroups of the remainder, which will be used throughout all the remainder pairing process, are the ones formed right after the MDP-Pairing. They are called S1R and S2R (to avoid any confusion with the subgroups S1 and S2 of the complete heterogeneous bracket).
If no more transpositions and exchanges are available for S1R and S2R, alter the order of the players in S2 with a transposition (see D.1), forming a new MDP-Pairing and possibly a new remainder (to be processed as written above).
again to try every possible transposition18, until we succeed - or use them up. In the latter case, we try the second exchange, once again with all the possible transpositions, and so on.
If we get to the point in which we have used up all the possible transpositions and exchanges, then a perfect pairing simply does not exist. In that case, we apply B.8, thus accepting a less than perfect result.
This article, a companion to the previous one, addresses the case of heterogeneous brackets. This kind of bracket is paired in two logical steps19:
- in the first step, we build an MDP-Pairing (see B.3), which takes care of the pairable moved-down players (as many as possible of them), giving raise to a remainder (and possibly a Limbo).
- in the second step, after the MDPs have been paired, we proceed to pair the remainder, which is made only of resident players (but we need to take notice that, when we are processing a CLB, those players may well have different scores. In this case, the PSD is of importance and must be accounted for – we will go back to this presently).
The rules to operate on the remainder are just the same that apply for a homogeneous bracket. The difference shows only when we reach the point in which all of the possible transpositions and exchanges in the remainder have been unsuccessfully tried.
In a homogeneous bracket, this is the moment when we lower our expectations, settling for a less than perfect pairing (see B.6). In a heterogeneous bracket, however, we are not yet ready to surrender: before laying down arms, we can try to change the composition of the remainder.
To do that, we try a new, different MDP-pairing by applying a transposition to the original subgroup S2 (viz. the subgroup S2 of the complete
18 Suppose we exchanged player A from S1 with player B from S2. After the exchange, player B, now in S1, has a rank that is lower than that of player A, now in S2. As transpositions proceed, we will get to a point in which the candidate puts together players B and A – and then of course some other pairs of players. Now, before making the exchange, we tried all transpositions in S2, and thus also the one which contains the pair A-B and all the same other pairs as well – in summary, this candidate has already been evaluated! Reasoning along the same lines, we reach the conclusion that the same holds true also for exchanges involving more players. We can thus deduce that every time a pair contains a player from S1 with a lower rank (higher BSN) than its opponent from S2, this pair belongs to a candidate that has already been evaluated, and therefore we do not need to evaluate it again.
19 Of course, a practical implementation need not necessarily compose the pairing in two steps, as long as the final effect is the same as specified by the rules. bracket, not that of the remainder!). This may leave us with a new, different remainder, which we process (just as described above) trying to find a complete pairing – and, if we have no success, we try transposition after transposition until we succeed, or exhaust them all20
If no more transpositions are available for the current S1, alter, if possible (i.e. if there is a Limbo), the original S1 and Limbo (see B.2), applying an exchange of MDPs between S1 and the Limbo (see D.3), reordering the newly formed
As we hinted above, the PPB and the CLB are subject to slightly different pairing rules: the downfloaters of the PPB are no longer required to optimise the pairing in the next bracket (as it would be for normal brackets, see C.7), but just to allow it (see C.4). With those downfloaters, together with the SCS, we build the CLB, which is (by definition) the last bracket.
This is a rather unusual bracket: it is by definition heterogeneous21, and its residents often have different scores (because they come from the SCS). Its pairing is different from that of the usual heterogeneous bracket in that we have a remainder that must be paired just like if it were homogeneous, but without disregarding the needs of players with different scores.
Thus, we must enforce some criteria that usually are not important in remainders. The main goal in pairing the CLB is to get the lowest possible PSD (because, basically, the number of pairs is determined by the number of PPB floaters). To find this minimum PSD, we have to look not only at the MDP(s) and at their opponents (as usual), but also at the pairs that can be made inside the remainder (i.e. between SCS residents).
When several candidates have the lowest possible PSD, we must also enforce some criteria for the remainders, which are not usually required. If in a pair there are players with different scores, to such players we must apply all those criteria that limit the repetition of floats [C.12 to C.15] and the score difference of the protected players whose protection has already failed once or more [C.16 to C.19].
If all the possible transpositions have been used up, we have a resource yet: trying to change the MDPs to be paired. Of course, this is only possible if there is a Limbo in the bracket. In this case, we can exchange one or more of the MDPs with a same number of players from the Limbo. This is called an MDP-exchange (see D.3).
20 Actually, we do not need to try all of the transpositions, because not all of them are meaningful: in fact, we only have to try those transpositions that actually change the players, or their order, in the first part of the subgroup S2 – i.e. those players, who are going to be paired with the MDPs from S1. All the other players in S2 do not take part in this phase of the pairing and are thus irrelevant (at least for the moment).
21 Remember that the CLB is born from a failure in a completion test. This means that the “rest of the players”, with the current downfloaters (possibly none!) from the just (unsuccessfully!) paired bracket, cannot be paired - it therefore requires some adequate MDPs.
S1 according to A.2 and restoring S2 to its original composition.
-
- Actions when no perfect candidate exists
Choose the best available candidate. In order to do so, consider that a candidate is better than another if it better satisfies a quality criterion (C5- C19) of higher priority; or, all quality criteria being equally satisfied, it is generated earlier than the other one in the sequence of the candidates (see B.6 or B.7).
After any MDP-exchange, we are actually pairing an altogether different bracket; hence, we need to reorder S1 and restore S2 to its original composition, in fact starting the pairing process anew. As it was for the homogeneous case, the MDP-exchanges must be tried in the correct sequence, one by one; and, for each one of them, we shall try all the possible transpositions in S2, thus generating a different remainder - that will of course have to undergo all the usual pairing attempts as described above.
This is where we must make ourselves content with what best we can: if we arrive here, we have already tried all possible transpositions and exchanges, only to reach a simple, if dismal, conclusion - there is no perfect candidate! Hence, we choose the best available candidate, which is the final provisional-best found during the evaluation of all candidates as illustrated in B.5.
The Sieve Pairing
A very interesting alternative to this method – not necessarily a practical one, but very important from the theoretical point of view – is the one we shall call “Sieve pairing” (because of its similarity with the famous Eratosthenes' Sieve).
The basic idea is very simple: we build all the possible acceptable pairings (i.e., all those that comply with the absolute criteria). Then we start applying all the pairing criteria, one by one - but this time we start with the most important one and proceed downwards.
Each criterion will eliminate part of the acceptable pairings, so that, as we proceed, the number of candidates becomes lower and lower. If, at some stage of the process, only one candidate remains, we choose that one – it may even be a rather bad one, but there is nothing better.
If, after applying all the pairing criteria, we are left with more than one candidate, then we choose the one that would be the first to be generated in accordance with the sequence defined by Section B.
- Pairing Criteria Absolute Criteria
The absolute criteria correspond to the requirements of Section C.04.1, “Basic Rules for Swiss Systems” in the FIDE Handbook, which we may want to look at closely.
No pairing shall violate the following absolute criteria:
C.1
See C.04.1.b (Two players shall not play against each other more than once
Those criteria must be complied with always: they cannot be renounced, whatever the situation22. To enforce them, players may even float down as needed.
If the game is won by forfeit, for the purposes of pairing those two players have never met. As a result, that pairing may be repeated later in the tournament (and sometimes this happens, too!).
22 There are however situations in which no pairing at all exists, which complies with the absolute criteria – in such cases, the arbiter must apply his better judgment to find a way out of the impasse (see A.9).
C.2
See C.04.1.d (A player who has already received a pairing-allocated bye, or has already scored a (forfeit) win due to an opponent not appearing in time, shall not receive the pairing-allocated bye)
C.3
Non-topscorers (see A.7) with the same absolute colour preference (see A6.a) shall not meet (see
C.04.1.f and C.04.1.g)
Completion Criterion C.4
If the current bracket is the PPB (see A.9): choose the set of downfloaters in order to complete the round-pairing
Quality Criteria
Please notice that, contrary to the previous rules, only PABs and forfeit wins prevent the allocation of a PAB (see A.5) – on the contrary, a player who received a requested bye (usually, half point) may receive the PAB in a subsequent round.
This criterion does not apply to topscorers (A.7) or topscorers’ opponents, who are the only possible exception to C.04.1.f/g.
Two players, who cannot be paired to each other without infringing criteria C.1 or C.3, are said to be incompatible.
This is an absolute criterion too, but it applies only to the processing of the PPB – hence, only after a completion test failure (see A.9). Contrary to ordinary brackets (whose downfloaters are chosen in order to optimise the pairing of the next bracket - see C.7), for the PPB we just require a choice of downfloaters that allows a completion of the round-pairing - independent from the optimization of the next bracket, which is of course the CLB, and hence must be completely paired.
Please note that, since C.4 precedes both C.5 and C.6, the compliance with this criterion may cause a reduction in the number of pairs, or an increase in the final PSD, with respect to the previous pairing23.
The above criteria set conditions that must be obeyed: a candidate that does not comply with them is discarded. The following criteria are of a different kind, in that they establish a frame of reference for a quantitative evaluation of the “goodness” of the pairings, by setting a sequence of “test points” in order of decreasing importance, according to the internal logic of the system. The level of compliance with each one of the following criteria is not a binary quantity (yes/no) but a numerical (integer or fractional) quantity. We will measure it by means of a “failure value”, whose meaning is of course tightly connected to the criterion itself (e.g., the number of pairs less than MaxPairs for C.5, or the number of players not getting their colour preference for C.10, and so forth).
When we compare two candidates, we in fact compare the failure values of the candidates for each criterion, one by one, in the exact sequence given by the Rules. If the two failure values are identical, we proceed to the next criterion. If they are different, we keep the candidate with the better value and discard the other one.
It seems worth noting that a candidate having a better failure value on a higher criterion is selected, even if the failure values for the following criteria are far worse. In other words, the optimisation with respect to a higher criterion may have a dramatic impact on the remaining failure values – and,
23 Of course, since the bracket we are pairing is a PPB, it has already been paired once.
we may add, the optimisation with respect to a criterion is always only relative to the current status, because even a small difference in a higher criterion may change the situation completely.
To obtain the best possible pairing for a bracket, comply as much as possible with the following criteria, given in descending priority:
C.5
Maximize the number of pairs (equivalent to: minimize the number of downfloaters).
Relative criteria are not so important as absolute ones, and they can be disregarded, if this is needed to achieve a complete pairing. In general, they are not important enough to make a player float – in fact, the first one of them, and hence the most important, instructs us to do just the very opposite, minimising the number of downfloaters!
Apart from the remaining player in odd brackets, only incompatible (or semi-incompatible) players should float. This too is an evidence of the attention of the FIDE (Dutch) system towards the choice of the “right strength opponent”.
The first “quality factor” is of course the number of pairs, a reduction of which increases the number of floaters (and, usually, also of the overall score difference between players).
Maximising the number of pairs actually means, build MaxPairs pairs (see B.1). At the beginning of the pairing process, though, MaxPairs, or the maximum number of pairs that can be built (which is a constant of the bracket), is actually unknown – hence, we need to "divine" it.
Actually, the only things we know for sure are the total number N of players in the bracket, and the number M0 of MDPs entering the bracket. We want to observe that the number of pairs can never be greater than N/2; thus, this value should make a good starting point, independent of the kind of bracket (homogeneous or heterogeneous).
The actual value of MaxPairs can be less than that, because some players might be impossible to pair in the bracket. Moreover, if this bracket is a PPB, it must also provide the downfloaters required to complete the round-pairing (see C.4), and that might detract to the number of pairs that can actually be built. Hence, the process to determine MaxPairs value is somewhat empirical and may require some “experimenting”.
If the bracket is heterogeneous (M0≠0), then as many MDPs as possible (M1) must be paired. They will be paired first, before proceeding with the rest of the players (see B.3) - but, as it happened for the value of MaxPairs, we still do not know the true value of M1, and we must divine it too. A first educated guess for its value is M0 – minus, of course, any incompatible MDPs.
If there is no way to make all those pairs, our estimate of the value of M1 was apparently too optimistic – in this case, we will have to gradually decrease it, until we succeed. Any remaining MDPs join the Limbo (see B.2) and shall
C.6
Minimize the PSD (This basically means: maximize the number of paired MDP(s); and, as far as possible, pair the ones with the highest scores)
C.7
If the current bracket is neither the PPB nor the CLB (see A.9): choose the set of downfloaters in order first to maximize the number of pairs and then to minimize the PSD (see C.5 and C.6) in the following bracket (just in the following bracket).
eventually float (after the completion of the pairing for the bracket).
The number of pairs made in the MDP-pairing will be subtracted into the total number of pairs to be made in the bracket, yielding the (plausible) number of pairs to be built in the remainder24.
Here too applies the same line of reasoning: if we cannot make all those pairs, our initial estimation of MaxPairs was apparently too optimistic – hence, we will have to gradually decrease their number. Any remaining players become downfloaters, and will eventually float down into the next bracket.
The same line of reasoning also holds for a homogeneous bracket - which, by definition, contains no Limbo or MDPs, but is otherwise essentially similar to a remainder.
In heterogeneous brackets, even when the same number of pairs is made, different choices of floaters, or different pairings, can lead to different mismatching between players’ scores (for an example, see the many possible ways to pair a heterogeneous bracket containing many players all having different scores). This important criterion, directly related to rule C.04.1:e, directs us to minimise the overall difference in scores. Its location before the colour related criteria (C.8-C.11) is suggestive of the attention the FIDE (Dutch) system gives to the choice of a “right strength” opponent rather than a “right colour” one.
The method to compute and compare the PSDs is explained in detail in the comment to article A.8.
When we get here, we have already complied with the absolute criteria (hence the pairing is a legal one) and optimised the most important pairing quality parameters (number of pairs, PSD).
Before going ahead to optimise colours and MDPs treatment, we take a look ahead to the next bracket. We do not want to ever come back to the current bracket again. Thus, we must make sure that the choice of downfloaters we are going to send to the next bracket will be the best possible one to comply with C.5 and C.6.
First, we check that the downfloaters (which will be the MDPs of the next bracket) will allow us to compose the maximum possible number of pairs.
24 We always want to remember that the pairing of the MDPs and of the remainder are two phases of a single operation, which is performed as a unit. Thus, we do not “go back” from the remainder pairing to the MDP-pairing, because we are already inside the same operation. For example, let us suppose that the current bracket produces only one downfloater and that the next scoregroup contains an odd25 number of players, one of which has no possible opponent. If we can choose between two possible downfloaters, both compatible in the destination bracket, but only one of them can be paired to the “problematic” player, we must choose that one - because choosing the other one would leave an incompatible player (and hence an unavoidable downfloater!) in the destination target. Only when the number of pairs have been maximised, we proceed to look into the PSD in the destination target. This in practice means that, when we may choose between two or more possible downfloaters, if all other conditions are equivalent, we must choose the downfloater that may be paired with the lowest score difference26. This optimisation is to be extended only to the next bracket. Actually, there are situations in which a small change in a previous pairing would bring in large benefits - but looking several brackets ahead would be too much difficult an operation to be carried on every time. So the rules settle for a practical optimisation, renouncing those that are out of reasonable reach. But the reason is not only this one: in the basic philosophy of the FIDE (Dutch) system, the pairings for the higher ranked players are considered far more important than those for the lower ones. Hence, altering the pairing of the current bracket for the benefit of some player, who is located two brackets below this one, would simply be opposite to that philosophy.
Having already made sure that both the number of floaters and their scores are at a minimum, we now start to optimise colour allocation. Actually, colour is less important than difference in score – and that’s why, consistently with the basic logic of the system, the colour allocation criteria are located after those that address number of pairs and PSD.
C.8
Minimize the number of topscorers or topscorers' opponents who get a colour difference higher than +2 or lower than -2.
C.9
Article C.3, in accordance with C.04.1:f-g, states that when two non-topscorers meet, their absolute preferences must be complied with. Here we have the special case of a topscorer who, for some reason, is bound to be paired with a player (who may or may not be also a topscorer)
25 Please note that if the next scoregroup contained an even number of players, the bracket built with it and the current downfloater would be odd. Hence, it would in any case produce (at least) one downfloater and the choice of the MDP would not be critical for the number of pairs.
26 Since this criterion does not apply for the PPB, the next bracket's resident players will all have the same score. Thus, it is not possible for moved-down players to be paired with players having different scores - but, if they cannot be paired in the bracket, they will have to float again, and this makes the PSD change!
Minimize the number of topscorers or topscorers' opponents who get the same colour three times in a row.
C.10
Minimize the number of players who do not get their colour preference.
having the same absolute preference. The outcome of those players’ games may be very important in determining the final ranking and podium positions; and this is an exception explicitly provided for by C.04.1:f-g, so we may compose such pairs. Thus, we choose the best possible matched opponent – but there must not be more such pairs than the bare minimum.
The subdivision into two individual rules establishes a definite hierarchy, giving more importance to colour differences than to repeating colours. Suppose that, for one same opponent, we can choose between two possible topscorers, and all those players have the same absolute colour preference. In this case, we must select the components of the pair in such a way that colour differences are minimised (as far as possible).
As hinted above, a player, who has an absolute colour preference without being a topscorer, may happen to be paired with a topscorer having an identical absolute colour preference. These two rules equate the players of the pair - thus, a player might be denied its absolute colour preference just as if it were a topscorer, even if it is not one!
We can have an idea about the minimum number of players who cannot get their colour preference, by inspecting the bracket, prior to the pairing.
Let us suppose that m players prefer a colour and n players prefer the other one, with m ≥ n. We can thus compose no more than n pairs in which the players are expecting different colours; and the colour preferences in these pairs can - and must - be satisfied.
The remaining m-n players all expect the same colour; and they will have to be paired among themselves. In each of the pairs thus composed, one of the two players cannot get its preferred colour. The number of such pairs, and henceforth of such players too, is x=(m-n)/2, rounded downward to the nearest integer if needed. Sometimes, in addition to those m+n players, the bracket contains also a more players who have no colour preference at all. Those players may get any colour, but, of course, they will usually get the minority colour, so that they will subtract to the number of disregarded preferences. Taking one more step further, we may reason that we can build a maximum of MaxPairs pairs. Among those, n+a pairs can satisfy both the colour preferences, whilst the remaining x=MaxPairs- n-a cannot help but disregard one colour preference. Of course, x cannot be less than zero
C.11
Minimize the number of players who do not get their strong colour preference.
(a negative number of pairs has no practical meaning); thus, we obtain the final and general definition for x:
x = max (0, MaxPairs-n-a)
Please take notice that a perfect pairing always has exactly x disregarded colour preferences – no more, no less.
Actually, there might be even more pairs in which a player does not get its preference - because of incompatibilities due to absolute criteria, as well as “stronger” relative ones. Thus, at first we propose to make the minimum possible number of such pairs – but we may need to increase this number, to find our way around various pairing difficulties.
Since the general philosophy of the FIDE (Dutch) system gives more importance to the correct choice of opponents than to colours, the pairs containing a disregarded colour preference will typically be among the first to be made27.
Only now, having maximised the number of “good” pairs, we can set our attention to satisfying as many strong colour preferences as possible.
The minimum number of players not getting their strong colour preference, which is usually represented by z, is of course a part of the total number x of disregarded colour preferences (see note to C.10) – therefore, z is at most equal to x.
For instance, let the number WT of white seekers be greater than the number BT of black seekers (we call White “the majority colour”). The x players will all be White seekers, and as many as possible among them should have mild colour preferences, while the rest will have strong colour preferences28. Hence we can estimate z simply as the difference between x and the number WM of White seekers who have a mild colour preference, with the obvious condition
27 Actually, transpositions swap players beginning with the last positions of S2 and going upwards, causing the bottom pairs of the bracket to be modified early in the transposition process, while the top pairs are modified later. Hence, a “colour-defective” pair located at the bottom of the candidate has a higher probability to be changed soon than a similar pair located at the top – therefore, perfect pairings with top “colour-defective” pairs have a definitely higher probability. Incidentally, we might also mention that players often seem to worry about “colour doublets” (like, for example, WWBB) and think that such colour histories are more frequent with the FIDE (Dutch) system than with other Swiss pairing systems. This is not so. In fact, such histories are usual enough (and unavoidable) in all manners of Swiss pairings – in the FIDE (Dutch) system they may seem more frequent just because they appear more often in the top pairs of the bracket, therefore involving higher ranked players, which makes them more noticeable.
28 We want to notice that, during the last round, some absolute colour preferences might be disregarded for topscorers or their opponents (see C.8, C.9), so that part of x may represent such players. In those instances, our line of reasoning should be suitably adapted. that z cannot be less than zero; hence:
z = max ( 0, x – WM ) if WT ≥ BT (White majority)
z = max ( 0, x – BM ) if WT < BT (Black majority)
With a careful choice of transpositions and/or exchanges, we might be able to minimise the number of disregarded strong preferences29.
For several reasons, however, the number of players who cannot get their strong preference may be greater than that.
The following group of criteria optimises the management of floaters, which is the last step towards the perfect pairing.
C.12
Minimize the number of players who receive the same downfloat as the previous round.
C.13
Minimize the number of players who receive the same upfloat as the previous round.
C.14
Minimize the number of players who receive the same downfloat as two rounds before.
C.15
Minimize the number of players who receive the same upfloat as two rounds before.
C.16
Minimize the score differences of players who receive the same downfloat as the previous round.
C.17
Minimize the score differences of players who receive the same upfloat as the previous round.
Rule C.04.1:e states that, in general, players should meet opponents with the same score. This is (of course) best achieved by pairing each player inside its own bracket. However, there are some situations, in which a player cannot be paired in its bracket - and then, by necessity, must float. These criteria limit the frequency with which such an event can happen to a same player
- but they are “very weak criteria”, in the sense that they are almost the last to be enforced - and almost the first to be ignored in case of need.
Here, each criterion establishing a certain protection for downfloaters is immediately followed by a similar one establishing the very same protection for upfloaters.
Because of this, there is a certain residual asymmetry in the treatment; viz. downfloaters are (just a little bit) more protected than upfloaters. Please note that, in some other Swiss systems, floaters’ opponents are not considered floaters themselves, and therefore enjoy no protection at all.
The four previous rules minimised the number of players who, having floated in the last two rounds, may get a float again in this round. However, those rules do not give any special protection either to a player who, being already a MDP in a bracket (in this round), cannot be paired and must float down again, or to its opponent. Such players, and their opponents, will
29 Of course, since the total number of disregarded preferences must remain the same (we cannot have it smaller, and do not want it to grow larger!), this may only happen at the expense of a same number of mild preferences. A brief example may shed some light on the matter. Consider the bracket {1Bb, 2b, 3Bb, 4b}, where we have x=2, but z=0. The latter means that we can build the pairs in such a way that any one of them contains no more than one strong colour preference – and, in fact, a simple transposition allows us to obtain just this result.
Minimize the score differences of players who
receive the same downfloat as two rounds before.
C.19
Minimize the score differences of players who receive the same upfloat as two rounds before.
The criteria C16-C.19 are for protected players whose protection has already failed once or more, and try to prevent such players from further floating. When we must make some players float down, we try, as long as possible, to choose those players who are not MDPs. Sometimes, however, this is not possible, and we must make some MDP float down. In this case, we should, as far as possible, choose those MDPs that are not (or are least) protected because of previous floats. Of course, the same holds (almost) symmetrically for the MDPs’ opponents.
For example, in a CLB (see A.9) that contains players with many different scores, the effect of these rules is that, if we have two possible prospective floaters and only one of them is protected, we try to pair the latter with a SD as little as possible30.
- Rules for the sequential generation of the pairings
This section states the rules to determine the sequence in which transpositions, exchanges, and MDP-exchanges must be tried, in order to generate the candidates in the correct order. The general basic principle is, as always, that of “minimal disturbance” of the pairing. This means that we have always to move that player (or those players) whose displacement will cause the least possible difference of the pairing from the “natural” one31 - while at the same time allowing the best possible quality of the pairing itself.
Before any transposition or exchange take place, all players in the bracket shall be tagged with consecutive in-bracket sequence-numbers (BSN for short) representing their respective ranking order (according to A.2) in the bracket (i.e. 1, 2, 3, 4,...).
-
- Transpositions in S2
A transposition is a change in the order of the BSNs (all representing resident players) in S2.
All the possible transpositions are sorted depending on the lexicographic value of their first N1 BSN(s), where N1 is the number of BSN(s) in S1 (the remaining BSN(s) of S2 are ignored in
The use of pairing-ids, in this phase, may sometimes be confusing. Therefore, we give temporary sequence numbers to the players, as a very handy remedy to simplify the application of the rules below.
All transpositions are sorted or compared based on the dictionary (“lexicographical”) order, so that one given transposition precedes or follows another one if the string formed by the players BSNs of the first one precedes or follows that of the second one. The method to compare the strings is the very same already illustrated for the comparison of PSDs 32.
30 Another example is the case of two MDPs with different scores, and a protected resident who must be paired with one of those two MDPs: the resident should be paired to the MDP who has the lower score of the two.
31 But, to avoid misunderstandings, we should keep in mind that any change in the order in S2 (transposition) is by definition preferable to even a single exchange between S1 and S2.
32 See the comment to C.6 [page 24] for details. Please note that the use of alphabet letters would be completely equivalent to that of numbers, at least for brackets with less than 26 players. The use of numbers, however, allows an identical treatment for all brackets, whatever the number of players they contain.
this context, because they represent players bound to constitute the remainder in case of a heterogeneous bracket; or bound to downfloat in case of a homogeneous bracket - e.g. in a 11- player homogeneous bracket, it is 6-7-8-9-10, 6- 7-8-9-11, 6-7-8-10-11,..., 6-11-10-9-8, 7-6-8-9-
10,..., 11-10-9-8-7 (720 transpositions); if the bracket is heterogeneous with two MDPs, it is: 3- 4, 3-5, 3-6,..., 3-11, 4-3, 4-5,..., 11-10 (72
transpositions)).
The subgroup S1 may or may not have the same number of players as S2. For the comparison to have a meaning, we must define the number of elements of each of the two strings of BSNs that we are comparing.
We are looking for mates for each element in S1 (which of course represent a player each). Thus, we consider the number N1 of elements in S1– while the remaining players are (for the moment) irrelevant.
A simple example will help us clarify the matter: consider a heterogeneous bracket {S1=[1]; S2=[2, 3, 4]}. All the possible transpositions of S2 (properly sorted, and including the original S2) are:
[2,3,4]; [2,4,3]; [3,2,4]; [3,4,2]; [4,2,3];
[4,3,2]33.
As we want to pair #1 with the first element of S2, it is at once apparent that [2,3,4] and [2,4,3] have the very same effect34; and the same holds for [3,2,4] and [3,4,2]; and for [4,2,3] and [4,3,2]. Hence, the actual sequence of transpositions is as follows (elements between braces “{…}” are ‘irrelevant’ and are ignored in this phase):
[2]{3, 4}; [3]{2, 4}; [4]{2, 3}
-
- Exchanges in homogeneous brackets or remainders (original S1 ↔ original S2)
An exchange in a homogeneous bracket (also called a resident-exchange) is a swap of two equally sized groups of BSN(s) (all representing resident players) between the original S1 and the original S2.
In order to sort all the possible resident- exchanges, apply the following comparison rules between two resident-exchanges in the specified order (i.e. if a rule does not discriminate between two exchanges, move to the next one).
The priority goes to the exchange having:
-
-
- the smallest number of exchanged BSN(s) (e.g. exchanging just one BSN is better than exchanging two of them).
- the smallest difference between the sum of the BSN(s) moved from the original S2
-
The exchanged sets must of course have the same size - because, were it not so, we would be changing the sizes of S1 and S2.
However, to evaluate the “weight” of the change, we must take into consideration not only the size of the exchanged sets but also the choice of players. To do that, we need a set of criteria addressing the various aspects of this choice. The aim is, as always, the “minimal disturbance” – viz. to try and have a pairing as similar as possible to the natural one.
The first criterion is, of course, the number of involved players: the less, the better!
From a theoretical point of view, all players in S1 should be stronger than any player in S2 is.
33 Please note that, in the very simple case where every BSN is a single digit, the string may be interpreted as a number, which becomes larger and larger as we proceed with each new transposition: 234, 243, 324, 342, 423, 432.
34 Of course, this equivalence is in no way general – it depends only on the fact that we are looking for just one element!
to S1 and the sum of the BSN(s) moved from the original S1 to S2 (e.g. in a bracket containing eleven players, exchanging 6 with 4 is better than exchanging 8 with 5; similarly exchanging 8+6 with 4+3 is better than exchanging 9+8 with 5+4; and so on).
-
-
- the highest different BSN among those moved from the original S1 to S2 (e.g. moving 5 from S1 to S2 is better than moving 4; similarly, 5-2 is better than 4-3; 5-4-1 is better than 5-3-2; and so on).
-
-
-
- the lowest different BSN among those moved from the original S2 to S1 (e.g. moving 6 from S2 to S1 is better than moving 7; similarly, 6-9 is better than 7-8; 6-7-10 is better than 6-8-9; and so on).
-
Therefore, when we have to swap two players across subgroups, we try to choose the weakest possible player in S1 and swap it with the strongest possible one from S2.
To do so, we can use the BSNs to choose a player as low-ranked as possible from S1, and a player as high-ranked as possible from S2, and then swap them, assuming that a higher rank should indicate a stronger player.
Thus, the difference between exchanged numbers is (or, at least, should be) a direct measure of the difference in (estimated) strength and should therefore be as little as possible.
When two possible choices of players to be exchanged show an identical difference in the sum of their respective BSNs, we choose the set which disturbs S1 as little as possible, i.e. the one in which the (highest BSN) player from S1 has a lower rank.
In the example, 5-2 is better than 4-3 because exchanging #5 is better than exchanging #4. Similarly, (5,4,1) is a better choice than (5,3,2), because exchanging #4 is better than exchanging #335.
Finally, having optimised the difference in ranking and the disturbance in S1, we can optimise the disturbance in S2 too.
Contrary to S1, now we try to exchange the lower possible BSNs. Hence, 6-9 is better than 7-8, because exchanging #6 is better than exchanging #7 – and so forth.
-
- Exchanges in heterogeneous brackets (original S1 ↔ original Limbo)
An exchange in a heterogeneous bracket (also called a MDP-exchange) is a swap of two equally sized groups of BSN(s) (all representing MDP(s)) between the original S1 and the original Limbo.
Here we are changing the composition of the set of pairable MDPs. Of course, this alteration may only occur when M1 < M036, because only in this situation does a Limbo exist. This means that we must choose which MDPs to exclude from the pairing. Sometimes the decision is easy – e.g. there may be some incompatible MDP, and we may have no choice at all37.
35 Sometimes, just as it happens in the above example, we might end up exchanging a higher-ranked player, as a side effect of enforcing the exchange of the lowest possible player. To understand this, we want to remember that, in the exchange, we do not operate on “several single players” but on a whole set of them, and we just have to decide if a set is better or worse than another one. In this case, (5, 4, 1) is better than (5, 3, 2) – therefore, we exchange #1, who is the top-player, because this is the way to exchange #4 rather than #3.
36 See B.1, p. 15.
37 We want to remember that, because of C.7, the downfloaters from the previous bracket (i.e. the MDPs of the current bracket) have already been optimised. Thus, if we have an incompatible here, it means that there was no alternative at all. Hence, there is no going back to the previous bracket (“backtracking”).
In order to sort all the possible MDP-exchanges, apply the following comparison rules between two MDP-exchanges in the specified order (i.e. if a rule does not discriminate between two exchanges, move to the next one) to the players that are in the new S1 after the exchange.
The priority goes to the exchange that yields a S1 having:
- the highest different score among the players represented by their BSN (this comes automatically in complying with the C.6 criterion, which says to minimize the PSD of a bracket).
- the lowest lexicographic value of the BSN(s) (sorted in ascending order)
Any time a sorting has been established, any application of the corresponding D.1, D.2 or D.3 rule, will pick the next element in the sorting order.
When we have a choice, we start by trying to pair as many MDPs as possible, and as high ranked as possible [B.2]. If we must change this original composition, we need to apply an MDP- exchange. The following criteria allow us to determine the priority among all the possible exchanges. Please note that this result is achieved by inspecting the composition of the new S1, not that of the Limbo. To a hasty reader, it might seem that, pairing a player with lower score would yield a lower score difference, and thus a lower PSD. Of course, this is definitely wrong! When we put a higher scored player in the Limbo, that player will float – hence, the corresponding SD, which is calculated with the artificial value defined in A.8, will be very high. To minimise the PSD, the Limbo must contain a minimum of players, and those must have as low a score as possible. Hence, complying with C.6, which instructs us to minimise the PSD, automatically satisfies this criterion too.
We also want to take notice that the number of exchanged players is not all-important. For example, consider an S1 with three players and a Limbo with two: in some circumstances, exchanging the two lower ranked players may give better results than exchanging just the top one.
This is the criterion we must strive to comply with. When the involved players have the same scores, we have to choose the lower ranked players. This is easily accomplished by comparing the BSNs of the players comprised in S1 after the exchange - in the very same way as we did in the previous cases.
If we are lucky enough, the first attempt to a transposition, exchange, or MDP-exchange will yield the desired result. Often, though, we must persevere in the attempts until we get a successful one. In this case, we must follow the order (sequence) established by the three rules above illustrated.
Ideally, we should start by establishing a full list of all the possible transformations - be them transpositions or exchanges of any kind - sorting that list by D.1, D.2 or D.3 (as the case may be), and then trying one after another until we find the first useful one38.
38 In common practice, exchanges and transpositions will be tried together (for each exchange, we will likely try one or more transpositions). To avoid mistakes, it is most advisable to annotate the last transformation (of each kind) used so that, on the following attempt, we can be sure about which element of the sequence is the next one.
- Colour Allocation rules
Initial-colour
It is the colour determined by drawing of lots before the pairing of the first round.
For each pair apply (with descending priority):
E.1
Grant both colour preferences.
E.2
Grant the stronger colour preference. If both are absolute (topscorers, see A.7) grant the wider colour difference (see A.6)
E.3
Taking into account C.04.2.D.5, alternate the colours to the most recent time in which one player had white and the other black.
E.4
The Initial-colour is not referred to any particular player. It is actually a parameter of the tournament – and the only one left to fate! – that allows the allocation of the correct colour to each player who has not a preference yet.
When two absolute preferences are involved, rule
-
- takes into consideration also the colour differences (see A.6) of the players. This, of course, may happen only for topscorers, and hence only in the last round (in previous rounds, a pairing with colliding absolute colour preferences is not allowed!). Let’s consider the example of two topscorers with the same absolute colour preferences and the following colour histories:
1: WWBWBW
2: BBWBWW
Here, player #1 has a colour difference CD=+2, while player #2 has CD=0. Thus, we try to equalize the colour differences by assigning to player #1 his preferred colour.
Please note that this rule applies only to pairs in which both players have an absolute preference, while in all other cases the rule does not apply – e.g., in the pair:
1: BWWBWBW (strong preference, CD=+1) 2: =BBWBWW (absolute preference, CD=0)
the absolute preference shall be satisfied, no matter how large the colour difference is.
To correctly manage colour assignments when one or both players have missed one or more games, we often need comparing colours histories by means of rule C.04.2:D.3.
For example, in the comparison between the colours histories of two players, the sequence
== WB is equivalent to BWWB and WBWB (but the latter two are not equivalent to each other!).
Grant the colour preference of the higher ranked player.
E.5 If the higher ranked player has an odd pairing number, give him the initial-colour; otherwise give him the opposite colour.
Note: Always consider sections C.04.2.B/C (Initial Order/Late Entries) for the proper management of the pairing numbers.
We may want to pay particular attention to this point: in all other conditions being equal, the higher ranked player gets not white but its own preferred colour!
When we get here, both players of the pair have no colour preference. Therefore, we use the Initial-colour decided by lot before the start of the tournament, to allocate colours to the players.
Of course, this rule will be used always in the first round (obtaining the usual results39), but it will be useful also in subsequent rounds, when we have a pairing between two players who did not play in the previous rounds (e.g. late entries or forfeits).
We ought to remember that players, who are actually entering the tournament only at a given round after the first – and who therefore were not paired in the previous rounds – in fact, do not exist, even if (seemingly) listed in the players’ list. An obvious side effect of this is that we cannot expect all “odd-numbered” and “even- numbered” players to have the same colour as would be usual (viz., as they would have in a “perfect” tournament).
Actually, such late entries may have different effects on the pairing numbers, depending on how they are managed.
If we insert all the players in the list straight from the beginning, the pairing numbers will not change on the subsequent rounds, but the pairing of the first round will have to “skip” the absent players. For example, if player #12 is not going to play on the first round, players #13, #15, and so forth, who should seemingly get the initial- colour, will actually have the opposite colour; while players #14, #16, and so on will get the initial-colour.
If, on the contrary, we insert a new player only when it actually enters the tournament, we must find the correct place to put it. All the subsequent players will therefore have their pairing numbers changed, in order to accommodate the new entry. For example, if the newly inserted player gets #12, the previous #12 (who had colour opposite to the initial-colour) will now be #13; and so on for all subsequent players.
39 Please note that, if we are using an accelerated pairing system, the usual colour alternation is disrupted unless the first score group contains a number of players multiple of four.
PART TWO – THE TOURNAMENT
-
-
- FOREWORD
-
This chapter illustrates a step-by-step example of pairing procedure for a six rounds Swiss tournament by means of the FIDE (Dutch40) Swiss pairing system, in the hope to help those who wish to improve their knowledge of the system or get more familiar with it.
During the FIDE Congress in Abu Dhabi 2015, the Swiss Rules for the FIDE (Dutch) system were partially modified and reworded in order both to avoid misunderstanding in some points and to correct some peculiar behaviours in particular situations - and thus get better pairings in some instances41. In the following Congress in Baku 2016, the Swiss Rules were completely reworded, with the aim to make them clearer and easier – but this time without introducing behavioural changes.
Only a general knowledge of the FIDE (Dutch) system is required to follow the exercise, but keeping a handy copy of the Rules is advisable.
Before ending this short introduction, two side notes about language are in order: first, this work has not been intended for, nor written by, native speakers
- hence, the language is far from perfect, but we hope that it will be easy enough to understand, and that any possible native speakers will forgive its many flaws. Second, and possibly more important, is that we definitely do not want to address a player as either man or woman. Luckily, English language offers a very good device to this end in the use of neutral pronouns - therefore, our readers are advised that our player will always be “it”.
Warm and heartfelt thanks go to IA Roberto Ricca for his valuable and patient work of technical review and the many useful suggestions.
Happy reading!
Notice: to help the reader, the text contains many references to relevant regulations. These references are printed in italics in square brackets “[ ]” - e.g., [C.04.2:B.1] refers to the FIDE Handbook, Book C: “General Rules and Recommendation for Tournaments”, Regulations 04:
40 The FIDE (Dutch) Swiss pairing system, so named with reference to its promoter and developer, Dutch IA Geurt Gijssen, was adopted by FIDE in 1992. Its rules are codified in the FIDE Handbook, available on www.fide.com.
41 For details about the changes, see the minutes of Abu Dhabi 2015 Congress in the Swiss Systems of Pairings and Programs Commission webpage, http://pairings.fide.com.
“FIDE Swiss Rules”, Section 2: “General Handling Rules”, item (B), paragraph (1). Since a great deal of our references will be made to section C.04.3: “FIDE (Dutch) System”, these will simply point to the concerned article or subsection - e.g., [A.7.b] indicates item (b) of Article
(7) of section (A) of those Rules. All regulations can be downloaded from the website of FIDE (www.fide.com).
-
-
- INITIAL PREPARATIONS
-
The preliminary stage of a tournament consists essentially in the preparation of the list of participants. To this end, we sort all players in descending order of score42, FIDE rating and FIDE title43 [C.04.2:B]. Homologous players (i.e. those players who have identical scores, ratings and titles) will normally be sorted alphabetically, unless the regulations of the tournament or event explicitly provide a different sort rule.
 Here we face our first problem: the FIDE (Dutch) system belongs to the group of rating controlled Swiss systems44, which means that the resulting pairings depend very closely on the rating of the players - therefore, to get a proper pairing for the round, the players’ ratings need to be the correct ones – i.e. they must correctly represent each player’s strength. Because of this, the Rules require us to carefully verify all of the ratings and, when a player does not have one, to make an estimation as accurate as possible [C.04.2:B.2]. When a player has a national rating, but no FIDE rating, we can convert the first to an equivalent value - in some cases directly, in others by using appropriate formulas. For instance, when a player has no rating at all, we shall usually need to estimate its strength according to current practices or national regulations.
Here we face our first problem: the FIDE (Dutch) system belongs to the group of rating controlled Swiss systems44, which means that the resulting pairings depend very closely on the rating of the players - therefore, to get a proper pairing for the round, the players’ ratings need to be the correct ones – i.e. they must correctly represent each player’s strength. Because of this, the Rules require us to carefully verify all of the ratings and, when a player does not have one, to make an estimation as accurate as possible [C.04.2:B.2]. When a player has a national rating, but no FIDE rating, we can convert the first to an equivalent value - in some cases directly, in others by using appropriate formulas. For instance, when a player has no rating at all, we shall usually need to estimate its strength according to current practices or national regulations.
42 Of course, at the beginning of the tournament all players have a null score, unless an accelerated pairing is used.
43 The descending order for FIDE titles is GM, IM, WGM, FM, WIM, CM, WFM and WCM - followed by all untitled players [C.04.2:B.3.b].
44 The “Rating Controlled Swiss Systems” belong to a more general class of “Controlled (or Seeded) Swiss Systems”, in which the initial ranking list is not random or assigned by lots, but sorted according to given rules.
After we prepared the list as indicated above, we can assign to each player its
|
Pairing Number |
Player |
Title |
Rating |
|
1 |
Alice |
GM |
2500 |
|
2 |
Bruno |
IM |
2500 |
|
3 |
Carla |
WGM |
2400 |
|
4 |
David |
FM |
2400 |
|
5 |
Eloise |
WIM |
2350 |
|
6 |
Finn |
FM |
2300 |
|
7 |
Giorgia |
FM |
2250 |
|
8 |
Kevin |
FM |
2250 |
|
9 |
Louise |
WIM |
2150 |
|
10 |
Marco |
CM |
2150 |
|
11 |
Nancy |
WFM |
2100 |
|
12 |
Oskar |
-- |
2100 |
|
13 |
Patricia |
-- |
2050 |
|
14 |
Robert |
-- |
2000 |
Our tournament is comprised of 14 players. The players’ list, already properly sorted according to [C.04.2:B], is on the right.
Because of a perhaps a bit controversial (but none the less almost universal) language convention, players who are first on this list (“higher ranked” players) are said to have the highest pairing numbers - in short, number 1 is higher than 14... This is somewhat odd – but, in time, it will become a habit.
The number of rounds is established by the tournament regulations, and cannot be changed after the tournament has started. We may want to notice that this number is, or should be, in close relation with the number of players, because a Swiss tournament can reasonably identify the winner only if the number N of players is less than or at most equal to 2 raised to the number T of rounds: N ≤ 2T. As a rule of thumb, each additional round enables us to correctly determine one more position in the final standings. For example, with 7 rounds we can determine the strongest player (and, therefore, the player who deserves to win) among at most 128 players while we will be able to correctly select the second best among only 64 players, and the third best only if the players are at most 3246. Thus, it is generally advisable to carry out one or two rounds more than the theoretical minimum: e.g., for a tournament with 50 players, 8 rounds are adequate, 7 are acceptable - while, strictly speaking, a 6 rounds tournament
45 Sometimes a player may be registered with a wrong rating, which needs to be corrected (this is necessary for rating purposes too). In such cases, the pairing numbers may be reassigned, but only for the first three rounds; from the fourth round on, pairing numbers shall not be changed, even if players’ data have to be adjusted [C.04.2:B.4].
46 This is true only if in every game the highest rated player ends up as winner. In practice, the occurrence of different results, such ad draws, forfeits and so on, may change the situation, making the individuation of a definite winner (the so called “convergence”) either slower or faster, according to specific circumstances.
(which are the “bare minimum” with respect to the number of players) would not be advisable47.
The preliminary stage ends with the possible preparation of “pairing cards”, a very useful aid for the management of a manual pairing. They are sort of a personal card, the heading of which contains player’s personal data (name, date and place of birth, ID, title, rating and possibly additional useful data) and of course the pairing number of the player. The body of the card is comprised of a set of rows, one for each round to be played, in which all pairing data are recorded (opponent, colour,
float status48, game result or scored points, progressive points). The card may
be made in any of several ways, as long as it is easy to read and to use. Here, we see a typical example.
The basic advantage of pairing cards is that we can arrange them on the desk, sorting them by rank, rearranging and pairing them in an easy and fast fashion. Nowadays, anyway, actual use of pairing cards has become pretty rare because an arbiter is very seldom required to manually make a pairing from scratch - but it’s not unusual that an unhappy player asks for detailed explanations, so that the arbiter has to justify an already made pairing (usually produced by computer software). With a little practice, we can work out such an explanation right from the tournament board - which, in this case, needs to contain all of the necessary data, just like a pairing card. In this paper, we too will follow this latter method.
47 Of course, this is just a theoretical point of view. In practice, many tournaments are comprised of 5 rounds, because sometimes this is the best we can put together in a weekend. Thus, the determination of the players who end up in the winning positions of the final standings must be entrusted to tiebreaks, which should therefore be chosen with the utmost care.
48 See “Scoregroups and brackets”, page 40.
Now we will draw by lot the Initial-colour49 [see section E]. The colours to assign for the first round to all players will be determined by this [A.6.d, E.5]. After that, we will be ready at last to begin the pairing of the first round. Let us say that a little child, not involved in the tournament, drew White as Initial- colour.
-
-
- THE MAKING OF THE FIRST ROUND
-
S1 = [ 1, 2, 3, 4, 5, 6, 7]
S2 = [ 8, 9, 10, 11, 12, 13, 14] 51
Now, we pair the first player from S1 with the first one from S2, the second one from S1 with the second one from S2 and so on, thus getting the (unordered) pairs {1-8, 2-9, 3-10, 4-11, 5-12, 6-13, 7-14}. Since this is the first round, unless there is some very special reason to do differently52, there is nothing to stop these pairings – so, to complete the pairing process, now we just need to assign to each player its appropriate colour.
Since no player has a colour preference yet, all colour allocation shall be regulated per [E.5]. Hence, in each pair, the higher ranked player (who comes from S1) gets the initial-colour if its pairing number is odd, while it gets the opposite colour if the pairing number is even. Thus, players 1, 3, 5 and 7 shall receive the initial-colour, for which we drawn white, while players 2, 4, 6 shall receive the opposite, which is black.
49 Some arbiters, misinterpreting the drawing of lots, assign colour at own discretion. It should be emphasized that the Rules explicitly require the drawing of lots (which, by the way, may be at the centre of a nice opening ceremony).
50 When the number of players is odd, S2 will contain one player more than S1.
51 Since names are inessential, from now on we will indicate players only by their own pairing numbers.
52 For example, in certain events, we might have specific rules, or reasons, to avoid players or teams from the same federation or club meet in the first round(s), or at all. Such cases usually occur only in major international tournaments, championships, Olympiads and so on, while in “normal” tournaments, in practice, nothing of the kind happens.
The opponents to each player from S1 shall receive, out of necessity, the opposite colour with respect to their opponents; therefore, the complete pairing will be:
1 : 1 - 8
2 : 9 - 2
3 : 3 - 10
3 : 3 - 10
4 : 11 - 4
5 : 5 - 12
6 : 13 - 6
7 : 7 - 14
Before publishing the pairing, we have to put it in order [C.04.2:D.9] with the following criteria: 1) the score of the higher ranked player in the pair, 2) the sum of scores of both players, 3) the rank according to the initial order [C.04.2:B] of the higher ranked player. In the vast majority of cases, the FIDE (Dutch) system already generates pairings in the right order (but we always want to check).
At last, we are almost ready to publish the pairing. Before that, we want to check it once again and with extreme care, because a published pairing should not be modified [C.04.2:D.10]53, except when two players should play with each other again.
In the event of an error (wrong result, game played with wrong colours, wrong ratings…), the correction will affect only the pairings yet to be done54 and only if the error is reported by the end of the next round, after which it will be taken into account only for the purposes of rating calculation [C.04.2:D.8]. This means that, in such cases, the final standings will include the wrong result, just as if it were correct!
The last thing to do (and the Pairings Controller may do it while everyone is playing) is the compilation of the tournament board, on which we will post pairings and results for each player. When we renounce the use of pairing cards, as we do here, the board should also contain any other relevant information needed to compose the pairings for following rounds.
For each game, we should indicate at least opponent, assigned colour, and result
– the choice of symbols is free, as long as it is clear, unambiguous, and uniform.
53 But, in this regard, see also FIDE Handbook C.05: “FIDE Competition Rules”, item 7.4.
54 Please note that this rule explicitly forbids the making of new pairings – which is a somewhat frequent request of players - in the event of an error.
Here we will show each pairing by means of a group of symbols comprised of the opponent’s pairing number, followed by a letter indicating the assigned colour (B for “Black”, “W” for “White”); next, we can have some optional “utility” symbols, and finally the result (“+”, “=“ or “-”, with obvious meaning).
Unplayed games are indicated in different ways, depending on their nature: a “PAB” indicates a Pairing-Allocated Bye, while “HPB” (Half Point Bye) or “ZPB” (Zero Point Bye) indicate an announced leave. In case of a forfeit (viz. a game that was scheduled but not played), we will use “+000” for the player who is present and “-000” for the absent one. Since we do not make use of pairing cards, our board will also show the players’ progressive scores, which help us in the preparation of pairings (and of intermediate standings too).
After collecting the results of all the games, we can proceed to the pairing of the next round.
| 1 | 1 (0.0) - 8 (0.0) | 1-0 |
| 2 | 9 (0.0) - 2 (0.0) | 0-1 |
| 3 | 3 (0.0) - 10 (0.0) | 1-0 |
| 4 | 11 (0.0) - 4 (0.0) | ½-½ |
| 5 | 5 (0.0) - 12 (0.0) | 1-0 |
| 6 | 13 (0.0) - 6 (0.0) | 0-1 |
| 7 | 7 (0.0) - 14 (0.0) | 1-0 |
-
-
- SECOND ROUND (BYES, TRANSPOSITIONS AND FLOATERS)
-
Here is the tournament board after the first round:
| Player | PN | 1 | 2 | 3 | 4 | 5 | 6 | ||||||
| Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | ||
| Alice | 1 | 8W+ | 1 |
|
|
|
|
|
|
|
|
|
|
| Bruno | 2 | 9B+ | 1 |
|
|
|
|
|
|
|
|
|
|
| Carla | 3 | 10W+ | 1 |
|
|
|
|
|
|
|
|
|
|
| David | 4 | 11B= | 0.5 |
|
|
|
|
|
|
|
|
|
|
| Eloise | 5 | 12W+ | 1 |
|
|
|
|
|
|
|
|
|
|
| Finn | 6 | 13B+ | 1 |
|
|
|
|
|
|
|
|
|
|
| Giorgia | 7 | 14W+ | 1 |
|
|
|
|
|
|
|
|
|
|
| Kevin | 8 | 1B- | 0 |
|
|
|
|
|
|
|
|
|
|
| Louise | 9 | 2W- | 0 |
|
|
|
|
|
|
|
|
|
|
| Mark | 10 | 3B- | 0 |
|
|
|
|
|
|
|
|
|
|
| Nancy | 11 | 4W= | 0.5 |
|
|
|
|
|
|
|
|
|
|
| Oskar | 12 | 5B- | 0 | ZPB |
|
|
|
|
|
|
|
|
|
| Patricia | 13 | 6W- | 0 |
|
|
|
|
|
|
|
|
|
|
| Robert | 14 | 7B- | 0 |
|
|
|
|
|
|
|
|
|
|
Player #12 (Oskar) informed us in advance that he will not be able to play the second round, thus he shall not be paired [C.04.2:D.4] and shall score zero
points55: hence, we already posted a “ZPB” in the tournament board. In this round we will then have an odd number of players - hence, a player will end up unpaired and receive a “pairing allocated bye” (PAB): one point56, no opponent, no colour [A.5, C.04.1:c].
Now players have different scores, and a basic principle of all Swiss pairing systems is that paired players shall have scores as similar as possible [C.04.1:e]. To achieve this result, we shall sort the players according to their scores. To this end, we define the scoregroup, which normally is a set of players who, in a given round, have identical scores57 [A.3].
A score group is the main component of a (pairing) bracket, which is a set of players to be paired among them. A bracket that contains just a scoregroup (i.e., all players in it have the same score) is called homogeneous. The pairing usually proceeds towards decreasing scores, one score group at a time, from the upper one (i.e. the one corresponding to the maximum score) to the lower one (corresponding to the minimum score) – hence, the first task in pairing a round is to divide the players into scoregroups.
In practice, it happens rather frequently that one or more players in a bracket cannot be paired within their own same bracket. Those players shall be moved down, to join the next scoregroup; therefore, in this bracket, they are called downfloaters.
The next scoregroup and those moved players, together form the next bracket, which contains players with different scores – and is thus called a heterogeneous bracket.
Such brackets will have to be treated somewhat differently from homogeneous brackets, because some players will meet opponents with different scores, usually called “floaters”. A player moved down from the previous bracket (thus
55 Please note that the Tournament regulations may provide for a different score [C.04.2:D.4].
56 The usual score for a player who gets the PAB is that of a win (most commonly, one point). However, the Tournament regulations may provide for a different score.
57 There is a particular situation in which we define the “Special Collapsed Scoregroup” (SCS for short), which is a scoregroup containing players with different scores [A.9]. We will not discuss SCSs now, but we will encounter such scoregroups later in the tournament.
having a higher score) is called a moved-down player (MDP for short) [A.4], while its opponent is usually said to be an “upfloater”.
First, we divide and group players according to their score, thus forming the various scoregroups [A.3]. Then, as already mentioned, those scoregroups will be processed (“paired”) one by one. We always begin with the topmost scoregroup, containing the highest ranked players; in this round, they have scored one point: they are {1, 2, 3, 5, 6, 7}. The next scoregroups, formed by the players who scored half point, contains {4, 11}. Last, we have the scoregroup of the players who scored zero58: {8, 9, 10, 13, 14}.
Pairing parameters
Now, it is time to begin the real pairing. Since this is our first time, we will perform a detailed, systematic process. Then, as we proceed in the tournament, we will cut a little short on the more mundane tasks, to dwell only on the more interesting ones.
Let us start with the first bracket, which is constituted by the first scoregroup. The first step is determining some important parameters of the bracket [B.1]. The first one is the number M0 of moved-down players, and it is very easy: since this is the very first bracket, there can be no MDPs at all – therefore, M0=0. Since there are no MDPs to be paired, also M1=0.
The second parameter is MaxPairs, or the number of pairs to be built in the bracket. There is no straightforward method to determine this number – actually, we must “divine” it – but an “educated guess” usually allows us to estimate it in a reasonably precise and reliable manner.
The first thing to consider is that the number of pairs cannot be larger than half the number of players, which is therefore an “absolute theoretical maximum” to the pairs we can build. The actual number of pairs may however be less than that, because of several reasons:
58 Please note that this scoregroup does not contain #12, who shall not be paired (because of its announced absence).
- there may be some players who, for whatever reason, cannot play with any other player in the bracket59. Such players are called incompatibles60 (here, there is none).
- Sometimes it happens that in a bracket there are certain players who “compete” for the same opponent(s), in such a way that any, but not all, of them can be paired61. This situation is usually called “semi– (in)compatibility”, or “island-compatibility”.
- in some circumstances, the next bracket may require some floaters to be moved down to it, to make the pairing possible at all. We will come back to this problem later on.
In this bracket, the task is fairly easy: there are six players, and no incompatibles of any sort (it is too early in the tournament). We can thus safely guess that we will be able to build three pairs.
Colour preferences
Each player who played at least one game has a colour preference (or expected colour). To determine it, we need first to define the colour difference CD. This is simply the difference between the number W of actually played rounds in which the player had the white, and the number B of those in which it had the black: CD = W - B [A.6]. This difference is positive for a player who had more often white, negative if it had more often black – while it is zero if the colours are balanced. The latter is, of course, the ideal situation, and the pairing shall try to comply with it as much as possible.
The colour preference is determined as follows:
- A player has an absolute colour preference [A.6.a] when CD > 1 or CD < - 1 – that is, when it had a colour (at least) twice more than the other one –
59 There is no way to pair such a player in the bracket - therefore, the player can’t help but go away, which means float to the next bracket.
60 In a second round, anyone may still play with almost everyone else... hence, we usually can’t have incompatible players – except when special circumstances arise, such as those already mentioned (see note 52, page 37).
61 For example, consider the bracket {1, 2, 3, 4} in which players #1, #2, and #3 can all play only with player #4. No player here is incompatible: we can pair any one among them, but we cannot pair them all.
or when it had the same colour for two games in a row62. The preference is towards the colour that it received fewer times, or respectively the colour that it did not receive in the last two games. In any case, the player must receive its due colour (and we shall write it right away on the pairing card or on the tournament board). The only exception may happen in the last round, for a player with more than half of the maximum possible score (this is called a “topscorer”, see [A.7]) or its opponent [C.3]: in this case, indeed, top ranking positions may be at stake, and pairing players of equal scores is therefore particularly important. In all other cases, the colour preference shall be honoured, period. It is an absolute criterion and, in order to obey it, players may float as necessary.
- A player has a strong colour preference [A.6.b] when CD = ±1 (i.e. when it had a colour once more than the opposite), the preference being of course for the colour it received fewer times [C.04.1.h.1].
- If CD = 0, the player has a mild colour preference [A.6.c] for the colour opposite to what it had in the previous game, so as to alternate colour in its history63 [C.04.1.h.2].
- Finally, a participant, who did not play any games yet (a “late entry”, or a player who received a PAB in the first round, or was involved in a forfeited game), has no colour preference at all [A.6.d] and receives the colour opposite to that awarded to its opponent.
Strong and mild colour preferences may be disregarded, when necessary, so that the player might also get the colour opposite to its preferred one. In all such cases, however, this player gains an absolute colour preference for the next round.
During the pairing process, we need to keep colour preferences for each player handy. To avoid the use of yet another table, we will temporarily record all colour preferences in the tournament board, in the column bound to the pairing
62 Please note that we always refer only to actually played games: the colour allocated for a game that was forfeited, is irrelevant, and shall be ignored.
63 The “colour history” of a player is the sequence of colours it received in the previous rounds.
for the round (when it is time to post the pairings, we will not need the preferences any more).
Now, we want to establish a code to indicate the various kinds of colour preferences64:
- A lower case “w” or “b” indicates a mild colour preference
- A couple “Ww” or “Bb” indicates a strong colour preference
- A capital “W” or “B” indicates an absolute colour preference
- A capital “A” indicates a player who has no colour preference (as it happens, we have no such players in this tournament).
We should now determine the colour preference for each player, and we do that by examining the colour history in all previous actually played games of the player.
The round we are going to pair is an even numbered one. Hence, any participant who did not miss any games played an odd number of them, thus obtaining a strong colour preference (this early in the tournament, we cannot have absolute colour preferences yet). In the bracket, we will indicate such preferences by means of the above defined symbols, putting them right after the player’s pairing number: {1Bb, 2Ww, 3Bb, 5Bb, 6Ww, 7Bb}.
We already estimated that MaxPairs (or the maximum number of pairs) for this bracket is three. Now we want to check how many of these pairs cannot fully satisfy the colour preferences: here, two players expect white and four expect black – out of the three pairs, at least one will necessarily include two players who both expect black – and therefore one player who shall receive a colour different from its preference. The minimum number of pairs that shall contain a disregarded preference is usually called x. In a perfect pairing, the number of disregarded colour preferences will be exactly x. We can compute this number easily enough by taking the integer part of half the difference between the number of players expecting white and the number of players who expect black
- any number of players without any preference would be counted as having the
64 Please note that this code is far from universal and other papers may use completely different codes.
same preference of the minority (but we have no such players here). Out of necessity, we will consider any pairing that contains x pairs with disregarded preferences as perfect (having less than that is simply impossible). However, we will not accept any pairing that contains more than that – unless, of course, when this is unavoidable [C.10].
In the present instance, we have W=2, B=4, A=0 (all players have a preference)
- hence, x = (4-2-0)/2 = 1, which means that, as anticipated above, at least one pair must contain a disregarded colour preference. This disregarded preference will of course be one among the most numerous – that is, for black, which is here called the “majority colour”.
Another parameter we want to know is the minimum number z of pairs in which it will be necessary to disregard a strong colour preference. This number will help us in the process of optimization, which requires that, if we have to disregard a colour preference, it should be a mild one rather than a strong one65. This number is obtained by subtracting from x the number of players with mild preferences for the colour of the majority.
However, if, apart from the absolute preferences, in a bracket we have only strong ones, or only mild ones, this parameter is useless – just as criterion C.11 is – and we may omit them both.
In the current bracket, we shall have to disregard at least one (black) strong preference.
Preparation of the candidate
Now we can divide the players of the bracket between subsets S1 and S2 [B.2]. We put into S1 the first MaxPairs players of the bracket (in this case the first half of the players), while the rest (namely the second half) ends up in S2:
S1 = [1Bb, 2Ww, 3Bb] S2 = [5Bb, 6Ww, 7Bb]
Then we sort each of the subgroups according to the usual rules [A.2]. This order normally coincides with the original one, and so there is no need to do
65 Of course, absolute colour preferences can never be disregarded – the only possible exception being for topscorers or their opponents, during last round.
anything unless we got to this point after an exchange66 of players between S1 and S2.
S1 - 1Bb 2Ww 3Bb
S2 - 5Bb 6Ww 7Bb
So far, we only performed the necessary preliminary steps – now we are ready to prepare a candidate, which is a tentative pairing built as explained in [B.3]. To build it, we associate the first player of S1 with the first player of S2, the second player of S1 with the second player of S2, and so on, just as we did for the first round, thus obtaining the (tentative) pairs:
Evaluation of the candidate
Now, having built a candidate, we proceed to evaluate it [B.4]. First, we must check it for compliance with the absolute criteria C.1 (players who already met) and C.3 (clashing absolute colour preferences). Criterion C.2 does not apply to this bracket, because it is not the last one, so we do not have to allocate a PAB here. Criterion C.4 does not apply because this is an ordinary bracket and not a PPB. A candidate, which, as this one, complies with all the (relevant) absolute criteria, is said to be legal and may be evaluated for quality. A candidate that is not legal is instead immediately discarded.
Now that we are sure that the absolute criteria are obeyed, we must evaluate the compliance of the candidate with quality criteria C.5 – C.19. This compliance is measured by means of a series of failure values. Those are numerical values that describe “how good” the pairing is – the lower the failure value is, the better is the candidate.
Of course, as we will presently realize, not all criteria need to be considered in any situation – in many instances, some of them are simply irrelevant67. Therefore, we usually limit our attention only to the significant ones. However,
66 We will first meet exchanges during the pairing of the third round (see page 54).
67 For example, criteria C.8 and C.9 concern only topscorers and their opponents – hence, they apply only in the last round of the tournament, and only to some brackets, thus being completely irrelevant in most situations.
since this is the very first time we pair a non-trivial bracket, let us briefly examine them all, one by one.
To synthesise the global quality of the candidate, we build a simple table in which we will accommodate the failure values of the bracket for each one of the criteria, obtaining a sort of “report card” for the candidate.
| C. 5 | C. 6 | C. 7 | C. 8 | C. 9 | C.1 0 | C.1 1 | C.1 2 | C.1 3 | C.1 4 | C.1 5 | C.1 6 | C.1 7 | C.1 8 | C.1 9 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
We must now fill our report card with the failure values; let us then examine the candidate with reference to each criterion, and determine the respective failure values.
[C.5] maximises the number of paired players – or, (almost) equivalently, minimises the number of downfloaters. The simplest (and most obvious) choice for the failure value here is the number of players that we cannot pair68. In our example, we need to build three pairs and we are going to make all of them – since we are making all the possible pairs, the failure value is zero.
[C.6] minimises the overall difference of scores between paired players (PSD, see A.8). This very important parameter is always zero in any candidate pairing of a homogeneous bracket, because all players have by definition identical scores (actually, trying to apply that criterion in a homogeneous bracket makes no sense at all). In fact, this criterion only applies for heterogeneous brackets, where minimising the PSD is practically equivalent to pair as many MDPs as possible, and the most natural failure value is the PSD itself. However, we will let the matter be for the time being, to be back there later on.
[C.7] chooses the best downfloaters, viz. those that best pair the next bracket, maximising the number of pairs and minimising the
68 Please note that the choice of the failure value for a criterion is largely arbitrary, in that it may be any of a (infinite) number of functions (in the mathematical sense of the word) – but the simplest and more natural choice is of course the number of failures (unpaired players, disregarded colour preferences and so on).
[C.8], [C.9] minimise the numbers of topscorers, or topscorers’ opponents, who get a colour difference with an absolute value higher than 2 [C.8]; or who get the same colour three times in a row [C.9]. These criteria only apply when pairing the last round, and only in processing those brackets that actually contain topscorers. The failure values would be the numbers of topscorers, or topscorers’ opponents, mentioned above. In the present bracket, both those numbers are zero, as there are no topscorers at all (this is not the last round!).
[C.10] minimises the number of players whose colour preference is disregarded. The failure value for this criterion is the number of players who do not get their colour preference. The minimum number of colour preferences that shall unavoidably be disregarded in a bracket is x, and we already know that this number is not necessarily zero. Therefore, to determine if the present candidate is a perfect one [B.4], we must compare the number of colour preferences actually disregard in the candidate against the number x, for which we found a value x=169. A direct inspection of the candidate shows that three out of three pairs contain one disregarded colour preference – hence, the failure value for this criterion is 3 and, since the reference value is 1, this candidate is not perfect.
[C.11] minimises the number of players whose strong colour preference is disregarded. The failure value for this criterion is the number of players who do not get their strong colour preference, and the
69 See the computation of x for this bracket in “Colour preferences” (page 43).
minimum for this number is z. All the considerations just made about C.10 also apply in respect to this criterion70.
[C.12]
… [C.15]
minimise the number of players who, having floated in the last two rounds, float again in this round. The failure value for each one of these four criteria is the number of floaters of the two kinds that float again. Since this bracket contains no floaters, all the failure values are zero. For the time being, we will not discuss those criteria any further – but we will go back to the matter later on.
[C.16]
… [C.19]
Minimise the score difference of players who receive a same float as they already got in the last two rounds. For these criteria, we will use as failure values the SDs of the involved players. As mentioned above, in the current bracket there are no floaters – hence, the failure values are all zero.
Now we can synthesise the global quality of the candidate, filling in the failure values of the bracket for each one of the criteria into the report card.
| C. 5 | C. 6 | C. 7 | C. 8 | C. 9 | C.1 0 | C.1 1 | C.1 2 | C.1 3 | C.1 4 | C.1 5 | C.1 6 | C.1 7 | C.1 8 | C.1 9 |
| 0 | 0 | 0/0 | 0 | 0 | 3 | 3 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
Looking for a better candidate: transpositions
As we mentioned above, this candidate is not a perfect one, because the number of disregarded colour preferences is larger than the bare minimum (x). We must then take some action to obtain a different candidate, looking for a better one. The process is simple enough: we alter the subgroups S2 and (if needed) S1 looking for a perfect, or at least better, candidate [B.5]. After each alteration, we must build and evaluate a new candidate, just in the same way as we did it above.
Since this is a homogeneous bracket, we are directed to rule B.6, which instructs us to first apply a transposition of S2. We will try all the possible transpositions,
70 Please note that, in the current bracket, the colour preferences are all strong, and therefore criterion
C.11 is useless and may be ignored. However, since this is our first example, we will consider it all the same.
one by one, until we find the first one among them that gives a perfect pairing. Only if that procedure fails to yield an acceptable result, we will also apply one or more exchanges between S1 and S2 – and, for each exchange, we will look for the first useful transposition as described above.
The first step is thus to try to alter the subgroup S2 applying a transposition, to see if we can reach the goal. A transposition changes the order of the players in S2, starting with the lowest ranked players and then gradually moving towards higher ranks - until an acceptable solution is found. The method to do that is explained in section D, which gives the rules for the sequential generation of candidates.
Before applying any transposition or exchange, each player is temporarily labelled with a number, representing the player’s ranking order in the bracket. This number is called “In-Bracket Sequence Number”, BSN for short. The BSNs will help us to keep track of the transformations we are going to apply to the subgroups, as they never change when we transpose or exchange players. In our bracket, there are six players, who shall be labelled with numbers from 1 to 671:
| player | 1Bb | 2Ww | 3Bb | 5Bb | 6Ww | 7Bb |
| BSN | 1 | 2 | 3 | 4 | 5 | 6 |
We are going to apply a transposition, which involves only players in S2, viz.
{4, 5, 6}. The transpositions of the bracket are represented by all the possible dispositions of those three numbers, sorted in lexicographic order – which in practice means to arrange in ascending order all numbers that can be constructed with these figures (in our case: 456, 465, 546, 564, 645, 654) [D.1]. The first of those transpositions always corresponds with the basic order of the subgroup, which was used in the first pairing attempt. Therefore, we now start trying from the second transposition on, which is 465, or [5Bb, 7Bb, 6Ww]:
71 Of course we could also choose any other set of numbers (or, why not, hexadecimal figures, letters of the alphabet, random words…), as long as they form an arithmetical progression (a sequence of equally spaced numbers in strict ascending order) – in this case, the rules just indicate the easiest possible choice.
In this candidate pairing, the pair 1-5 does not meet all of the colour preferences, while the subsequent 2-7 and 3-6 do. Hence, the failure values for C.10 and C.11 are now both 1. Since we already know that (at least) one pair shall disregard a colour preference, this candidate is perfect, and we accept it72. The pairs formed are hence [(1,5), (2,7), (3,6)]. Colours to be assigned to each player remain yet to be defined – we will do that only after the pairing of all players is complete.
Now, this latter candidate is the first perfect one that we found, and there can be no better and earlier candidate than this one, which is therefore immediately accepted. Hence, there is no need to compare it with the previous one. However, we will do that all the same, just as a useful exercise in evaluating the candidate. First, we prepare the report card, and put it side-to-side with that of the previous candidate:
|
|
C. 5 | C. 6 | C.7 | C. 8 | C. 9 | C.1 0 | C.1 1 | C.1 2 | C.1 3 | C.1 4 | C.1 5 | C.1 6 | C.1 7 | C.1 8 | C.1 9 |
| new | 0 | 0 | 0/0 | 0 | 0 | 1 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| old | 0 | 0 | 0/> 0 | 0 | 0 | 3 | 3 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
Now, to compare the candidates, we start from the first failure value (C.5) and compare them: if one of the two is smaller than the other one, that one of course relates to the better candidate, and the comparison process stops here. As long as the failure values are identical, the candidates are still “equivalent” and we proceed downwards to the next failure value (C.6) – then to C.7 and so forth, always operating in the same way, possibly until the end (C.19). If, in the end, there were no differences at all, the candidates are actually equivalent (from the point of view of the quality of pairing) and therefore we must choose the one which was produced first of the two in the sequence of generation.
This kind of complete comparison is of course of great theoretical value.
72 It is worth noting that, since we choose the first useful transposition, it is possible (and even statistically likely) that the pairs in which we find the disregarded colour preferences are formed at the top of the bracket. Note that this may be different from what happens with other Swiss systems.
However, when we operate by hand, in practice we will almost never need to make recourse to this explicit formal procedure – in general, it will suffice to compare the relevant failure values.
Towards the next bracket – The “Requirement Zero”
Now the bracket has almost been paired. The last step, before proceeding to the next bracket, is to verify that this round-pairing can actually be completed. To do this, we must prove that at least one pairing exists for all the yet unpaired players. This is informally called the “Requirement zero”. We do not need to find the correct pairing now; we are only looking for any legal pairing, and quality is no concern of ours, so that this is, of course, a far easier task – actually, it is usually easy enough73.
First, we need to define the set of players yet to be paired – we usually call it the “rest”74. Looking into the crosstable, we readily find that the rest is {4, 8, 9, 10, 11, 13, 14}. Then we can immediately find a legal pairing, e.g. [4-8, 9-10, 11-13, 14-PAB]. Of course, this pairing, which is “almost random”, will almost certainly not be the correct one – but this is irrelevant: as we mentioned, we just want to be sure that at least one legal pairing exists. Since we just found one, we are now certain that this pairing can be completed.
By the way, we ought to note that this check, which is called a “Completion test”, is essentially useless in the early rounds of the tournament, because only very few players have already met and it is therefore virtually impossible that the pairing does not come to fruition. It will however become more and more important as we proceed in the tournament, especially if there are not many players.
The next bracket
Now, let us move to the next bracket, which contains the players who have scored 0.5 points, namely [4Ww, 11Bb]. We know that player #4 already played with #11 in the first round. Thus, it has no compatible opponent in the bracket.
73 We need no special method to find this acceptable pairing – it is just the first legal mesh-up we can think of!
74 Please note that this rest has nothing to do with the remainder, which is the residual part of a heterogeneous bracket, after the MDP-Pairing (see B.3).
Since no possible pairing exists, we have no other option but to move both players #4 and #11 down to the next bracket.
From a formal point of view, we can say that our initial estimate of MaxPairs was wrong, and we must thus mend it – actually, the correct value is zero, and therefore the perfect candidate will have no pairs and two downfloaters.
Those players, who are downfloaters in this bracket, will be moved-down players (MDPs) in the next bracket, where they are going to play against opponents with lower scores. Likewise, their opponents, who are usually called “upfloaters”, will play against higher scoring opponents.
The pairing of two players with different scores, although sometimes unavoidable, is a violation of the basic principles of Swiss systems [C.04.1.e]. Therefore, in order to avoid making players float too often, every player who is going to play with a lower-scored opponent, receives a special flag, which is called a downfloat. In the same way, every player who is going to play with a higher-scored opponent receives a special flag called upfloat. We mark those events on the players’ cards, or on the tournament board, respectively with a downward arrow “↓” (often replaced for convenience by a lowercase “v”) for downfloats; or with an upward arrow “↑” (often replaced by a “^”) for upfloats. The pairing system protects players from repetitions of a same kind of floating, limiting such repetitions for the next round [C.12, C.13] and for the following one [C.14, C.15].
Before proceeding to the next, and last, bracket, we should verify that the selected downfloaters maximise the pairing in the next bracket, and that the Requirement Zero is satisfied. Actually, there is no need to do this now, because the new bracket will contain all the rest from the previous bracket, and we already know that that rest can be paired (because it passed the completion test at the end of the pairing for the previous bracket).
The last bracket
Having exhausted (so to speak...) the half point bracket, we finally go to the last and lowest bracket, namely the one with zero points. This is a heterogeneous bracket, since it contains not only players with zero points, but also the two half point downfloaters from the previous bracket. For clarity, we keep downfloaters
separated from other players: [4Ww 11Bb] [9Bb 8Ww 10Ww 14Ww 13Bb] (player #12 is absent, and therefore is not in the list and receives a zero points forfeit, with no opponent and no colour, which is a downfloat too).
Before proceeding with the pairing, we try to compute the usual parameters. First, we have 7 players; since this is the last bracket, we are certain (thanks to the completion test!) that we will be able to pair all them, except the “odd one”, who shall get the PAB. Hence, for once we know that MaxPairs=3. Moreover, we have two MDPs, M0 = 2; and we have no reason to think that they cannot be paired – therefore, at least for the moment75, we can safely assume M1 = 2. Four players “prefer” white, while three prefer black. Hence, we should be able to satisfy all colour preferences and x = 0, while z is useless.
Since the bracket is a heterogeneous one, the procedure is a little different from that that we used for the previous brackets [B.3]. The formal procedure is as follows: first, putting in S1 only the MDPs, we build an MDP-Pairing, obtaining some pairs and a remainder. The latter is then paired in the same way as a homogeneous bracket, giving some more pairs. All the pairs, put together, form the complete pairing of the bracket, which shall be evaluated.
We shall therefore put in S1 only the two MDPs, to form two pairs. The initial pairing scheme is:
The resulting MDP-Pairing [B.3] is 4-8, 11-9. We should then proceed to build the remainder and pair it. However, of course, we do not need to be as dumb as a computer: in this case, it is apparent that the colour matching is unsatisfactory in both the pairs of the MDP-Pairing – while, since x = 0, we know we should satisfy all colour preferences. Hence, we go straight on to B.5, where we learn that we should apply B.7 to straighten the situation.
75 The parameter M1 must be “divined” in much the same way as MaxPairs. As we mentioned, an educated guess usually allows us to estimate its value, but sometimes we may find our estimate wrong, and therefore we have to correct it. Nonetheless, we should remember that this parameter is not a variable but a constant of the bracket.
The first attempt should be to apply transpositions and exchanges in the remainder, to try to make the pairing better; but, of course, no alteration in the order of the remainder can possibly change the MDP-Pairing. Therefore, we boldly jump straight to the next step, applying a transposition to the S2 subgroup of the complete bracket, in order to modify the MDP-Pairing (perhaps changing the remainder too, but for the moment we do not care).
S1 - 4Ww 11Bb
S2 - 9Bb 8Ww 10Ww 13Bb 14Ww
Once again, we must thus make use of rule D.1, which give instructions as to how to generate all the transpositions in the correct order. For heterogeneous brackets, this rule gives us a most practical hint, specifying that we are interested in the lexicographical order of only the first N1 elements of S2, where N1 is the number of elements in S1 – in our case, this is the number of MDPs to be paired, which is M1=2. Hence, having assigned the BSNs to the players of the bracket in the usual way [1, 2][3, 4, 5, 6, 7], we only need to focus on the first two elements of the list for S2. There, we need to change both the pairings, and the first transposition that meets this need is [4, 3, x, x, x] – while, of course, any other transpositions brings higher BSNs in the first two positions and is hence higher in the lexicographical order76. The next candidate to try is therefore:
We thus obtain the pairs 4-9 and 8-11 for the MDP-Pairing, which seem to be satisfactory, and can now proceed to the pairing of the remainder, which is
{10Ww, 13Bb, 14Ww}. We begin by building the subgroups S1R and S2R. The pairing scheme is now:
S1R - 10Ww S2R 13Bb
The pairing of the remainder is 10-13; player #14 ends up unpaired. We can now put together the MDP-Pairing and the remainder pairing, to build the
76 A not rigorous but simple way to see the procedure is as follows: take the first player of S1, then scroll S2 until a match is found, keeping in mind that we have to make x pairs containing a disregarded colour preference. Then repeat this procedure with the second element of S1, the third, and so on, until all of S1 is used up.
complete candidate, which is 4-9, 8-11, 10-13, (14). We must now evaluate this candidate: since it complies with all the pairing criteria, it is perfect – and is therefore immediately approved. Player #14, as directed by the Rules, receives a PAB: 1 point, no opponent, no colour [A.5]. The player who receives the PAB also receives a downfloat [A.4.b], which is annotated on the player’s card.
To complete the preparation of the round, we now assign colours and rearrange chessboards. The unordered pairs we built are: 1Bb-5Bb, 2Ww-7Bb, 3Bb-6Ww, 4Ww-9Bb, 11Bb-8Ww, 10Ww-13Bb; #12 is absent, while bye goes to #14. We need to examine those pairs one by one, accordingly to colour allocation criteria (see part E of the Rules), which are very logical and reasonable:
- If possible, we satisfy both players [E.1];
- If we can’t satisfy both players, we satisfy the strongest colour preference: first are absolute preferences, then strong ones, mild ones come last [E.2];
- All above being equal, we alternate colours with respect to the last time they played with different colours [E.3]. It may happen that in the sequence of colours (or “colour history”) there are “holes”, of course in correspondence with unplayed games (due to a bye or forfeit). In this case, we simply skip those “holes”, moving them to the beginning of the sequence – basically, this means that we look at the colour of the previous played game.
- All above being still equal, we satisfy the colour preference of the higher ranked player – thus, the player with higher score or, if scores are tied, the one who comes first in the initial ranking list [E.4].
The last item is just the one that applies in assigning colours to the pair 1-5: the players in this pair have the same colour preference and identical colours histories. We shall therefore assign black to player #1. In all other pairs, we can satisfy both players - and so we shall do.
Having thus finished the preparations for the second round, we check the order of chessboards and publish the pairing (indeed, to cut it short we post the results too):
| 1 | 5 (1.0) - 1 (1.0) | 1-0 |
| 2 | 2 (1.0) - 7 (1.0) | 1-0 |
| 3 | 6 (1.0) - 3 (1.0) | ½-½ |
| 4 | 4 (0.5) - 9 (0.0) | 1-0 |
| 5 | 8 (0.0) - 11 (0.5) | 0-1 |
| 6 | 10 (0.0) - 13 (0.0) | 1-0 |
| 7 | 14 (0.0): PAB | 1F |
We must now pair the third round. We already had a little practice, so we can go a bit faster – but without neglecting any of the necessary checks and cautions!
As usual, our first task is the determination of all the colour preferences. We want to notice that player #5 has an absolute colour preference – hence, we know right from the beginning that the player shall be assigned its due colour. We may also want to observe that players #12 and #14 played one game less than the others did, so their colour preference is strong, while all remaining players have only mild preferences.
There are no absent players in this round, so all players shall be paired. The tournament board (see below) has been duly updated with all the relevant data (opponents, colours, results, scores, floats, colour preferences).
| Player | PN | 1 | 2 | 3 | 4 | 5 | 6 | ||||||
| Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | ||
| Alice | 1 | 8W+ | 1.0 | 5B- | 1.0 | w |
|
|
|
|
|
|
|
| Bruno | 2 | 9B+ | 1.0 | 7W+ | 2.0 | b |
|
|
|
|
|
|
|
| Carla | 3 | 10W+ | 1.0 | 6B= | 1.5 | w |
|
|
|
|
|
|
|
| David | 4 | 11B= | 0.5 | 9W↓+ | 1.5 | b |
|
|
|
|
|
|
|
| Eloise | 5 | 12W+ | 1.0 | 1W+ | 2.0 | B |
|
|
|
|
|
|
|
| Finn | 6 | 13B+ | 1.0 | 3W= | 1.5 | b |
|
|
|
|
|
|
|
| Giorgia | 7 | 14W+ | 1.0 | 2B- | 1.0 | w |
|
|
|
|
|
|
|
| Kevin | 8 | 1B- | 0.0 | 11W↑- | 0.0 | b |
|
|
|
|
|
|
|
| Louise | 9 | 2W- | 0.0 | 4B↑- | 0.0 | w |
|
|
|
|
|
|
|
| Mark | 10 | 3B- | 0.0 | 13W+ | 1.0 | b |
|
|
|
|
|
|
|
| Nancy | 11 | 4W= | 0.5 | 8B↓+ | 1.5 | w |
|
|
|
|
|
|
|
| Oskar | 12 | 5B- | 0.0 | ZPB↓ | 0.0 | Ww |
|
|
|
|
|
|
|
| Patricia | 13 | 6W- | 0.0 | 10B- | 0.0 | w |
|
|
|
|
|
|
|
| Robert | 14 | 7B- | 0.0 | PAB↓ | 1.0 | Ww |
|
|
|
|
|
|
|
From the board, we can build the five scoregroups. For our convenience, we indicate colour preference and possible float markers for the last and previous rounds77 for each player. Let us notice that the number of upfloats can be less
77 The convention we adopt, which is widespread enough, uses a downward arrow for a downfloat and an upward arrow for an upfloat. A single arrow indicates a float had in the last round. When there are two arrows, the first (from left to right) indicates the float marker for the last round, while the second indicates the float marker for the previous (last-but-one) round. When we have to indicate a float in the last-but-one round but no float in the last round, we put a “-” sign in the first place – e.g., “-↓” indicates a downfloat in the last-but-one round, and no float in the last round.
than or equal to the number of downfloats. In this round, in fact, we have more downfloats than upfloats – this is normal and happens every time there are byes or unplayed games.
Before even starting the pairing, we want to check that the players can actually be paired (the by now usual “Requirement Zero”). This is easy. For example, looking at the crosstable we see that (2-5, 3-4, 6-11, 1-7, 10-14, 8-9, 12-13) is a legal pairing78, so we can proceed.
Here are the scoregroups: 2.0 {2b, 5B}
1.5 {3w, 4b↓, 6b, 11w↓}
1.0 {1w, 7w, 10b, 14Ww↓}
0.0 {8b↑, 9w↑, 12Ww↓, 13w}
As usual, we process the above scoregroups one by one from top to bottom, building and pairing the brackets as we go. By the way, there is no half point scoregroup. This happens now and then and is completely normal. After having paired the one point scoregroup, we simply proceed to the next one, which is the one with zero points.
The first bracket, whose players scored 2 points, is [2b, 5B] (MaxPairs=1, M1=M0=0, x=1, while z is ignored because there is just one non-absolute preference)79. We are requested to form just one pair. Since the two players have not played each other, they can be paired. This is the only one possible pairing, and it is legal – therefore, there is no need to evaluate it, no better pairing can exist! However, we must perform (as always) the completion test, to see that the rest of the players can actually be paired. This check is straightforward (it may be descended right from the previous one), and is successful, so we may proceed.
78 Once again, we want to emphasise that we do not care in the least how bad this pairing may be (and, by the way, actually is) – it is a legal one, and this is all we need to be certain that at least one legal pairing exists!
79 From now on, we will make explicit reference to the computation of the bracket parameters only when necessary; however, their values are always found as previously discussed.
To complete the pairing for the bracket, we only need to allocate colours80. Here, we must satisfy the stronger colour preference, which is the absolute one of player #5, so the pairing is 2-5. As already mentioned, we may as well observe that player #2 will have an absolute colour preference for black on the next round.
S1 - 3w 4b↓
S2 - 6b 11w↓
The next bracket, with 1.5 points, is [3w, 4b↓, 6b, 11w↓] (MaxPairs=2, M0=M1=0, x=0). Before starting the pairing, we may want to look at the bracket and take notice of its possible peculiarities. For example, here we observe that we already had the games 6-3 and 11-4, and that players 4 and 11 just had a downfloat. Such information will help us not to waste time and efforts in the proceedings. And now, to business! The initial candidate is:
Here, both pairs are forbidden (the players already played each other [C.1]). Therefore, this candidate is not a legal one, and we cannot help but reject it.
S1 - 4b↓
S2 - 11w↓ 6b
Therefore, to build the next candidate, we move on to the first transposition (which, in this case, is also the only one) [B.5, D.1]:
Still we are not lucky: this candidate contains two pairs that disregard colour preferences – therefore, since x = 0, it is not perfect. However, we store it somewhere as a “provisional-best”. If we cannot find a better candidate, this one will still be usable. As we build more candidates, we will evaluate them against the current provisional-best, always keeping the better of the two as provisional- best. If at any time we find a perfect candidate, we choose it at once, discarding any possible provisional-best. However, after all the possible candidates are used up, if we found no perfect candidate at all, the surviving provisional-best is actually the best candidate we can have, and therefore the one to be used.
Since this was the last possible transposition, and the bracket is homogeneous,
80 In the previous rounds, we (correctly) left the allocation of colours as a final phase of the pairing, to better focus the problems of pairing itself. However, after the completion test succeeds, we will never have to change the pairing of the bracket, so we have no strong reason to delay the colour allocation.
we must try a resident exchange, which is a swap of resident players between S1 and S2 [B.6, D.2]. We take a player from S2 and swap it for a player from S1, in an attempt to obtain an acceptable pairing. If the exchange of one player is not enough, we can swap two, three, and so on81 - until we find a solution (or use up all the possible exchanges).
A single exchange (one player for one player) is usually rather easy, but the matter may become definitely tricky when more players are involved. To avoid errors in the exchange, we want to follow the general rules always:
- first, we shall exchange as few players as possible – this item needs no explanation...
- then, we exchange the smallest possible difference between the sum of BSNs of the moved players. To clarify this rule, let us consider the example of an exchange between players P1a and P1b from S1 and players P2a and P2b from S2 – all represented by their BSNs. From the principle that the exchanged players must be as near in ranking as possible, we obtain that, on the whole, the differences between the BSNs of exchanged couples must be as little as possible. To comply with this principle, the rules choose to minimise the difference of the sums, which is equal to the sum of those differences82
81 Let us consider the example of a six players bracket {[1,2,3][4,5,6]}. Let us exchange players 3 and 4 between S1 and S2, thus obtaining the new subgroups composition {[1,2,4][3,5,6]}. This exchange is “useful”, because it gives us candidates that were not previously found (e.g., 1-3, …). Now, let us exchange players (3,5), then (2,4), then again (2,5), and so on. Each new exchange gives some new candidates, so all these exchanges are useful (at least until we try to exchange player #1: actually, this player was already paired with all possible opponents during the previous exchanges, so this exchange is useless). However, candidates containing pairs formed by the exchanged players are always useless, because they were already checked – e.g., exchanging (3,4) we obtain among the others, also the candidates (1-5, 2-6, 4-3) and (1-6, 2-5, 4-3) that were already examined as (1-5, 2- 6, 3-4) and (1-6, 2-5, 3-4) – and this is easily extended to brackets containing any number of players. Now, let us consider the exchange of two players per subgroup, e.g. {[1,4,5][2,3,6]}: now, every candidate contains at least one pair formed by exchanged players and is therefore useless. We may see that this situation happens in any bracket, each time the number of exchanged players is greater than half the number of players in S2 (which is the largest subgroup). We conclude that there is a theoretical maximum to the number of useful exchanges in the bracket, which is half the number of players in S2 (rounded down if needed).
82 In general, this sum is S = (P2a-P1a) + (P2b-P1b) + (P2c-P1c) + (P2d-P1d) + … or, readjusting the terms, S = (P2a+P2b+P2c+P2d+…) – (P1a+P1b+P1c+P1d+…). Actually, the difference of the sums would be simpler to understand as a rule. However, the Rules choose to use the difference of the sums because, from a practical point of view, computing it is a bit simpler than computing the sum of differences.
- then again, when we have two possible exchanges with the same difference, we must decide which one is to be tried first of the two. The first criterion it to choose the lowest possible exchanged player(s) in S1 (and therefore the greatest different BSN83). For example, exchanging players (3, 4) from S1 is better than exchanging players (2, 4) – but it is worse than exchanging players (1, 5), because, in S1, exchanging #5 is always better than exchanging #4, whoever the higher ranked players in the exchange may be
- finally, when two exchanges have not only the same differences, but also the same exchanged players from S1, we choose the exchange with the higher players(s) of S2 (and therefore the one with the lowest different BSN). Just as in the previous case, this may sometimes lead to seemingly peculiar choices. For example, exchanging players (7, 10) is better than exchanging players (8, 9), but is worse than exchanging (6, 11) – because, in S2, exchanging #7 is always better than exchanging #8, and exchanging #6 is even better, whoever the lower ranked players in the exchange may be.
After the exchange, the subgroups S1 and S2 must be put in order in the usual way [A.2] (which we only seldom need to do, because they usually are already in the right order).
S1- 3w 6b
S2 - 4b↓ 11w↓
Well, now we may proceed to the first possible exchange, which is between players #4 and #6 and yields the following candidate:
This candidate is perfect, so we can now discard the previously stored “provisional-best” and form the pairs 3-4, 11-6. Now, we perform the completion test – which will of course be successful. The allocation of colour is straightforward: 3-4, 11-6.
Now we can move on to the one point bracket: [1w, 7w, 10b, 14Ww↓]
83 To compare the two exchanges and find the first of the two, we begin with the lowest players (highest BSNs), comparing the corresponding BSNs; if they are different, we choose the exchange with the higher BSN. If, on the contrary, they are equal, we proceed to the next lowest players, and repeat the comparison until we find the first difference or we use up all the players to exchange.
(MaxPairs=2, x=1). Here, players 7 and 14 already played with each other. One of the players has a strong colour preference and a downfloat.
The first pairing candidate is:
S1 - 1w 7w
S2 - 10b 14Ww↓
S1 - 1w 7w
S2 - 14Ww↓ 10b
and of course it is not acceptable [C.1]. Let’s then proceed to the first (and, once again, only one) transposition:
Since x = 1, this is a perfect pairing. The completion test is passed and the pairs are 14-1 ([E.2]: the colour preference of player 14 is stronger than that of player 1) and 7-10 ([E.1]).
S1 - 8b↑ 9w↑
S2 - 12Ww↓ 13w
No players have a half point score; the next bracket to be paired is the lowest one, with zero points. It is comprised of [8b↑, 9w↑, 12Ww↓, 13w] (MaxPairs=2, x=1). Player #12, who was absent in the previous round and therefore lost by forfeit, has now a strong colour preference and a downfloat84 [A.4.b]. Then we have the following candidate pairing:
Strangely enough, we were lucky at the first shot... Since the candidate is perfect, let us thank our good fate and accept the proposed pairs. As to the colours, the first pair is 12-8, in agreement with both preferences [E.1], while for the second one, in which players have not only identical preferences but also the same colours histories, we satisfy the colour preference of the higher ranked player [E.4], thus obtaining 9-13.
We are done! After checking everything as usual, and particularly the order of chessboards, we may publish the pairing and let the round begin.
| 1 | 2 (2.0) - 5 (2.0) | ½-½ |
84 We register the presence of float markers as a matter of completeness – but please note that previous upfloats are irrelevant in any ordinary homogeneous bracket, while downfloats are irrelevant if the bracket does not produce downfloaters.
| 2 | 3 (1.5) - 4 (1.5) | ½-½ |
| 3 | 11 (1.5) - 6 (1.5) | 0F-1F |
| 4 | 14 (1.0) - 1 (1.0) | 0-1 |
| 5 | 7 (1.0) - 10 (1.0) | 1-0 |
| 6 | 12 (0.0) - 8 (0.0) | ½-½ |
| 7 | 9 (0.0) - 13 (0.0) | 1-0 |
Twist! Player #11 does not show in time to play, thus forfeiting the game: we need to fix the pairing cards (if used) and/or the tournament board to reflect this mishap, especially in the light of the fact that the pairing between #6 and #11, not having actually been put in practice, may be repeated in a future round. Moreover, both players get a downfloat.
-
-
- FOURTH ROUND (CHOOSING THE BEST POSSIBLE CANDIDATE) After the third round, our tournament board is as follows. For our convenience, from now on, we will report the colour preferences and the possible last two
-
floats for each player. The hyphen (“-”) indicates that the player did not float in
the last round, but it did in the previous round. By the way, at this point, a piece of advice is in order: as we proceed in the tournament, we collect more and more data, and overlooking something becomes easier and easier... We should always pay extreme attention while posting data on the board, and inspect everything two, three or even more times: as strange as it may seem, making a mistake is really easy!
| Player | PN | 1 | 2 | 3 | 4 | 5 | 6 | ||||||
| Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | ||
| Alice | 1 | 8W+ | 1.0 | 5B- | 1.0 | 14B+ | 2.0 | W |
|
|
|
|
|
| Bruno | 2 | 9B+ | 1.0 | 7W+ | 2.0 | 5W= | 2.5 | B |
|
|
|
|
|
| Carla | 3 | 10W+ | 1.0 | 6B= | 1.5 | 4W= | 2.0 | Bb |
|
|
|
|
|
| David | 4 | 11B= | 0.5 | 9W↓+ | 1.5 | 3B= | 2.0 | Ww-↓ |
|
|
|
|
|
| Eloise | 5 | 12W+ | 1.0 | 1W+ | 2.0 | 2B= | 2.5 | Bb |
|
|
|
|
|
| Finn | 6 | 13B+ | 1.0 | 3W= | 1.5 | +000↓ | 2.5 | b↓ |
|
|
|
|
|
| Giorgia | 7 | 14W+ | 1.0 | 2B- | 1.0 | 10W+ | 2.0 | Bb |
|
|
|
|
|
| Kevin | 8 | 1B- | 0.0 | 11W↑- | 0.0 | 12B= | 0.5 | Ww-↑ |
|
|
|
|
|
| Louise | 9 | 2W- | 0.0 | 4B↑- | 0.0 | 13W+ | 1.0 | Bb-↑ |
|
|
|
|
|
| Mark | 10 | 3B- | 0.0 | 13W+ | 1.0 | 7B- | 1.0 | Ww |
|
|
|
|
|
| Nancy | 11 | 4W= | 0.5 | 8B↓+ | 1.5 | -000↓ | 1.5 | w↓↓ |
|
|
|
|
|
| Oskar | 12 | 5B- | 0.0 | ZPB↓ | 0.0 | 8W= | 0.5 | b-↓ |
|
|
|
|
|
| Patricia | 13 | 6W- | 0.0 | 10B- | 0.0 | 9B- | 0.0 | W |
|
|
|
|
|
| Robert | 14 | 7B- | 0.0 | PAB↓ | 1.0 | 1W- | 1.0 | b-↓ |
|
|
|
|
|
The scoregroups now are: 2.5 {2B, 5Bb, 6b↓}
2.0 {1W, 3Bb, 4Ww-↓, 7Bb}
1.5 {11w↓↓}
1.0 {9Bb-↑, 10Ww, 14b-↓}
0.5 {8Ww-↑, 12b-↓}
0.0 {13W}
As always, before starting the pairing we must check the Requirement Zero, viz. we must verify that at least one pairing exists for all the players. For example, we may have the pairing (2-6, 5-3, 1-4, 7-11, 9-10, 14-8, 12-13). As usual, this pairing is almost certainly not the one we are looking for – but the pairing is possible, and therefore we may proceed.
The first bracket is: [2B, 5Bb, 6b↓] (MaxPairs=1, x=1). Here, players #2 and #5 already played with each other [C.1] and the first candidate pairing is therefore not legal. We should go to the first transposition [C.7], which yields the pair 6-2 – while player #5 shall float to the next bracket (with 2 points):
[5Bb][1W, 3Bb, 4Ww-↓, 7Bb] (MaxPairs=2, M0=M1=1, x = 0), which gives:
S1 - 5Bb
S2 - 1W 3Bb 4Ww-↓ 7Bb
We already had the pair 5-1 in the second round. Hence, the first MDP-Pairing cannot give origin to any legal candidate – and, therefore, we may discard it at once.
The first useful transposition yields for the MDP-Pairing the pair 5-3, which is legal but does not comply with C.10, because of colliding colour preferences (since the failure value for C.10, relative to the complete pairing of the bracket, cannot be less than 1, no pairing originating from this MDP-Pairing will be perfect).
However, the evaluation should be made on the complete bracket pairing, so we proceed to pair the remainder, which is now [1W, 4Ww-↓, 7Bb]. The first candidate is thus (5-3, 1-4, 7 to float), which presents us with a failure value for criterion C.10 equal to 2 – we will write it as C.10(2) for short. Since the candidate is legal, we store it as provisional-best and proceed with a transposition in the subgroup S2R of the remainder [B.7], obtaining the new candidate (5-3, 1-7, 4 to float), which has failure values C.10(1), and C.14(1). This candidate is not perfect, but has a lower failure value for C.10 than the
provisional-best. Hence, it becomes the new provisional-best (the previous one is discarded).
However, we shall proceed to examine more candidates, because we did not find a perfect one yet. Since we used up all transpositions in S2R, we now try an exchange between S1R and S2R: we swap the last (and only) element of S1R with the first element of S2R. With this exchange, the remainder now becomes [4Ww-↓, 1W, 7Bb]. The first candidate is again (5-3, 1-4, 7 to float), which we already examined85 (and discarded). We therefore apply the only possible transposition in S2R, obtaining the new candidate (5-3, 4-7, 1 to float). This candidate had failure value C.10(1) and is therefore the new provisional-best.
The next, and last, remainder exchange gives [7Bb, 1W, 4Ww-↓], but all the candidates originating from this remainder have already been evaluated.
Now we have completed the evaluation of all the possible candidates given by the current MDP-Pairing. Since none among them is perfect, we must proceed with another transposition in the original S2, to look for a perfect candidate. The next useful transposition yields for the MDP-Pairing the pair 4-5, which is legal, and the remainder [1W, 3Bb, 7Bb]. This in turn provides us with (4-5, 1-3, 7 to float) that is, at last, a perfect candidate.
Before choosing this candidate, however, we must check that its floater maximises the number of pairs and minimises the PSD in the next bracket –and just in that one: be they as they may, we do not proceed to inspect the following brackets! The next bracket would be [7Bb] [11 w↓↓]: the two players are not incompatibles, so that we have the maximum number of pairs, and no player from the bracket just paired would lend us a better PSD.
The last check to perform is the completion test. The rest is now {7Bb, 11w, 9Bb, 10Ww, 14b, 8Ww, 12b, 13W}, and (7-11, 9-10, 14-8, 13-12) is a legal
(although awful) pairing, so we may proceed.
Before going to the next bracket, however, we may want to do some thinking about the procedure we just used. Actually, we may reason that, although we
85 See note 18, page 19.We also want to note that every time a player from the original S1 goes to S2, it can only be paired to another player of the original S1 or float, because any other pairing would give origin to a candidate that has already been evaluated (see also note 81, page 56).
cannot be sure, we may well suspect that a MDP-Pairing with unsatisfactory failure values may (likely) bring us nowhere. Sometimes, a brief inspection of the whole bracket might show that a perfect pairing exists (just as it was here) and that we do not really need to waste precious time in analysing imperfect candidates. This is of course sound reasoning, but skipping steps and jumping ahead is always a potential risk. For example, we may easily verify that in the bracket [5Bb][1B, 3Bb, 4Ww-↓, 7Bb] (x=1), which is rather similar to the previous one, the correct pairing would have been (3-5, 4-1, 7↓): a different colour preference in a player who is incompatible with the MDP, changed the latter’s pairing! The lesson we must learn from this, is that we must always be very careful, and beware of all shortcuts.
The next bracket, which is heterogeneous, is:
[7Bb] [11 w↓↓] (MaxPairs=1, M1=M0=1, x=0).
Since we have only one possible candidate, and players #7 and #11 did not play with each other, we can make the pairing at once: 11-7. The rest is now {9Bb, 10Ww, 14b, 8Ww, 12b, 13W}, and (9-10, 14-8, 13-12) is a legal pairing – therefore, the completion test is passed.
S1 - 9Bb-↑
S2 - 10Ww 14b-↓
The next bracket is: [9Bb-↑, 10Ww, 14b-↓] (MaxPairs=1, x=0), which gives us:
Here, all players are compatible and therefore can play with each other, but we have a small problem: the “natural” pairing would leave #14 unpaired - but this player had a downfloat in the second round and therefore should not get one more now [C.14].
S1 - 9Bb-↑
S2 - 14b-↓ 10Ww
The pairing is legal, but not perfect: we thus store it as provisional-best and then proceed to look for a possibly perfect, or simply better, candidate. First, we try a transposition:
Here the problem is that the players’ colour preferences are not matched well enough [C.10]. Let us compare this candidate with the provisional-best: the latter fails (once) for C.14, while this candidate fails (once) for C.10, which is worse – hence, we keep the current provisional-best.
S1 - 10Ww
S2 - 9Bb-↑ 14b-↓
Thus, even with a transposition, we cannot come to a valid conclusion, and we have to try one homogeneous exchange:
S1 - 10Ww
S2 - 14b-↓ 9Bb-↑
We already tried the pair 10-9. Thus, once again we go on to a transposition, which yields:
At last, we get the perfect pairing with 10-14, while player #9 floats to the next bracket.
To get this pairing we followed a formal procedure, which is correct but is not always the simplest possible one. Actually, there is an alternative method of pairing which, especially in the case of a bracket with few players, may be more convenient. We call it Sieve pairing86, and it is very simple: first, we generate, in due order, all the possible candidates. Then, starting from criterion C1 and proceeding one by one through all the pairing criteria, we check the failure values of the candidates, and keep only those candidates whose failure value is not worse than the best failure value for that criterion, discarding all the others. In this way, we gradually reduce the number of possibly acceptable candidates, until we are left with only one (which is immediately selected), or with a small group of them, in which case we select the first one (as they were generated in due order).
For the last bracket, which was very simple, the possible candidates were just four:
86 See “The Sieve Pairing” in [B.8], page 21.
| Candidate | Failure values |
|
| float 9Bb-↑, 10Ww, 14b-↓ | C.5(1) | dropped at C.5 -87 |
| (9Bb-↑, 14b-↓), float 10Ww | C.10(1) | dropped at C.10 |
| (9Bb-↑, 10Ww), float 14b-↓ | C.14(1) | dropped at C.14 |
| (10Ww, 14b-↓), float 9Bb-↑ | none | perfect – selected after |
C.14
In this very easy example, there is a perfect candidate, which is of course the one we must select. However, even when there is no perfect candidate, this method is very convenient because it always allows us to choose the best one easily and safely88.
We must now check that the selected floater, #9, optimises the pairing in the next bracket, which would be [9Bb-↑][8Ww-↑ 12b-↓]. Since 9-12 is a legal pairing, and a different selection of floater(s) would not give a better PSD, the floater is acceptable.
Before proceeding, we must perform the usual completion test, to see that the rest of the players may be paired. The rest is {9Bb, 8Ww, 12b, 13W}, and (9-8, 13-12) is a legal pairing, so we may proceed.
S1 - 9Bb-↑
S2 - 8w-↑ 12b-↓
The next bracket is the half point one: [9Bb-↑][8Ww-↑ 12b-↓] (MaxPairs=1, M1=M0=1, x=0), where #8 and #12 are incompatible because of [B.1.a].
Once again, the candidate is not perfect because #8 got an upfloat and #12 got a downfloat during the second round, so we have a failure for [C.14] and one for [C.15]. The only possible transposition cannot help us because x is zero and both 9, 12 have a colour preference for black, so we have a failure for [C.10].
On the other hand, an exchange would make #9 float down, giving a failure for [C.6]. Since this is the first time we find this case, we may want to expand a little about it, possibly giving an example on how to compute and compare PSDs. To do this, we need to remember the score of each player: #9 (1.0), #8
87 Although surely not a very good one, a candidate with no pairs and all players floating down is however legal and must be considered among the possible pairings (and, sometimes, it may be the only possible one).
88 Of course, the Sieve method may be used always, whatever is the number of players to pair. However, when pairing manually, this method is especially useful for “small but complicated” brackets, while for large brackets it may become rather tiring.
(0.5), #12 (0.5). Since a player shall float down from this bracket, we need to compute the artificial value used in [A.8] to calculate the score difference of that player. This value is one point less than the minimum score in the heterogeneous bracket, hence AV=-0.5. The fact that this value is negative is no concern of ours, because this value will be subtracted into a positive number, giving a positive score difference. The PSD of a candidate is simply the list of all score differences in the candidate, sorted in descending order. We have three possible candidates and the respective PSDs are:
- 9-8 [SD= score(9)-score(8)=0.5], 12-float [SD= score(12)-AV=1.0]:
PSD={1.0, 0.5}
- 9-12 [SD= score(9)-score(12)=0.5], 8-float [SD= score(8)-AV=1.0]:
PSD={1.0, 0.5}
- 8-12 [SD= score(8)-score(12)=0.0], 9-float [SD= score(9)-AV=1.5]:
PSD={1.5, 0.0}
It is at once apparent that the PSD of the last candidate, which is the one in which the MDP is floating down again, is worse than the others are, because its first element is greater [A.8].
In summary, we have three possible candidates, and neither of them is perfect. We must therefore choose the candidate whose quality is the best possible among them, and that is (9-8, downfloat to 12), because it only infringes C.14 and C.15, which is weaker than the other involved pairing criteria.
Now we must verify that the given downfloater maximises the pairing in the next bracket, which is the last one and contains only [12b-↓][13W]. The two players are compatible and there is no PAB to be assigned – therefore the floater is immediately acceptable. The final check is the completion test: now the rest is comprised only of the last bracket, which can be paired – hence, the test is passed.
In the last bracket, the only possible candidate is perfect and gives the pair 13-
12. As usual, we check everything, rearrange (if necessary) the chessboards order, start the round - and reach the fifth round.
| 1 | 6 (2.5) - 2 (2.5) | 0-1 |
| 2 | 4 (2.0) - 5 (2.5) | ½-½ |
| 3 | 1 (2.0) - 3 (2.0) | 1-0 |
| 4 | 11 (1.5) - 7 (2.0) | 1-0 |
| 5 | 10 (1.0) - 14 (1.0) | ½-½ |
| 6 | 8 (0.5) - 9 (1.0) | ½-½ |
| 7 | 13 (0.0) - 12 (0.5) | 1-0 |
- FIFTH ROUND (PPB AND CLB)
After the fourth round is played out, the tournament board is as follows:
| Player | PN | 1 | 2 | 3 | 4 | 5 | 6 | ||||||
| Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | ||
| Alice | 1 | 8W+ | 1.0 | 5B- | 1.0 | 14B+ | 2.0 | 3W+ | 3.0 | b |
|
|
|
| Bruno | 2 | 9B+ | 1.0 | 7W+ | 2.0 | 5W= | 2.5 | 6B+ | 3.5 | w |
|
|
|
| Carla | 3 | 10W+ | 1.0 | 6B= | 1.5 | 4W= | 2.0 | 1B- | 2.0 | w |
|
|
|
| David | 4 | 11B= | 0.5 | 9W↓+ | 1.5 | 3B= | 2.0 | 5W↑= | 2.5 | b↑ |
|
|
|
| Eloise | 5 | 12W+ | 1.0 | 1W+ | 2.0 | 2B= | 2.5 | 4B↓= | 3.0 | W↓ |
|
|
|
| Finn | 6 | 13B+ | 1.0 | 3W= | 1.5 | +000↓ | 2.5 | 2W- | 2.5 | B-↓ |
|
|
|
| Giorgia | 7 | 14W+ | 1.0 | 2B- | 1.0 | 10W+ | 2.0 | 11B↓- | 2.0 | w↓ |
|
|
|
| Kevin | 8 | 1B- | 0.0 | 11W↑- | 0.0 | 12B= | 0.5 | 9W↑= | 1.0 | b↑ |
|
|
|
| Louise | 9 | 2W- | 0.0 | 4B↑- | 0.0 | 13W+ | 1.0 | 8B↓= | 1.5 | w↓ |
|
|
|
| Mark | 10 | 3B- | 0.0 | 13W+ | 1.0 | 7B- | 1.0 | 14W= | 1.5 | b |
|
|
|
| Nancy | 11 | 4W= | 0.5 | 8B↓+ | 1.5 | -000↓ | 1.5 | 7W↑+ | 2.5 | Bb↓↑ |
|
|
|
| Oskar | 12 | 5B- | 0.0 | ZPB | 0.0 | 8W= | 0.5 | 13B↓- | 0.5 | Ww↓ |
|
|
|
| Patricia | 13 | 6W- | 0.0 | 10B- | 0.0 | 9B- | 0.0 | 12W↑+ | 1.0 | b↑ |
|
|
|
| Robert | 14 | 7B- | 0.0 | PAB↓ | 1.0 | 1W- | 1.0 | 10B= | 1.5 | Ww |
|
|
|
After having checked that at least one pairing is legal (e.g. 1-2, 3-5, 4-6, 7-8, 9-
10, 11-12, 13-14), we separate the scoregroups as usual:
| 3.5 | {2w} |
| 2.0 | {1b, 5W↓} |
| 2.5 | {4 b↑, 6B-↓, 11Bb↓↑} |
| 2.0 | {3w, 7w↓} |
| 1.5 | {9w↓, 10b, 14Ww} |
| 1.0 | {8b↑, 13b↑} |
| 0.5 | {12Ww↓} |
The first bracket, with 3.5 points, is [2w] (MaxPairs=0) - but, with a lonely player, there is not very much to do... it can’t help but float down to the next bracket, which is the one with 3 points: [2w][1b, 5W↓] (MaxPairs=1, M0=M1=0, x=0). Here, 1-5 and 2-5 already met each other. Thus, the only remaining candidate is 2-1, which is legal and is hence accepted. The completion test is successful and we may proceed to the next bracket.
The next bracket is heterogeneous: [5W↓] [4b↑, 6B-↓, 11Bb↑] (MaxPairs=2, M0=M1=1, x=1, z=0). The games 4-5 and 11-4 have already been played.
Therefore, out of necessity, whichever the float status of players is, we will have to use the only legal pair 4-6, and therefore 5-11, even if this pairing is not perfect (it fails because of C.13). In this case, the Sieve pairing method helps us not to waste any time – just excluding any other candidate simply because it would be an illegal one.
Before going ahead to the next bracket, we want to perform the completion test. The rest is now {3w, 7w↓, 9w↓, 10b, 14Ww, 8b↑, 13b↑, 12Ww↓}, which allows the legal pairing (3-7, 9-10, 8-13, 12-14).
In the next bracket, with 2 points, we have players [3w, 7w↓] (MaxPairs=1), who did not play with each other in previous rounds - therefore they can be paired89. The players have identical preferences and colours histories – therefore, we satisfy the colour preference of the highest ranked player, thus obtaining the pair 3-7 – which satisfies the completion test.
With 1.5 points, we have [9w↓, 10b, 14Ww]. The first candidate yields 9-10 and player #14 floats to the next bracket, which is [14Ww][8b↑, 13b↑]. Here, all players are compatible, and therefore the selected downfloater complies with
C.7. The rest is {14Ww, 8b↑, 13b↑, 12Ww↓}, which allows the pairing (8-13, 12-14), hence the completion test is passed too.
Let us now go to the next bracket: [14Ww][8b↑, 13b↑] (MaxPairs=1, M0=M1=1, x=0). The first candidate here is 14-8, which is legal but violates
C.7 (because player #13, as a downfloater, does not maximise the pairing in the following bracket, where it is incompatible with the only resident, player #12) and C.13 (because player #8 already got an upfloat in the previous round). We store it as provisional-best, and proceed to examine the next candidate, which is 14-13. Once again, the candidate is legal but shows C.7 and C.13 failures: since it is not better than the current provisional-best, this candidate is discarded. With
89 When, as in this bracket, all the colour preferences are for the same colour, x is useless and we may simply omit its calculation. The same applies when only one player in a bracket expects a different colour than all the other players, and the bracket does not produce floaters (because, in that case, the player’s colour preference will be unavoidably complied with). In such cases, we may ignore the corresponding criterion C.10. Please note that, even if x is useless, the same is not necessarily true for z. If, for example, all the preferences are for black, and half of them are strong and the other half are mild, there is no way to change the number of total disregarded preferences (hence x is useless). However, there is a way to minimise the number of disregarded strong preferences and, therefore, we want to use z as a guideline for this optimisation.
an exchange, we have 8-13, which violates both C.6 and C.10 and therefore is worse than the current provisional-best – so we discard it too. Since there are no more possible candidates, we must elect the current provisional-best. Let us then consider the first candidate, 14-8, and check if the player #13, as a downfloater, satisfies the Requirement Zero. The next scoregroup, as mentioned, contains only the player #12, who has already met #13 in the tournament. Hence, the latter, as a downfloater, not only violates C.7, but also yields a failure on the completion test: we are stuck with a failure in Requirement zero.
The bracket we are pairing, which is [14Ww][8b↑, 13b↑], now becomes the PPB, while the rest of the players – which, in this case, is simply {12Ww↓} – becomes the SCS [A.3, A.9].
For the pairing of the CLB, we no longer have to comply with the optimisation of downfloaters [C.7], but with a different criterion: the downfloater(s) generated by this bracket must pair the rest of the players. Since this criterion is of a higher level than the optimisation of the PSD in the bracket [C.6], this allows us to let any player float. It is very easy to verify that the one and only downfloater, which complies with requirement C.4, is #14 (which, in this bracket, is simply the only player compatible with #12).
The pairing of the PPB is thus 13-8, leaving #14 to downfloat as required. Of course, there is no need to perform a completion test, because we already selected the floater in such a way as to make the complete pairing possible. The CLB is now built putting together the MDP from the PPB and the rest of the players: [14Ww][12Ww↓], and is immediately paired.
The last thing to do is colours allocation. Both players in the CLB have identical (strong) colour preferences. Let’s look at the colours histories of the players: 14:B-WB; 12:B-WB, which are yet again identical. We cannot help but satisfy the colour preference of the higher ranked player [E.4], which is of course #14 who has a higher score - thus, we obtain 14-12. Let’s see what shall be of players #8 and #13: both have mild colour preferences, but now the colours histories are different: 8:BWBW; 13:WBBW - thus, we should alternate colours with respect to the last round in which they played with different colours [E.3], obtaining 13-8. As usual, we double-check everything, then we start the round.
| 1 | 2 (3.5) - 1 (3.0) | ½-½ |
| 2 | 5 (3.0) - 11 (2.5) | 1-0 |
| 3 | 4 (2.5) - 6 (2.5) | ½-½ |
| 4 | 3 (2.0) - 7 (2.0) | 0-1 |
| 5 | 9 (1.5) - 10 (1.5) | 0-1 |
| 6 | 14 (1.5) - 12 (0.5) | 1-0 |
| 7 | 13 (1.0) - 8 (1.0) | 0-1 |
- SIXTH ROUND (PPB AND CLB AGAIN)
After the fifth round is played out, the tournament board is as follows:
| Player | PN | 1 | 2 | 3 | 4 | 5 | 6 | ||||||
| Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | ||
| Alice | 1 | 8W+ | 1.0 | 5B- | 1.0 | 14B+ | 2.0 | 3W+ | 3.0 | 2B↓= | 3.5 | Ww↓ |
|
| Bruno | 2 | 9B+ | 1.0 | 7W+ | 2.0 | 5W= | 2.5 | 6B+ | 3.5 | 1W↑= | 4.0 | Bb↑ |
|
| Carla | 3 | 10W+ | 1.0 | 6B= | 1.5 | 4W= | 2.0 | 1B- | 2.0 | 7W- | 2.0 | Bb |
|
| David | 4 | 11B= | 0.5 | 9W↓+ | 1.5 | 3B= | 2.0 | 5W↑= | 2.5 | 6W= | 3.0 | B-↑ |
|
| Eloise | 5 | 12W+ | 1.0 | 1W+ | 2.0 | 2B= | 2.5 | 4B↓= | 3.0 | 11W↓+ | 4.0 | Bb↓↓ |
|
| Finn | 6 | 13B+ | 1.0 | 3W= | 1.5 | +000↓ | 2.5 | 2W- | 2.5 | 4B= | 3.0 | w |
|
| Giorgia | 7 | 14W+ | 1.0 | 2B- | 1.0 | 10W+ | 2.0 | 11B↓- | 2.0 | 3B+ | 3.0 |
W-↓ |
|
| Kevin | 8 | 1B- | 0.0 | 11W↑- | 0.0 | 12B= | 0.5 | 9W↑= | 1.0 | 13B+ | 2.0 | Ww- |
|
| Louise | 9 | 2W- | 0.0 | 4B↑- | 0.0 | 13W+ | 1.0 | 8B↓= | 1.5 | 10W- | 1.5 | Bb-↓ |
|
| Mark | 10 | 3B- | 0.0 | 13W+ | 1.0 | 7B- | 1.0 | 14W= | 1.5 | 9B+ | 2.5 | Ww |
|
| Nancy | 11 | 4W= | 0.5 | 8B↓+ | 1.5 | -000↓ | 1.5 | 7W↑+ | 2.5 | 5B- | 2.5 | w-↑ |
|
| Oskar | 12 | 5B- | 0.0 | ZPB | 0.0 | 8W= | 0.5 | 13B↓- | 0.5 | 14B↑- | 0.5 | W↑↓ |
|
| Patricia | 13 | 6W- | 0.0 | 10B- | 0.0 | 9B- | 0.0 | 12W↑+ | 1.0 | 8W- | 1.0 | B-↑ |
|
| Robert | 14 | 7B- | 0.0 | PAB↓ | 1.0 | 1W- | 1.0 | 10B= | 1.5 | 12W↓+ | 2.5 | b↓ |
|
As usual, we check that at least one pairing is legal (e.g. 1-4, 2-3, 5-6, 7-8, 9- 11, 10-12, 13-14), then we separate the scoregroups:
| 4.0 | {2Bb↑, 5Bb↓↓} |
| 3.5 | {1Ww↓} |
| 3.0 | {4B-↑, 6w, 7W-↓} |
| 2.5 | {10Ww, 11w-↑, 14 b↓} |
| 2.0 | {3Bb, 8Ww-↑} |
| 1.5 | {9Bb-↓} |
| 1.0 | {13B-↑} |
| 0.5 | {12W↑↓} |
First, we want to remember that this is the final round – hence, it is possible that some topscorers [A.7], as well as their opponents, may have their absolute colour preference disregarded [A.6].
Now, we must observe that players #1, #2 and #5 have all played with each other, so there is no meaning in trying to pair them – they must all float into the third scoregroup, forming the heterogeneous bracket:
[2Bb↑, 5Bb↓↓, 1Ww↓][4B-↑, 6w, 7W-↓] (MaxPairs=3, M0=M1=3, x=0, z=0)
S1- 2Bb↑ 5Bb↓↓ 1Ww↓
S2- 4B-↑ 6w 7W-↓
Here, the only possible opponent for #2 is #4; since, as we know, player #5 did already play with #1, there are only two possible candidates. The first generated one is:
This candidate is apparently far from perfect. Actually, it fails for colour matching (both for all preferences [C.10] and for strong preferences [C.11]) on two pairs.
It also fails on upfloaters protection [C.19] on pair 2-4 – the latter because the score difference between players #2 and #4 is not minimum (player #2 is a double-floater in this bracket, so #4 is too). Finally, we might also register the fact that #4 is now getting an upfloat for the second time running, thus failing on [C.15]. However, since the pairing 2-4 is the only possible one for player #2, all possible candidates will fail on these four particular criteria ([C.10], [C.11], [C.15], [C.19]), which therefore cannot help us find the best candidate.
S1- 2Bb↑ 5Bb↓↓ 1Ww↓
S2- 4B-↑ 7W-↓ 6w
Anyway, since the candidate is legal, we store it as provisional-best, and proceed to build and evaluate the next (and only other) possible one, which is:
The evaluation of this candidate shows that it fails for [C.10] on the first and third pair, just as the previous one above, but now we have only one failure for
[C.11]. Therefore, we discard the previous candidate and keep the current one, which, although not perfect, is better. Since this is also the last possible legal candidate, we choose it and thus obtain the pairing (2-4, 7-5, 1-6).
We must now perform the completion test; the rest is now {10Ww, 11w, 14b, 3Bb, 8Ww, 9Bb, 13B, 12W}, which allows at least one possible pairing (e.g., 10-11, 13-3, 9-12, 8-14), so the Requirement Zero is satisfied.
The next bracket is {10Ww, 11w-↑, 14b↓}, and #10 already played with #14.
The first candidate (10-11, 14 to float) is legal but fails for C.10 and for C.12 – as usual, we store it as provisional-best. The second candidate is not even legal (because of 10-14) and we discard it straight away. An exchange lends us the next possible candidate, which is (11-14, 10 to float), which is perfect and is therefore immediately accepted.
We must now check that player #10, as a downfloater, maximises the pairing in the next bracket [C.7], which is readily made, because in the bracket [10][3, 8] we can actually build one pair. The check for the Requirement Zero is successful (e.g. 3-13, 8-10, 9-12) and we may proceed to the next bracket, which is [10Ww][3Bb, 8Ww-↑]. The game 10-3 already took place – therefore 10-8 is the only possible MDP-pairing, with an empty pairing of the remainder and with player #3 getting a downfloat. We check that this floater maximises the next bracket (it does) and that the rest is pairable (it is).
We thus get to the next bracket, which is [3Bb][9Bb-↓]. Once again, we have only one possible pairing, which is 9-3. We must check that the rest is pairable
– and, big surprise, we find that it is not (in the rest, there are now only two players, #12 and #13, and they already played each other), so we have a Requirement Zero failure. The current bracket (that is, the one we just paired) is now the PPB, and must give the floaters needed – we have no choice but to make players #3 and #9 float down. Hence, the pairing in the PPB produces no pairs at all, and we obtain the CLB: [3Bb, 9Bb-↓][13B-↑, 12W↑↓]
Now, we can be sure that at least one pairing exists for this bracket, because of the success of the completion test on the previous bracket. Actually, player #13 already played with #9 and #12. Hence, the pairing 3-13 is unavoidable, and this only leaves room for 12-9 as the other pair. We now make the usual checks on
the pairings and their order, then... Ladies and gentlemen, please start clocks for the final round!
| 1 | 2 (4.0) - 4 (3.0) | ½-½ |
| 2 | 7 (3.00) - 5 (4.0) | ½-½ |
| 3 | 1 (3.5) - 6 (3.0) | 1-0 |
| 4 | 11 (2.5) - 14 (2.5) | 0-1 |
| 5 | 10 (2.5) - 8 (2.0) | 0-1 |
| 6 | 3 (2.0) - 13 (1.0) | 1-0 |
| 7 | 12 (0.5) - 9 (1.5) | ½-½ |
- FINAL STEPS
Now the tournament is over. The final operations, with regard to pairing, consist of the harvesting of results and final compilation of the tournament board.
| Player | PN | 1 | 2 | 3 | 4 | 5 | 6 | ||||||
| Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | Pair | Pnts | ||
| Alice | 1 | 8W+ | 1.0 | 5B- | 1.0 | 14B+ | 2.0 | 3W+ | 3.0 | 2B↓= | 3.5 | 6W+ | 4.5 |
| Bruno | 2 | 9B+ | 1.0 | 7W+ | 2.0 | 5W= | 2.5 | 6B+ | 3.5 | 1W↑= | 4.0 | 4W= | 4.5 |
| Carla | 3 | 10W+ | 1.0 | 6B= | 1.5 | 4W= | 2.0 | 1B- | 2.0 | 7W- | 2.0 | 13W+ | 3.0 |
| David | 4 | 11B= | 0.5 | 9W↓+ | 1.5 | 3B= | 2.0 | 5W↑= | 2.5 | 6W= | 3.0 | 2B= | 3.5 |
| Eloise | 5 | 12W+ | 1.0 | 1W+ | 2.0 | 2B= | 2.5 | 4B↓= | 3.0 | 11W↓+ | 4.0 | 7B= | 4.5 |
| Finn | 6 | 13B+ | 1.0 | 3W= | 1.5 | +000↓ | 2.5 | 2W- | 2.5 | 4B= | 3.0 | 1B- | 3.0 |
| Giorgia | 7 | 14W+ | 1.0 | 2B- | 1.0 | 10W+ | 2.0 | 11B↓- | 2.0 | 3B+ | 3.0 | 5W= | 3.5 |
| Kevin | 8 | 1B- | 0.0 | 11W↑- | 0.0 | 12B= | 0.5 | 9W↑= | 1.0 | 13B+ | 2.0 | 10B+ | 3.0 |
| Louise | 9 | 2W- | 0.0 | 4B↑- | 0.0 | 13W+ | 1.0 | 8B↓= | 1.5 | 10W- | 1.5 | 12B= | 2.0 |
| Mark | 10 | 3B- | 0.0 | 13W+ | 1.0 | 7B- | 1.0 | 14W= | 1.5 | 9B+ | 2.5 | 8W- | 2.5 |
| Nancy | 11 | 4W= | 0.5 | 8B↓+ | 1.5 | -000↓ | 1.5 | 7W↑+ | 2.5 | 5B- | 2.5 | 14W- | 2.5 |
| Oskar | 12 | 5B- | 0.0 | ZPB | 0.0 | 8W= | 0.5 | 13B↓- | 0.5 | 14B↑- | 0.5 | 9W= | 1.0 |
| Patricia | 13 | 6W- | 0.0 | 10B- | 0.0 | 9B- | 0.0 | 12W↑+ | 1.0 | 8W- | 1.0 | 3B- | 1.0 |
| Robert | 14 | 7B- | 0.0 | PAB↓ | 1.0 | 1W- | 1.0 | 10B= | 1.5 | 12W↓+ | 2.5 | 11B+ | 3.5 |
That’s all!
Manual checking of computer pairings
Using the data from a random tournament Check list for pairings of round 6
The pairing program used is Swiss Manager
Explanations of the columns used for checking: Rk = rank Colour = colours in previous rounds
w = white
‐ = black
C = colour in upcoming round D = expected colour
p = floater direction in penultimate round l = floater direction in last round
Cd = colour difference
Sc = same colour in a row
| Checklist for Round 6 | ||||||||||||||
| Rk. | SNo. |
|
Name | Rtg. | Pts | Color | C | D | p | l | Cd | Sc | Opponents |
|
| 1 | 36 |
|
Kouskoutis Georgios- | 2314 | 4½ | -w--w |
|
W |
|
|
-1 | 1 | 4,9,12,35,80 | 0 |
| 2 | 1 | IM | Martirosyan Haik M. | 2570 | 4 | w-w-- |
|
W |
|
+ | -1 | -2 | 10,18,37,46,62 | 0 |
| 3 | 2 | GM | Deac Bogdan-Daniel | 2559 | 4 | -w--w |
|
W | + |
|
-1 | 1 | 6,8,19,32,39 | 0 |
| 4 | 3 | IM | Santos Ruiz Miguel | 2505 | 4 | w-w-w |
|
- | + |
|
1 | 1 | 1,22,26,44,46 | 0 |
| 5 | 6 | IM | Thybo Jesper Sonderg | 2466 | 4 | -w-w- |
|
W |
|
|
-1 | -1 | 17,25,27,47,56 | 0 |
| 6 | 8 | IM | Morozov Nichita | 2461 | 4 | -w-w- |
|
W |
|
|
-1 | -1 | 3,16,27,30,42 | 0 |
| 7 | 12 | FM | Janik Igor | 2418 | 4 | -w-w- |
|
W |
|
|
-1 | -1 | 27,34,54,57,59 | 0 |
| 8 | 63 |
|
Yayloyan Konstantin | 2142 | 4 | w--w- |
|
W | - |
|
-1 | -1 | 3,21,24,53,54 | 0 |
| 9 | 4 | FM | Sorokin Aleksey | 2486 | 3½ | -w-w- |
|
W |
|
|
-1 | -1 | 1,10,17,41,79 | 0 |
| 10 | 7 | IM | Livaic Leon | 2461 | 3½ | w-w-w |
|
- |
|
- | 1 | 1 | 2,9,40,43,58 | 0 |
| 11 | 9 | IM | Plenca Jadranko | 2440 | 3½ | w-w-w |
|
- |
|
|
1 | 1 | 15,28,31,44,53 | 0 |
| 12 | 10 | FM | Vykouk Jan | 2440 | 3½ | -w-w- |
|
W |
|
|
-1 | -1 | 1,32,42,45,72 | 0 |
| 13 | 11 | IM | Costachi Mihnea | 2418 | 3½ | w-w-w |
|
- |
|
|
1 | 1 | 40,44,57,58,67 | 0 |
| 14 | 16 | FM | Haria Ravi | 2398 | 3½ | -w-w- |
|
W |
|
|
-1 | -1 | 17,34,45,51,60 | 0 |
| 15 | 18 | FM | Tica Sven | 2389 | 3½ | -w-w- |
|
W |
|
|
-1 | -1 | 11,27,34,56,61 | 0 |
| 16 | 21 | IM | Sousa Andre Ventura | 2386 | 3½ | w-w-w |
|
- |
|
|
1 | 1 | 6,18,29,49,68 | 0 |
| 17 | 33 |
|
Radovic Janko | 2330 | 3½ | w-w-w |
|
- |
|
|
1 | 1 | 5,9,14,24,73 | 0 |
| 18 | 44 | FM | Lazov Toni | 2289 | 3½ | -w-w- |
|
W |
|
|
-1 | -1 | 2,16,22,23,41 | 0 |
| 19 | 45 | FM | Askerov Marat | 2281 | 3½ | w-w-w |
|
- |
|
|
1 | 1 | 3,26,38,40,69 | 0 |
| 20 | 13 |
|
Drygalov Sergey | 2415 | 3 | w-ww- |
|
- | + |
|
1 | -1 | 28,29,33,44,47 | 0 |
| 21 | 15 | FM | Warmerdam Max | 2399 | 3 | w-w-w |
|
- |
|
|
1 | 1 | 8,26,33,48,67 | 0 |
| 22 | 17 | FM | Haldorsen Benjamin | 2397 | 3 | w-w-- |
|
W |
|
|
-1 | -2 | 4,18,28,29,49 | 0 |
| 23 | 19 | FM | Tomczak Mikolaj | 2387 | 3 | w-w-w |
|
- |
|
|
1 | 1 | 18,32,48,50,67 | 0 |
| 24 | 20 |
|
Stauskas Lukas | 2387 | 3 | -w-w- |
|
W |
|
|
-1 | -1 | 8,17,30,57,63 | 0 |
| 25 | 23 | FM | Haug Johannes | 2379 | 3 | w-w-w |
|
- |
|
|
1 | 1 | 5,48,50,58,62 | 0 |
| 26 | 32 | FM | Tokranovs Dmitrijs | 2334 | 3 | -w-w- |
|
W |
|
|
-1 | -1 | 4,19,21,59,72 | 0 |
| 27 | 39 |
|
Akhvlediani Irakli | 2303 | 3 | w-w-w |
|
- |
|
|
1 |
1 | 5,6,7,15,81 | 0 |
| 28 | 40 | FM | Lopez Mulet Inigo | 2302 | 3 | -w-w- |
|
W |
|
|
-1 |
-1 | 11,20,22,60,76 | 0 |
| 29 | 42 | FM | Dobrovoljc Vid | 2293 | 3 | -w-ww |
|
- |
|
|
1 |
2 | 16,20,22,35,77 | 0 |
| 30 | 51 |
|
Vasiesiu Victor | 2267 | 3 | w-w-w |
|
- |
|
|
1 |
1 | 6,24,53,81,83 | 0 |
| 31 | 52 | FM | Karayev Kanan | 2266 | 3 | -w-w- |
|
W |
|
|
-1 |
-1 | 11,54,65,72,75 | 0 |
| 32 | 53 |
|
Friedland Moshe | 2264 | 3 | w-ww- |
|
- |
|
|
1 |
-1 | 3,12,23,52,66 | 0 |
| 33 | 56 | FM | Jogstad Martin | 2259 | 3 | -w-w- |
|
W |
|
|
-1 |
-1 | 20,21,55,74,76 | 0 |
| 34 | 57 | FM | Sevgi Volkan | 2240 | 3 | w-w-w |
|
- |
|
|
1 |
1 | 7,14,15,36,38 | 0 |
| 35 | 5 | IM | Dragnev Valentin | 2483 | 2½ | w-w-w |
|
- |
|
|
1 |
1 |
1,29,57,64,67 | 0 |
| 36 | 14 | FM | Dolana Andrei-Theodo | 2403 | 2½ | -w-w- |
|
W | + |
|
-1 |
-1 |
34,47,51,61,72 | 0 |
| 37 | 22 | FM | O`donnell Conor | 2383 | 2½ | -w-w- |
|
W |
|
|
-1 |
-1 |
2,47,51,59,71 | 0 |
| 38 | 24 | FM | Flick Antoine | 2371 | 2½ | -w-ww |
|
- |
|
+ | 1 |
2 |
19,34,60,63,64 | 0 |
| 39 | 28 | IM | Perez Garcia Alejand | 2361 | 2½ | -ww-w |
|
- |
|
|
1 |
1 |
3,49,51,68,71 | 0 |
| 40 | 30 | FM | Lagunow Raphael | 2357 | 2½ | -w-w- |
|
W |
|
|
-1 |
-1 |
10,13,19,65,74 | 0 |
INTERNATIONAL TITLE REGULATIONS
As approved by the 1982 General Assembly and amended by the General Assemblies of 1984 through 2016, effective from 1 July 2017.
- Introduction
-
- Only the titles as in 0.3 are acknowledged by FIDE.
- The following regulations can only be altered by the General Assembly following recommendation by the Qualification Commission (QC).
-
- Any such changes shall only be made every fourth year, commencing from 2004 (unless the Commission agrees urgent action is required).
- Any such changes shall take effect from 1 July of the year following the decision by the General Assembly. For tournaments, such changes shall apply to those starting on or after that date.
-
- The International FIDE titles shall be under the umbrella of the Qualification Commission, which is the final judging unit. The titles are:
0.31 Titles for over-the-board standard chess (as defined in the Laws of Chess), the judging unit being the QC:
Grandmaster (GM), International Master (IM), FIDE Master (FM), Candidate Master (CM), Woman Grandmaster (WGM), Woman International Master (WIM), Woman FIDE Master (WFM), Woman Candidate Master (WCM).
-
- The titles are valid for life from the date confirmed.
-
- Use of a FIDE title or rating to subvert the ethical principles of the title or rating system may subject a person to revocation of his title upon recommendation by the Qualification and Ethics Commissions and final action by the General Assembly.
- A title is officially valid from the date all the requirements are met. In order for a title to be confirmed where it is based on an application, it must be published on the FIDE website and in other relevant FIDE documents for at least 60 days. For registered automatic titles see below, 0.5.
- The title can be used for results of opponents only in tournaments starting after the confirmation. (exception: see 1.15).
- In terms of, for example, the age of achieving a title, the title is considered to be achieved when the last result is achieved, and the rating requirement is fulfilled, whichever date is later.
-
- In case it is found after a title has been awarded that the player was in breach of the Anti-Cheating Regulations in one or more of the tournaments on which the title application was based, then the title may be removed by the Qualification Commission. The federation of this player may appeal this decision to FIDE Presidential Board within 30 days after they had been informed in writing.
-
- Definitions
In the following text some special terms are used.
Rating performance is based on the player’s result and average rating of opponents (see 1.48).
Title performance (for example, GM performance) is a result that gives a performance rating as defined in 1.48 and 1.49 against the minimum average of the opponents, taking into account article 1.46, for that title. For example, for GM performance, average rating of the opponents ≥2380, and performance ≥2600, this might be achieved, for example, by a result of 7 points out of 9 games.
GM performance is ≥ 2600 performance against opponents with average rating ≥ 2380.
IM performance is ≥ 2450 performance against opponents with average rating ≥ 2230.
WGM performance is ≥ 2400 performance against opponents with average rating ≥ 2180.
WIM performance is ≥ 2250 performance against opponents with average rating ≥ 2030.
Title norm is a title performance fulfilling additional requirements concerning the mix of titled players and nationalities as specified in articles 1.42 to 1.47.
Direct title (automatic title) is a title gained by achieving a certain place or result in a tournament. For example, winning, or achieving a result ≥50 percent in a tournament. On application by the player’s federation and confirmation by the Qualification Commission, such titles are awarded automatically by FIDE.
-
- The Award of Titles
-
- Titles may be awarded for specific results in specific Championship events, or are awarded on achieving a rating as laid down in these regulations. Such titles are confirmed by the QC Chairman on advice from the FIDE Office. They are then awarded by FIDE.
- For a direct title to be awarded immediately an applicant has to have achieved at some time or other a minimum rating as follows:
| GM | 2300 | WGM | 2100 |
| IM | 2200 | WIM | 2000 |
| FM | 2100 | WFM | 1900 |
CM 2000 WCM 1800
If an applicant is rated lower the title is awarded conditionally and will be awarded finally on request by the respective federation as soon as the minimum rating is achieved. Any player with a conditional title may take a lower title when they reach the required rating for that lower title.
-
- Titles are also awarded based on applications with norms with a sufficient number of games. These titles shall be awarded by the General Assembly on recommendation by the QC that the candidate meets the requirements. The Presidential Board or Executive Board may award titles in clear cases only, after consultation with the QC.
- Requirements for titles designated in 0.31
-
- Administration
-
- Play shall be governed by the FIDE Laws of Chess. Tournaments where the composition is changed (without QC approval) during the tournament or those where players have different conditions in terms of rounds and pairing are not valid. Unless with prior approval of the QC Chairman, the tournament must be registered at least 30 days in advance on the FIDE server.
- There must be no more than twelve hours play in one day. This is calculated based on games that last 60 moves, although games played using increments may last longer.
- No more than 2 rounds shall be played on any one day.
With an increment of a minimum of 30 seconds for each move, the minimum time is 90 minutes for the entire game for each player, apart from the increment.
Without an increment the minimum playing time is 2 hours for each player. From 1 July 2021 games played without an increment of at least 30 seconds per move are not valid for titles or title norms, except in the case of disabled players.
1.13a In the application for the GM title based on norms, at least one norm shall be achieved in a tournament with only one round per day for a minimum of 3 days.
-
- b In any title tournament the time controls and clock settings for all players must be the same, except as defined in Appendix G.4 of the Laws of Chess (e.g. if the time control is increment based, all players must use increment; if delay based, all players must use delay; if no increment or delay is specified, then all players must compete with no increment and no delay). There can be no mixed use of clock settings (increment, delay, none at all).
-
- Leagues and national team championships may last longer than 90 days, but not more than one year. Normally for individual tournaments, a period of at most 90 days is permitted but the QC Chairman may give prior approval to tournaments of a longer duration.
- In tournaments which last longer than 90 days, the opponents’ ratings and titles used shall be those applying when the games were played.
- The Chief Arbiter of a title tournament shall be an International Arbiter (IA) or FIDE Arbiter (FA). He may appoint a temporary deputy. An IA or FA must always be in the playing venue.
- No appointed arbiter may play in a title tournament even just as a filler.
|
It is also advisable no appointed arbiter to play in a FIDE rated tournament even just as a filler. |
-
- Titles achieved from International Championships:
-
- As indicated below, a player may gain
- a title from such an event, or
- a single title norm. Then the requirements in 1.42 - 1.49 shall apply.
- The minimum score is 35 % for all titles. The result shown is the minimum required.
- For continental, sub-continental or approved competitions of FIDE International Affiliates, a title or result can be achieved if at least one third or five of the appropriate member federations – whichever is lower – participate in the event. The minimum number of participants in the event is eight. The World Championships (including U20) of the IBCA, ICSC and IPCA are exempted from this rule.
-
- a If groups are combined to make a bigger group, then the requirements (at least 8 participants from at least 5 federations) in 1.23 shall apply to this merged group. Titles can be awarded to the best player(s) of the subgroups, provided the subgroup has at least 5 participants from at least 3 federations and the player scores a minimum of 50% in a minimum of 9 games.
- Terms used in Tables 1.24a and 1.24b:
Gold = first after tiebreak;
1st equal = best 3 players after tiebreak; norm = 9 games (unless otherwise specified);
Continental and Regional = Continental and a maximum 3 regional events per continent, and include Arab Youth
Sub-Continentals - include Zonals, Sub-zonals and Arab adult.
Zonals and Sub-zonals are accepted for direct titles only if they establish qualifiers to World Cup or World Championship.
Each continent is allowed to designate a maximum of 3 regional youth/school events for direct titles. The Continent must inform QC of the composition of these regions before the beginning of each year.
-
- Titles may be gained by achieving a published or interim rating at some time or other (see 1.53a) having at that time played at least 30 rated games:
-
- FIDE Master ≥2300
- Candidate Master ≥2200
- Women FIDE Master ≥2100
- Women Candidate Master ≥2000
-
- The GM, IM, WGM, WIM titles can also be gained by achieving norms in internationally rated tournaments played according to the following regulations.
1.41 The number of games
1.41a The player must play at least 9 games, however
1.41b only 7 games are required for 7 round World Team or Club and Continental Team or Club Championships,
only 7 games are required for 8 or 9 round World Team or Club and Continental Team or Club Championships,
only 8 games are required for the World Cup or Women`s World Championship Tournament, where these 8 game norms count as 9 games.
1.41c For a 9 round tournament, if a player has just 8 games because of a forfeit or Bye, but he has met the correct mix of opponents in those games, then if he has a title result in 8 games, it counts as an 8 game norm. d Where a player exceeds the norm requirements by one or more full points, then these full points count as additional number of games when computing the total number of games for the norm achieved.
1.42a Games against opponents who do not belong to FIDE federations. Players with federation “FID” are accepted, but do not count as a foreign player.
1.42b Games against computers.
1.42c Games against unrated players who score zero against rated opponents in round robin tournaments.
1.42d Games which are decided by forfeit, adjudication or any means other than over the board play. Other games once started, which are forfeited for whatever reason, shall however be included. In the instance of a last round game where the opponent forfeits, the norm shall still count if the player must play in order to have the required number of games, but can afford to lose.
1.42e A player who has achieved a title result before the last round may ignore all games played subsequently, provided
- he has met the required mix of opponents,
- this leaves him with at least the minimum number of games as in 1.41,
- in the case of a tournament with pre-determined pairings, the mix of opponents must be such that a norm is possible for the complete tournament.
- in a double round robin tournament, the games counted for the norm must include different opponents sufficient for a norm over the full length of the tournament.
1.42f A player may ignore his game(s) against any opponents he has defeated, provided he has met the required mix of opponents, and provided that this leaves him with at least the minimum number of games as in 1.41, against the required mix of opponents. Nonetheless, the full cross-table of the event must be submitted. In the case of a tournament with pre-determined pairings, the full requirements, other than score, must be met for the complete tournament.
-
- g Tournaments that make changes to favour one or more players (for example by altering the number of rounds, or the order of rounds, or providing particular opponents, not otherwise participating in the event), shall be excluded.
- Federations of opponents
At least two federations other than that of the title applicant must be included, except 1.43a - 1.43e shall be exempt. Nevertheless, 1.43f shall apply.
1.43a The final stage of the national men’s (or open) championship and also national women’s championships. In the year when the Subzonal tournament of a single federation is held, then the national championship is not exempt for that federation. This exemption applies only to players from the federation which registers the event.
1.43b National team championships. This exemption applies only to players from the federation which registers the event. Results from different divisions may not be combined.
1.43c Zonal and Subzonal tournaments.
1.43d Tournaments of other types may also be included with the prior approval of the QC Chairman.
1.43e Swiss System tournaments in which participants include in every round at least 20 FIDE rated players participate, not from the host federation, from at least 3 different federations, at least 10 of whom hold GM, IM, WGM or WIM titles. Otherwise 1.44 applies.
-
- f At least one of the norms has to be achieved under normal foreigner requirement. (See 1.43 and 1.44)
- A maximum of 3/5 of the opponents may come from the applicant’s federation and a maximum of 2/3 of the opponents from one federation. For exact numbers see the table in 1.72.
Opponents shall be calculated using rounding up (minimum) to the next whole number, to the next lower number (maximum).
-
- Titles of opponents
1.45a At least 50% of the opponents shall be title-holders (TH) as in 0.31, excluding CM and WCM.
1.45b For a GM norm at least 1/3 with a minimum 3 of the opponents (MO) must be GMs.
1.45c For an IM norm at least 1/3 with a minimum 3 of the opponents (MO) must be IMs or GMs.
1.45d For a WGM norm at least 1/3 with a minimum 3 of the opponents (MO) must be WGMs, IMs or GMs.
1.45e For a WIM norm at least 1/3 with a minimum 3 of the opponents (MO) must be WIMs, WGMs, IMs or GMs.
-
- f Double round-robin tournaments need a minimum of 6 players. An opponent’s title as in 1.45b-e shall be counted only once.
- Rating of opponents
1.46a The Rating List in effect at the start of the tournament shall be used, see exception 1.15. The rating of players who belong to federations which are temporarily excluded when the tournament starts can be determined on application to the FIDE Office.
1.46b For the purposes of norms, the minimum rating (adjusted rating floor) for the opponents shall be as follows:
Grandmaster 2200
International Master 2050
Woman Grandmaster 2000
Woman International Master 1850
1.46c No more than one opponent shall have his rating raised to this adjusted rating floor. Where more than one opponent are below the floor, the rating of the lowest opponents shall be raised.
-
- d Unrated opponents not covered by 1.46b shall be considered to be rated 1000. Minimum number of rated opponents, see table in 1.72. It can be calculated also so that maximum number of unrated opponents is 20 percent of (number of opponents+1).
- Rating average of opponents
1.47a This is the total of the opponents’ ratings divided by the number of opponents taking 1.46c into account.
-
- b Rounding of the rating average is made to the nearest whole number. The fraction 0.5 is rounded upward.
- Performance Rating (Rp)
In order to achieve a norm, a player must perform at a level at least of that shown below:
|
|
Minimum level prior to rounding | Minimum level after rounding |
| GM | 2599.5 | 2600 |
| IM | 2449.5 | 2450 |
| WGM | 2399.5 | 2400 |
| WIM | 2249.5 | 2250 |
Calculation of a Performance Rating (Rp):
Rp = Ra + dp (see the table below)
Ra = Average rating of opponents + rating difference „dp“ from table 8.1a of FIDE Rating Regulations B.02 (conversion from percentage score „p“ into rating differences „dp“).
-
- a The minimum average ratings Ra of the opponents are as follows:
GM 2380; IM 2230; WGM 2180; WIM 2030.
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
All percentages are rounded to the nearest whole number. 0.5% is rounded up.
-
- Requirements for award of the title, having achieved norms
-
- Two or more norms in events covering at least 27 games, except that if one norm is an 8 game norm as the result of a win by forfeit, then 26 games is sufficient.
- If a norm is sufficient for more than one title, then it may be used as part of the application for both.
- To have achieved at some time or other a rating as follows: GM≥2500 IM≥2400 WGM ≥2300 WIM≥2200
-
- a Such a rating need not be published. It can be obtained in the middle of a rating period, or even in the middle of a tournament. The player may then disregard subsequent results for the purpose of their title application. However the burden of proof then rests with the federation of the title applicant. It is recommended that players receive a certificate from the Chief Arbiter where they achieve the rating level during an event. Such a certificate should include a note of the date each game was played. Title applications based on unpublished ratings shall only be accepted by FIDE after agreement with the Rating Administrator and the QC. Ratings in the middle of a period can be confirmed only after all tournaments for that period have been received and rated by FIDE.
- A title result shall be valid if it was obtained in accordance with the FIDE Title Regulations prevailing at the time of the tournament when the norm was obtained.
- Title norms gained before 1.7.2005 must have been registered with FIDE before 31.7.2013 or they will be considered to have expired.
-
- Summary of Title Tournaments Requirements
In the case of any discrepancy, the regulations above shall take precedence.
|
|
|
Notes |
| Number of games per day | not more than 2 | 1.13 |
| rate of play | minimum requirements | 1.13 |
| period for the whole tournament | within 90 days, with exceptions | 1.14 |
| administrator in charge | International Arbiter or FIDE Arbiter | 1.16 |
| number of games | minimum 9 (7 in World/Continental Teams with 7-9 rounds) | 1.41a-d |
| type of tournament | No individual single matches | 1.1 |
| games not Included |
against computers adjudicated games forfeited before play starts against opponents who do not belong to FIDE federations |
1.42 |
-
-
- For the numbers below, see the formula calculating titles in 1.45.
-
|
|
|
Notes |
| Number of GMs, for GM MO | 1/3 of opponents, minimum 3 GMs | 1.45b |
| Number of IMs, for IM MO | 1/3 of opponents, minimum 3 IMs | 1.45c |
| Number of WGMs, for WGM MO | 1/3 of opponents, minimum 3 WGMs | 1.45d |
| Number of WIMs, for WIM MO | 1/3 of opponents, minimum 3 WIMs | 1.45e |
| Minimum Performance Rating | GM 2600; IM 2450; WGM 2400; WIM 2250 | 1.48 |
| Opponents’ minimum average rating | 2380 for GM; 2230 for IM; 2180 for WGM; 2030 for WIM | 1.7 |
| Minimum score | 35% | 1.7 |
-
- Summary of Requirements for the Number of Opponents
-
- Determining whether a result is adequate for a norm, dependent on the average rating of the opponents. Tables 1.72 show the range for tournaments up to 19 rounds. Norms achieved in a tournament with more than 13 rounds count only as 13 games.
- Tables
Available only for 7 to 9 round Continental and World Team Championships
| 7 rounds | GM | IM | WGM | WIM |
| Different MO | 3 GM | 3 IM | 3 WGM | 3 WIM |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
| Different TH | 4 | 4 | 4 | 4 |
| Max. number unrated | 1 | 1 | 1 | 1 |
| Max. from 1 fed. | Irrelevant | Irrelevant | Irrelevant | Irrelevant |
| Max. from own fed. | Irrelevant | Irrelevant | Irrelevant | Irrelevant |
| Min. other feds. | Irrelevant | Irrelevant | Irrelevant | Irrelevant |
| 5½ | 2380-2441 | 2230-2291 | 2180-2241 | 2030-2091 |
| 5 | 2442-2497 | 2292-2347 | 2242-2297 | 2092-2147 |
| 4½ | 2498-2549 | 2348-2399 | 2298-2349 | 2148-2199 |
| 4 | 2550-2599 | 2400-2449 | 2350-2399 | 2200-2249 |
| 3½ | 2600-2649 | 2450-2499 | 2400-2449 | 2250-2299 |
| 3 | 2650-2701 | 2500-2551 | 2450-2501 | 2300-2351 |
| 2½ | ≥2702 | ≥2552 | ≥2502 | ≥2352 |
Available only for 8 or 9 round Continental and World Team Championships; or after 8 games in the World Cup or Women’s World Championship. The latter two are counted as 9 rounds when computing to 27 games.
| 8 rounds | GM | IM | WGM | WIM |
| Different MO | 3 GM | 3 IM | 3 WGM | 3 WIM |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
| Different TH | 4 | 4 | 4 | 4 |
| Max. number unrated | 1 | 1 | 1 | 1 |
| Max. from 1 fed. | Irrelevant | Irrelevant | Irrelevant | Irrelevant |
| Max. from own fed. | Irrelevant | Irrelevant | Irrelevant | Irrelevant |
| Min. other feds | Irrelevant | Irrelevant | Irrelevant | Irrelevant |
| 6½ | 2380-2406 | 2230-2256 | 2180-2206 | 2030-2056 |
| 6 | 2407-2458 | 2257-2308 | 2207-2258 | 2057-2108 |
| 5½ | 2459-2504 | 2309-2354 | 2259-2304 | 2109-2154 |
| 5 | 2505-2556 | 2355-2406 | 2305-2356 | 2155-2206 |
| 4½ | 2557-2599 | 2407-2449 | 2357-2399 | 2207-2249 |
| 4 | 2600-2642 | 2450-2492 | 2400-2442 | 2250-2292 |
| 3½ | 2643-2686 | 2493-2536 | 2443-2486 | 2293-2336 |
| 3 | ≥2687 | ≥2537 | ≥2487 | ≥2337 |
The material following refers to 9-19 rounds:
* The regulations regarding mix of federations as in the boxes marked * are waived if the event is a Swiss System tournament in which the competitors include at least 20 FIDE Rated players, not from the host federation, from at least 3 federations, at least 10 of whom hold GM, IM, WGM or WIM titles. See 1.46c concerning the rating floor of the lowest rated opponent.
| 9 rounds | GM | IM | WGM | WIM |
| Different MO | 3 GM | 3 IM | 3 WGM | 3 WIM |
| *Min. other feds. | 2 | 2 | 2 | 2 |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
| Different TH | 5 | 5 | 5 | 5 |
| Max. number unrated | 2 | 2 | 2 | 2 |
| *Max. from 1 fed. | 6 | 6 | 6 | 6 |
| *Max. from own fed. | 5 | 5 | 5 | 5 |
| 7 | 2380-2433 | 2230-2283 | 2180-2233 | 2030-2083 |
| 6½ | 2434-2474 | 2284-2324 | 2234-2274 | 2084-2124 |
| 6 | 2475-2519 | 2325-2369 | 2275-2319 | 2125-2169 |
| 5½ | 2520-2556 | 2370-2406 | 2320-2356 | 2170-2206 |
| 5 | 2557-2599 | 2407-2449 | 2357-2399 | 2207-2249 |
| 4½ | 2600-2642 | 2450-2492 | 2400-2442 | 2250-2292 |
| 4 | 2643-2679 | 2493-2529 | 2443-2479 | 2293-2329 |
| 3½ | ≥2680 | ≥2530 | ≥2480 | ≥2330 |
For 10 rounds or more it is possible that deleting a game that has been won could be advantageous.
SR refers to single round and DR to double round events.
| 10 rounds | GM | IM | WGM | WIM | ||||
| Different MO | 4 GM | 4 IM | 4 WGM | 4 WIM | ||||
| *Min. other feds. | 2 | 2 | 2 | 2 | ||||
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 | ||||
|
|
SR | DR | SR | DR | SR | DR | SR | DR |
| Different TH | 5 | 3 | 5 | 3 | 5 | 3 | 5 | 3 |
| Max. number unrated | 2 | 1 | 2 | 1 | 2 | 1 | 2 | 1 |
| *Max. from 1 fed. | 6 | 3 | 6 | 3 | 6 | 3 | 6 | 3 |
| *Max. from own fed. | 6 | 3# | 6 | 3# | 6 | 3# | 6 | 3# |
| 8 | 2380-2406 | 2230-2256 | 2180-2206 | 2030-2056 | ||||
| 7½ | 2407-2450 | 2257-2300 | 2207-2250 | 2057-2100 | ||||
| 7 | 2451-2489 | 2301-2339 | 2251-2289 | 2101-2139 | ||||
| 6½ | 2490-2527 | 2340-2377 | 2290-2327 | 2140-2177 | ||||
| 6 | 2528-2563 | 2378-2413 | 2328-2363 | 2178-2213 | ||||
| 5½ | 2564-2599 | 2414-2449 | 2364-2399 | 2214-2249 | ||||
| 5 | 2600-2635 | 2450-2485 | 2400-2435 | 2250-2285 | ||||
| 4½ | 2636-2671 | 2486-2521 | 2436-2471 | 2286-2321 | ||||
| 4 | 2672-2709 | 2522-2559 | 2472-2509 | 2322-2359 | ||||
| 3½ | ≥2710 | ≥2560 | ≥2510 | ≥2360 | ||||
#If there were 4 players from 1 federation out of the 6 contestants, neither of the other 2 players would be able to gain a title norm. This would be satisfactory if, for example, both were GMs.
| 11 rounds | GM | IM | WGM | WIM |
| Different MO | 4 GM | 4 IM | 4 WGM | 4 WIM |
| *Min. Other feds. | 2 | 2 | 2 | 2 |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
| Different TH | 6 | 6 | 6 | 6 |
| Max. number unrated | 2 | 2 | 2 | 2 |
| *Max. from 1 fed. | 7 | 7 | 7 | 7 |
| *Max. from own fed. | 6 | 6 | 6 | 6 |
| 9 | 2380-2388 | 2230-2238 | 2180-2188 | 2030-2038 |
| 8½ | 2389-2424 | 2239-2274 | 2189-2224 | 2039-2074 |
| 8 | 2425-2466 | 2275-2316 | 2225-2266 | 2075-2116 |
| 7½ | 2467-2497 | 2317-2347 | 2267-2297 | 2117-2147 |
| 7 | 2498-2534 | 2348-2384 | 2298-2334 | 2148-2184 |
| 6½ | 2535-2563 | 2385-2413 | 2335-2363 | 2185-2213 |
| 6 | 2564-2599 | 2414-2449 | 2364-2399 | 2214-2249 |
| 5½ | 2600-2635 | 2450-2485 | 2400-2435 | 2250-2285 |
| 5 | 2636-2664 | 2486-2514 | 2436-2464 | 2286-2314 |
| 4½ | 2665-2701 | 2515-2551 | 2465-2501 | 2315-2351 |
| 4 | ≥2702 | ≥2552 | ≥2502 | ≥2352 |
SR refers to single round and DR to double round events.
| 12 rounds | GM | IM | WGM | WIM | ||||
| Different MO | 4 GM | 4 IM | 4 WGM | 4 WIM | ||||
| *Min. Other feds. | 2 | 2 | 2 | 2 | ||||
| Rating floor for - 1 player | 2200 | 2050 | 2000 | 1850 | ||||
|
|
SR | DR | SR | DR | SR | DR | SR | DR |
| Different TH | 6 | 3 | 6 | 3 | 6 | 3 | 6 | 3 |
| Max. number unrated | 2 | 1 | 2 | 1 | 2 | 1 | 2 | 1 |
| *Max. from 1 fed. | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 |
| *Max. from-own fed. | 7 | 3# | 7 | 3# | 7 | 3# | 7 | 3# |
| 9½ | 2380-2406 | 2230-2256 | 2180-2206 | 2030-2056 | ||||
| 9 | 2407-2441 | 2257-2291 | 2207-2241 | 2057-2091 | ||||
| 8½ | 2442-2474 | 2292-2324 | 2242-2274 | 2092-2124 | ||||
| 8 | 2475-2504 | 2325-2354 | 2275-2304 | 2125-2154 | ||||
| 7½ | 2505-2542 | 2355-2392 | 2305-2342 | 2155-2192 | ||||
| 7 | 2543-2570 | 2393-2420 | 2343-2370 | 2193-2220 | ||||
| 6½ | 2571-2599 | 2421-2449 | 2371-2399 | 2221-2249 | ||||
| 6 | 2600-2628 | 2450-2478 | 2400-2428 | 2250-2278 | ||||
| 5½ | 2629-2656 | 2479-2506 | 2429-2456 | 2279-2306 | ||||
| 5 | 2657-2686 | 2507-2536 | 2457-2486 | 2307-2336 | ||||
| 4½ | ≥2687 | ≥2537 | ≥2487 | ≥2337 | ||||
# If there were 4 players from 1 federation out of the 7 contestants, none of the other 3 players would be able to gain a title norm. This would be satisfactory if, for example, all were GMs.
| 13 rounds | GM | IM | WGM | WIM |
| Different MO | 5 GM | 5 IM | 5 WGM | 5 WIM |
| *Min. other feds. | 2 | 2 | 2 | 2 |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
| Different TH | 7 | 7 | 7 | 7 |
| Max. number unrated | 2 | 2 | 2 | 2 |
| *Max. from 1 fed. | 8 | 8 | 8 | 8 |
| *Max. from own fed. | 7 | 7 | 7 | 7 |
| 10½ | 2380-2388 | 2230-2238 | 2180-2188 | 2030-2038 |
| 10 | 2389-2424 | 2239-2274 | 2189-2224 | 2039-2074 |
| 9½ | 2425-2458 | 2275-2308 | 2225-2258 | 2075-2108 |
| 9 | 2459-2489 | 2309-2339 | 2259-2289 | 2109-2139 |
| 8½ | 2490-2512 | 2340-2362 | 2290-2312 | 2140-2162 |
| 8 | 2513-2542 | 2363-2392 | 2313-2342 | 2163-2192 |
| 7½ | 2543-2570 | 2393-2420 | 2343-2370 | 2193-2220 |
| 7 | 2571-2599 | 2421-2449 | 2371-2399 | 2221-2249 |
| 6½ | 2600-2628 | 2450-2478 | 2400-2428 | 2250-2278 |
| 6 | 2629-2656 | 2479-2506 | 2429-2456 | 2279-2306 |
| 5½ | 2657-2686 | 2507-2536 | 2457-2486 | 2307-2336 |
| 5 | ≥2687 | ≥2537 | ≥2487 | ≥2337 |
| 14 rounds - counts as 13 rounds | GM | IM | WGM | WIM | ||||
| Different MO | 5 GM | 5 IM | 5 WGM | 5 WIM | ||||
| Min. other feds. | 2 | 2 | 2 | 2 | ||||
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 | ||||
|
|
SR | DR | SR | DR | SR | DR | SR | DR |
| Different TH | 7 | 4 | 7 | 4 | 7 | 4 | 7 | 4 |
| *Max unrated | 3 | 1 | 3 |
1 |
3 | 1 | 3 | 1 |
| *Max. from 1 fed. | 9 | 4 | 9 | 4 | 9 | 4 | 9 | 4 |
| *Max. from own fed. | 8 | 4# | 8 | 4# | 8 | 4# | 8 | 4# |
| 11 | 2380-2406 | 2230-2256 | 2180-2206 | 2030-2056 | ||||
| 10½ | 2407-2441 | 2257-2291 | 2207-2241 | 2057-2091 | ||||
| 10 | 2442-2466 | 2292-2316 | 2242-2266 | 2092-2116 | ||||
| 9½ | 2467-2497 | 2317-2347 | 2267-2297 | 2117-2147 | ||||
| 9 | 2498-2519 | 2348-2369 | 2298-2319 | 2148-2169 | ||||
| 8½ | 2520-2549 | 2370-2399 | 2320-2349 | 2170-2199 | ||||
| 8 | 2550-2570 | 2400-2420 | 2350-2370 | 2200-2220 | ||||
| 7½ | 2571-2599 | 2421-2449 | 2371-2399 | 2221-2249 | ||||
| 7 | 2600-2628 | 2450-2478 | 2400-2428 | 2250-2278 | ||||
| 6½ | 2629-2649 | 2479-2499 | 2429-2449 | 2279-2299 | ||||
| 6 | 2650-2679 | 2500-252 | 2450-2479 | 2300-2329 | ||||
| 5½ | 2680-2701 | 2530-2551 | 2480-2501 | 2330-2351 | ||||
| 5 | ≥2702 | ≥2552 | ≥2502 | ≥2352 | ||||
# If there were 5 players from 1 federation out of the 8 contestants, none of the other 3 players would be able to gain a title norm. This would be fine if, for example, all were GMs.
| 15 rounds - counts as 13 rounds | GM | IM | WGM | WIM |
| Different MO | 5 GM | 5 IM | 5 WGM | 5 WIM |
| *Min. other feds. | 2 | 2 | 2 | 2 |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
| Different TH | 8 | 8 | 8 | 8 |
| max unrated | 3 | 3 | 3 | 3 |
| Max. from 1 fed. | 10 | 10 | 10 | 10 |
| Max. from own fed. | 9 | 9 | 9 | 9 |
| 12 | 2380-2388 | 2230-2238 | 2180-2188 | 2030-2038 |
| 11½ | 2389-2424 | 2239-2274 | 2189-2224 | 2039-2074 |
| 11 | 2425-2450 | 2275-2300 | 2225-2250 | 2075-2100 |
| 10½ | 2451-2474 | 2301-2324 | 2251-2274 | 2101-2124 |
| 10 | 2475-2504 | 2325-2354 | 2275-2304 | 2125-2154 |
| 9½ | 2505-2527 | 2355-2377 | 2305-2327 | 2155-2177 |
| 9 | 2528-2549 | 2378-2399 | 2328-2349 | 2178-2199 |
| 8½ | 2550-2578 | 2400-2428 | 2350-2378 | 2200-2228 |
| 8 | 2579-2599 | 2429-2449 | 2379-2399 | 2229-2249 |
| 7½ | 2600-2620 | 2450-2470 | 2400-2420 | 2250-2270 |
| 7 | 2621-2649 | 2471-2499 | 2421-2449 | 2271-2299 |
| 6½ | 2650-2671 | 2500-2521 | 2450-2471 | 2300-2321 |
| 6 | 2672-2694 | 2522-2544 | 2472-2494 | 2322-2344 |
| 5½ | ≥2695 | ≥2545 | ≥2495 | ≥2345 |
| 16 rounds - counts as 13 rounds | GM | IM | WGM | WIM | ||||
| Different MO | 6 GM | 6 IM | 6 WGM | 6 WIM | ||||
| Min. other feds. | 2 | 2 | 2 | 2 | ||||
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 | ||||
|
|
SR | DR | SR | DR | SR | DR | SR | DR |
| Different TH | 8 | 4 | 8 | 4 | 8 | 4 | 8 | 4 |
| *Max unrated | 3 | 1 | 3 | 1 | 3 | 1 | 3 | 1 |
| *Max. from 1 fed. | 10 | 5 | 10 | 5 | 10 | 5 | 10 | 5 |
| *Max. from own fed. | 9 | 4 | 9 | 4 | 9 | 4 | 9 | 4 |
| 12½ | 2380-2406 | 2230-2256 | 2180-2206 | 2030-2056 | ||||
| 12 | 2407-2433 | 2257-2283 | 2207-2233 | 2057-2083 | ||||
| 11½ | 2434-2458 | 2284-2308 | 2234-2258 | 2084-2108 | ||||
| 11 | 2459-2482 | 2309-2332 | 2259-2282 | 2109-2132 | ||||
| 10½ | 2483-2504 | 333-2354 | 2283-2304 | 2133-2154 | ||||
| 10 | 2505-2534 | 2355-2384 | 2305-2334 | 2155-2184 |
| 9½ | 2535-2556 | 2385-2406 | 2335-2356 | 2185-2206 |
| 9 | 2557-2578 | 2407-2428 | 2357-2378 | 2207-2228 |
| 8½ | 2579-2599 | 2429-2449 | 2379-2399 | 2229-2249 |
| 8 | 2600-2620 | 2450-2470 | 2400-2420 | 2250-2270 |
| 7½ | 2621-2642 | 2471-2492 | 2421-2442 | 2271-2292 |
| 7 | 2643-2664 | 2493-2514 | 2443-2464 | 2293-2314 |
| 6½ | 2665-2686 | 2515-2536 | 2465-2486 | 2315-2336 |
| 6 | ≥2687 | ≥2537 | ≥2487 | ≥2337 |
| 17 rounds counts as 13 rounds | GM | IM | WGM | WIM |
| Different MO | 6 GM | 6 IM | 6 WGM | 6 WIM |
| *Min. other feds. | 2 | 2 | 2 | 2 |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
| Different TH | 9 | 9 | 9 | 9 |
| Max unrated | 3 | 3 | 3 | 3 |
| Max. from 1 fed. | 11 | 11 | 11 | 11 |
| Max. from own fed. | 10 | 10 | 10 | 10 |
| 13½ | 2380-2397 | 2230-2247 | 2180-2197 | 2030-2047 |
| 13 | 2398-2415 | 2248-2265 | 2198-2215 | 2048-2065 |
| 12½ | 2416-2441 | 2266-2291 | 2216-2241 | 2066-2091 |
| 12 | 2442-2466 | 2292-2316 | 2242-2266 | 2092-2116 |
| 11½ | 2467-2489 | 2317-2339 | 2267-2289 | 2117-2139 |
| 11 | 2490-2512 | 2340-2362 | 2290-2312 | 2140-2162 |
| 10½ | 2513-2534 | 2363-2384 | 2313-2334 | 2163-2184 |
| 10 | 2535-2556 | 2385 2406 | 2335-2356 | 2185-2206 |
| 9½ | 2557-2578 | 2407-2428 | 2357-2378 | 2207-2228 |
| 9 | 2579-2599 | 2429-2449 | 2379-2399 | 2229-2249 |
| 8½ | 2600-2620 | 2450-2470 | 2400-2420 | 2250-2270 |
| 8 | 2621-2642 | 2471-2492 | 2421-2442 | 2271-2292 |
| 7½ | 2643-2664 | 2493-2514 | 2443-2464 | 2293-2314 |
| 7 | 2665-2686 | 2515-2536 | 2465-2486 | 2315-2336 |
| 6½ | ≥2687 | ≥2537 | ≥2487 | ≥2337 |
| 18 rounds - counts as 13 rounds | GM | IM | WGM | WIM |
| Different MO | 6 GM | 6 IM | 6 WGM | 6 WIM |
| Min. other feds. | 2 | 2 | 2 | 2 |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
|
|
SR | DR | SR | DR | SR | DR | SR | DR |
| Different TH | 9 | 5 | 9 | 5 | 9 | 5 | 9 | 5 |
| *Max unrated | 3 | 1 | 3 | 1 | 3 | 1 | 3 | 1 |
| *Max. from 1 fed. | 12 | 6 | 12 | 6 | 12 | 6 | 12 | 6 |
| *Max. from own fed. | 10 | 5 | 10 | 5 | 10 | 5 | 10 | 5 |
| 14 | 2380-2406 | 2230-2256 | 2180-2206 | 2030-2056 | ||||
| 13½ | 2407-2433 | 2257-2283 | 2207-2233 | 2057-2083 | ||||
| 13 | 2434-2458 | 2284-2308 | 2234-2258 | 2084-2108 | ||||
| 12½ | 2459-2474 | 2309-2324 | 2259-2274 | 2109-2124 | ||||
| 12 | 2475-2497 | 2325-2347 | 2275-2297 | 2125-2147 | ||||
| 11½ | 2498-2519 | 2348-2369 | 2298-2319 | 2148-2169 | ||||
| 11 | 2520-2542 | 2370-2392 | 2320-2342 | 2170-2192 | ||||
| 10½ | 2543-2556 | 2393-2406 | 2343-2356 | 2193-2206 | ||||
| 10 | 2557-2578 | 2407-2428 | 2357-2378 | 2207-2228 | ||||
| 9½ | 2579-2599 | 2429-2449 | 2379-2399 | 2229-2249 | ||||
| 9 | 2600-2620 | 2450-2470 | 2400-2420 | 2250-2270 | ||||
| 8½ | 2621-2642 | 2471-2492 | 2421-2442 | 2271-2292 | ||||
| 8 | 2643-2656 | 2493-2506 | 2443-2456 | 2293-2306 | ||||
| 7½ | 2657-2679 | 2507-2529 | 2457-2479 | 2307-2329 | ||||
| 7 | 2680-2701 | 2530-2551 | 2480-2501 | 2330-2351 | ||||
| 6½ | ≥2702 | ≥2552 | ≥2502 | ≥2352 | ||||
| 19 rounds - counts as 13 rounds | GM | IM | WGM | WIM |
| Different MO | 7 GM | 7 IM | 7 WGM | 7 WIM |
| *Min. other feds. | 2 | 2 | 2 | 2 |
| Rating floor for 1 player | 2200 | 2050 | 2000 | 1850 |
| Different TH | 10 | 10 | 10 | 10 |
| Max unrated | 4 | 4 | 4 | 4 |
| Max. from 1 fed. | 12 | 12 | 12 | 12 |
| Max. from own fed. | 11 | 11 | 11 | 11 |
| 15 | 2380-2397 | 2230-2247 | 2180-2197 | 2030-2047 |
| 14½ | 2398-2415 | 2248-2265 | 2198-2215 | 2048-2065 |
| 14 | 2416-2441 | 2266-2291 | 2216-2241 | 2066-2091 |
| 13½ | 2442-2466 | 2292-2316 | 2242-2266 | 2092-2116 |
| 13 | 2467-2482 | 2317-2332 | 2267-2282 | 2117-2132 |
| 12½ | 2483-2504 | 2333-2354 | 2283-2304 | 2133-2154 |
| 12 | 2505-2519 | 2355-2369 | 2305-2319 | 2155-2169 |
| 11½ | 2520-2542 | 2370-2392 | 2320-2342 | 2170-2192 |
| 11 | 2543-2563 | 2393-2413 | 2343-2363 | 2193-2213 |
| 10½ | 2564-2578 | 2414-2428 | 2364-2378 | 2214-2228 |
| 10 | 2579-2599 | 2429-2449 | 2379-2399 | 2229-2249 |
| 9½ | 2600-2620 | 2450-2470 | 2400-2420 | 2250-2270 |
| 9 | 2621-2635 | 2471-2485 | 2421-2435 | 2271-2285 |
| 8½ | 2636-2656 | 2486-2506 | 2436-2456 | 2286-2306 |
| 8 | 2657-2679 | 2507-2529 | 2457-2479 | 2307-2329 |
| 7½ | 2680-2694 | 2530-2544 | 2480-2494 | 2330-2344 |
| 7 | ≥2695 | ≥2545 | ≥2495 | ≥2345 |
-
- Title Tournament Certificates
The Chief Arbiter must prepare in quadruplicate certificates of title results achieved. These copies must be provided to the player, the player’s federation, the organizing federation and the FIDE Office. The player is recommended to ask the Chief Arbiter for the certificate before leaving the tournament.
The Chief Arbiter is responsible for that TRF file must be submitted to FIDE.
-
- Submission of Reports on Title Tournaments
Such tournaments must be registered as in 1.11.
-
- The end of a tournament is the date of the last round and the deadline for submitting the tournament shall be calculated from that date.
- The Chief Arbiter of a FIDE registered tournament has to provide the tournament report (TRF file) within 7 days after the end of the tournament to the Rating Officer of the federation where the tournament took place. The Rating Officer shall be responsible for uploading the TRF file to the FIDE Rating Server not later than 30 days after the end of the tournament.
- Reports shall include a database of at least those games played by players who achieved title results.
-
- Application Procedure for Players’ Titles
1.10a Registration of Direct Titles
The Chief Arbiter sends the results to the FIDE Office. The FIDE Office together with the QC Chairman creates a list of possible titles. The federations concerned are informed by the FIDE Office. If the federation agrees to apply for the title, then the title is confirmed.
1.10b Titles by application
The application must be sent and signed by the player’s federation. If the player’s federation refuses to apply, the player can appeal to FIDE and apply (and pay) for the title himself.
All the certificates have to be signed by the chief arbiter of the tournament and by the federation responsible for the tournament.
Application Forms for titles are annexed hereto. They are:
| Title | Norm Forms | Application Forms |
| Certificate of Title Result | IT1 | IT2 |
| Tournament Report Form | IT3 |
|
-
- Applications for these titles must be prepared on these forms and all the information required supplied together with the application:
GM; IM; WGM; WIM - IT2, IT1s, each with cross-tables
-
- Applications must be submitted to FIDE by the federation of the applicant. The national federation is responsible for the fee.
- There is a 30 day deadline in order for the applications to be considered properly. There is a 50% surcharge for applications to be considered in a shorter time-scale than this.
Exception: the surcharge may be waived, if the last norm was achieved so late that the time limit could not be observed.
Those arriving during the Presidential Board, Executive Board or General Assembly shall be charged a 100% supplement, with no exception.
-
- All applications together with full details must be posted on the FIDE website for a minimum of 60 days prior to finalisation. This is in order for any objections to be lodged.
- Certificate of title result IT1.
- Title Application form IT2.
- Tournament report form IT3.
Table for Direct Titles effective from 1 July 2017
DIRECT TITLES: Gold = first after tiebreak; 1st equal = best 3 players after tiebreak; norm = 9 games
Continental & Regional = Continental & maximum 3 regional events per continent, including Arab Youth
Sub-Continental Individual = include Zonals & Sub-zonals (if they establish qualifiers to World Cup or World Championship
– see 1.24), Arab adult Titles may be awarded conditional upon rating – see 0.62
Table 1.24a
| EVENT | WGM | WIM | WFM | WCM |
| Women’s World | Reaching last 8 - title | Qualifying through play - title |
|
|
| Olympiad |
|
|
65% in min 9 games - title | 50% in min 7 games - title |
| World Team (or Club) |
|
|
65% in min 7 games - title | 50% in min 7 games – title |
| World Amateur - <2300, <2000 |
|
|
Gold - title | Silver & Bronze – title |
| <1700 |
|
|
|
Gold - title |
| World >65; >50 Individual | Gold – title; | Silver & Bronze - title |
|
|
| World U20 | 1st equal – norm |
|
|
|
| World U18 | Gold – norm | 1st equal – title; Silver & Bronze – norm | Silver & Bronze - title |
|
| World U16 |
|
Gold – title; | Silver & Bronze - title |
|
| 1st equal – norm | ||||
| World U14 |
|
Gold – norm | 1st equal – title | Silver & Bronze – title |
| World U12 - World Schools U17; U15; U13 |
|
|
1st equal – title |
Silver & Bronze – title |
| World U10; U8 World Schools U11; U9; U7 |
|
|
|
Gold, Silver & Bronze - title |
| Continental Team (or Club) |
|
|
65% in min 7 games - title | 50% in min 7 games – title |
| Continental Individual | Gold – title; 1st equal - norm | Silver & Bronze - title |
|
|
| Continental >65; >50 Individual Continental & Regional U20 | Gold – norm | 1st equal – title; Silver & Bronze – norm | Silver & Bronze – title |
|
| Continental & Regional U18 |
|
Gold – title; 1st equal – norm | Silver & Bronze – title |
|
| Continental & Regional U16 |
|
Gold – norm | 1st equal – title | Silver & Bronze – title |
| Cont. & Regional U14; U12 Cont. Schools U17; U15; U13 |
|
|
Gold – title | Silver & Bronze – title |
| Continental Amateur Cont. & Regional U10; U8 Cont. Schools U11; U9; U7 |
|
|
|
Gold Silver & Bronze - title |
| Sub-Continental Individual |
|
1st equal – title; Silver & Bronze – norm | Silver & Bronze – title 65% in min 9 games - title | 50% in min 9 games - title |
| Commonwealth, Francophone, Iberoamerican Individual Disabled - World adult |
|
1st equal – title; Silver & Bronze – norm | Silver & Bronze - title |
|
| Disabled - U20 World |
|
|
1st equal – title | Silver & Bronze – title |
Table 1.24b
| EVENT | GM | IM | FM | CM |
| World Cup | Reaching last 16 - title | Qualification through play - title |
|
|
| Women’s World | Winner – title; Finalist - norm | Finalist - title |
|
|
| Olympiad |
|
|
65% in min 9 games - title | 50% in min 7 games - title |
| World Team (or Club) |
|
|
65% in min 7 games - title | 50% in min 7 games – title |
| World Amateur - <2300, <2000,<1700 |
|
|
Gold - title | Silver & Bronze – title Gold - title |
| World >65; >50 Individual World U20 | Gold – title; 1st equal – norm | Silver & Bronze - title |
|
|
| World U18 | Gold – norm | 1st equal – title; Silver & Bronze – norm | Silver & Bronze - title |
|
| World U16 |
|
Gold – title; 1st equal – norm | Silver & Bronze - title |
|
| World U14 |
|
Gold – norm | 1st equal – title | Silver & Bronze – title |
| World U12 World Schools U17; U15; U13 |
|
|
1st equal – title | Silver & Bronze – title |
| World U10; U8 World Schools U11; U9; U7 |
|
|
|
Gold, Silver & Bronze - title |
| Continental Team (or Club) |
|
|
65% in min 7 games - title | 50% in min 7 games – title |
| Continental Individual | Gold – title; 1st equal - norm | Silver & Bronze - title |
|
|
| Continental >65; >50 Individual Continental & Regional U20 | Gold – norm | 1st equal – title; Silver & Bronze – norm | Silver & Bronze – title |
|
| Continental & Regional U18 |
|
Gold – title; 1st equal – norm | Silver & Bronze – title |
|
| Continental & Regional U16 |
|
Gold – norm | 1st equal – title | Silver & Bronze – title |
| Cont. & Regional U14; U12 Cont. Schools U17; U15; U13 | Gold – title | Silver & Bronze – title | ||
| Continental Amateur Cont. & Regional U10; U8 Cont. Schools U11; U9; U7 | Gold Silver & Bronze - title | |||
| Sub-Continental Individual |
|
1st equal – title; Silver & Bronze – norm | Silver & Bronze – title 65% in min 9 games - title | 50% in min 9 games - title |
| Commonwealth, Francophone, Iberoamerican Individual Disabled - World adult | 1st equal – title; Silver & Bronze – norm | Silver & Bronze - title | ||
| Disabled - U20 World | 1st equal – title | Silver & Bronze – title |
Guideline for checking if a players’ result is a valid title norm:
In a 13 round Swiss tournament a player from Hungary played the following opponents with the relevant results and is looking for a GM norm:
| 1. GM Alfred | GER | 2383 | 1 |
| 2. GM Bernhard | ENG | 2508 | 0,5 |
| 3. Christian | GER | 2573 | 0 |
| 4. David | AUT | 2180 | 1 |
| 5. GM Evgeny | RUS | 2598 | 1 |
| 6. GM Friedrich | GER | 2568 | 0 |
| 7. Georg | GER | 2070 | 1 |
| 8. IM Herbert | GER | 2483 | 1 |
| 9. Igor | RUS | 2497 | 1 |
| 10. Konrad | GER | 2561 | 0,5 |
| 11. FM Ludwig | GER | 2440 | 1 |
| 12. IM Manfred | GER | 2479 | 0,5 |
| 13. GM Norbert | GER | 2492 | 0,5 |
Working from Table for 13 rounds:
-
- The average rating of his opponents is 2449. There are two low rated players, David in round 4 and Georg in round 7. According to article 1.46c for one player, the lowest rated one, the adjusted rating floor may be used for calculation. For a GM norm it is 2200. If we raise the rating of George from 2070 to 2200 it gives an average rating 2459.
- For GM norm, player needs 9 points (Average of opponents 2459-2489). It is OK.
- Check the titles of the opponents – see art. 1.45.
For a GM norm at least 1/3 with a minimum 3 of the opponents must be GMs. He should have 5 GMs. It is OK
At least 50% of the opponents shall be title‐holders; CM and WCM are not counted. He should have 7 Different Title holders. There are 5 GM, 2 IM and 1 FM. It is OK.
-
- Check the federations of the opponents – see art. 1.43 and 1.44.
For the GM norm at least two federations other than that of the title applicant must be included and there are four such federations, GER, ENG, AUT and RUS. It is OK.
A maximum of 3/5 of the opponents may come from the applicant’s federation and a maximum of 2/3 of the opponents from one federation.
9 opponents are coming from GER. Max. 2/3 may come from one federation. Therefore, the foreigner condition is not fulfilled. We proceed to the next step.
-
- Check if some exceptions are valid – see art. 1.42e and 1.43e.
1.43e : The foreigner rule is not valid for Swiss System tournaments in which at least 20 FIDE rated players, not from the host federation, are included, from at least 3 federations, at least 10 of whom hold GM, IM, WGM or WIM titles.
When applying for the title at least one of the norms has to be achieved under normal foreigner requirement.
1.42e: A player may ignore his game(s) against any opponents he has defeated, provided he has met the required mix of opponents, and provided that this leaves him with at least the minimum number of games as in 1.41, against the required mix of opponents.
If the win against Georg is deleted we remain with 8 points from 12 games, average rating is 2480. As for a 67% result dp = 125 we have a performance of 2605, sufficient for a GM norm.
Furthermore the player had 5 GMs and enough title holders. The title criteria are fulfilled.
Out of 12 opponents only 8 are coming from Germany, this criterion is fulfilled as well.
Now we have a valid GM title norm.
Some more examples of title norms:
- In an 11 round tournament a player finished with the following result
- 9 points
- average rating of opponents is 2376
- he played 4 GM and 2 FM
- his lowest rated opponents had 2140 (a win) and 2160 (a draw)
9 points, 4 GM and 6 title holders from 11 opponents are sufficient for a GM norm. For a GM norm the average rating of opponents is too low. It should be 2380 – see
1.49 Following the article 1.46.c the rating of one player may be raised to the adjusted rating floor for a GM norm, which is 2200. Using this adjustment, the average rating of opponents now is 2381 and the GM norm is valid.
Another possibility gives article 1.42e:
The player may ignore a game against any opponent he had defeated, provided he has met the required mix of opponents, and provided that this leaves him with at least the minimum number of games as in 1.41, against the required mix of opponents.
If we delete the game against the player with the rating of 2140 the player had 8 points from 10 games and an average rating of 2400. The GM norm is fulfilled.
Now we have to check the federations of the opponents – see article 1.43. Only 6 opponents may come from the players’ federation, only 7 opponents may come from one federation.
- In a women tournament, scheduled for 9 rounds, a player from Russia has after 8 rounds the following result
- 6 points
- average rating of opponents is 2165
- she played 2 WGM, 1 WIM and 2 WFM
- 6 of her opponents came from Germany and 2 from England
For a WGM norm she needs 7 points, and average rating of her opponents of 2180 or more and 3 WGMs. The number of title holders is already sufficient. Up to now she had 6 opponents from Germany, which is the maximum number coming from one federation.
In order to achieve this WGM norm for the last round she needs a third WGM having a rating of at least 2311, which is not from Germany, and she has to win.
- A player from Armenia, who has the IM title, participates in a 9 rounds Swiss System Tournament, has the following results against his 8 opponents and before the start of the last round asks the Arbiter what shall be his result of the last round so that he will achieve a norm for GM :
| 1. (NOR) |
|
2470 | 0 |
| 2. (GEO) | ‐ | 2150 | 1 |
| 3. (GER) | ‐ | 2410 | 1 |
| 4. (ARM) | IM | 2570 | 0 |
| 5. (GEO) | ‐ | 2340 | 1 |
| 6. (GEO) |
|
2380 | 1 |
| 7. (ARM) | GM | 2675 | 0,5 |
| 8. (ENG) | IM | 2540 | 1 |
| 9. (USA) |
|
2695 | ?? |
- We check the requirements for the GM title:
- Games: He will have played 9 games (9 are required). So it is o.k.
- Title holders (TH): He has 6 TH in 9 opponents. It is more than 50%. So it is o.k.
- Unrated opponents: None: 0 < 2. So it is o.k. (d). Federations:
- max 2/3 from one Fed.: 3 out of 9(GEO) : 3/9<2/3. Then it is o.k.
- max 3/5 from own Fed : 2 out of 9 (ARM)) :2/9<3/5. Then it is o . k .
(e). GMs : He met 3 GMs (3 are required). It is o.k.
- We calculate the Average Rating of the Opponents Ra:
(a). First we consider the Rating adjusted floor ( it is 2200 for GM norm ) for the opponent who is has lower rating than 2200 : 2. (GEO), ‐, 2150.
(b). We find : Ra=22280:9=2475,55‐‐‐‐‐‐2476
- Using the tables 1.49 for 9 games and GM norm :
For a Ra=2476 the player needs 6 points in 9 games for GM norm. Ha has 5.5 points in 8 games. So HE NEEDS A DRAW in the last round to get his GM norm.
FIDE RATING REGULATION
Effective from 1 July 2017
Approved by the 1982 General Assembly, amended by the General Assemblies of 1984 through 2016.
A game played over the board will be rated by FIDE when it takes place in a FIDE registered tournament and meets all the following requirements.
-
- The following regulations shall be altered by the General Assembly upon recommendation of the Qualification Commission (QC). Any such changes shall come into effect on 1st July of the year following the decision by the General Assembly. For tournaments, such changes will apply to those starting on or after that date.
- The tournaments to be rated shall be pre-registered by the federation that will be responsible for the submission of results and rating fees. The tournament and its playing schedule must be registered one week before the tournament starts. The QC Chairman may refuse to register a tournament. He may also allow a tournament to be rated even though it has been registered less than one week before the tournament starts. Tournaments where norms will be available must be registered 30 days in advance.
- All arbiters of a FIDE rated tournament shall be licensed otherwise the tournament shall not be rated.
- Tournament reports for all official FIDE and Continental events must be submitted and shall be rated. The Chief Arbiter is responsible for the results submitted.
- FIDE reserves the right not to rate a specific tournament. The organizer of the tournament has the right to appeal to the QC. Such an appeal must be made within seven days of communicating the decision.
- Rate of Play
-
- For a game to be rated each player must have the following minimum periods in which to complete all the moves, assuming the game lasts 60 moves. Where at least one of the players in the game has a rating 2200 or higher, each player must have a minimum of 120 minutes.
Where at least one of the players in the game has a rating 1600 or higher, each player must have a minimum of 90 minutes.
Where both of the players in the game are rated below 1600, each player must have a minimum of 60 minutes.
|
Suppose in a group of players below 2200 there is one player with a rating of 2210. Only the games of this higher rated player will not be rated. |
-
- Where a certain number of moves is specified in the first time control, it shall be 40 moves.
- For a game to be rated on the Rapid list, each player must have more than ten minutes but less than sixty minutes.
- For a game to be rated on the Blitz list, each player must have at least five but no more than ten minutes.
2.1. Play must take place according to the FIDE Laws of Chess.
3.1. There must be no more than 12 hours play in one day. This is calculated based on games that last 60 moves, although games played using increments may last longer.
- Duration of the Tournament:
- For tournaments, a period not greater than 90 days, except:
- Leagues may be rated which last for a period greater than 90 days.
- The QC may approve the rating of tournaments lasting more than 90 days.
- For tournaments lasting more than 90 days, interim results must be reported on a monthly basis.
- For tournaments, a period not greater than 90 days, except:
5.1. Whether these occur because of forfeiture or any other reason, they are not counted. Any game where both players have made at least one move will be rated.
- Composition of the Tournament
- If an unrated player scores zero in his first tournament, his score and that of his opponents against him are disregarded. Otherwise if an unrated player has played rated games, then this result is included in computing his overall rating.
- In a round-robin tournament at least one-third of the players must be rated. Subject to this requirement,
- If the tournament has less than 10 players, at least 4 must be rated.
- In a double round-robin tournament with unrated participants, there must be at least 6 players, 4 of whom must be rated.
-
-
- National Championships played as round-robin shall be rated if at least 3 players (or 2 women in events exclusively for women) had official FIDE Ratings before the start of the tournament.
- In a Swiss or Team Tournament:
- For an unrated player’s first performance to count, he must score at least 1/2 point.
- For rated players, only games against rated opponents are counted.
- In the case of a round-robin tournament where one or more games are unplayed, the results of the tournament must be reported for rating as if for a Swiss system tournament.
- Where a match is over a specific number of games, those played after one player has won shall not be rated.
- Matches in which one or both of the players are unrated shall not be rated.
-
- Official FIDE Rating List
- On the first day of each month, the QC shall prepare a list which incorporates the rated play during the rating period into the previous list. This shall be done using the rating system formula.
- The rating period (for new players, see 7.14) is the period where a certain rating list is valid.
- The following data will be kept concerning each player whose rating is at least 1000 as of the current list:
- On the first day of each month, the QC shall prepare a list which incorporates the rated play during the rating period into the previous list. This shall be done using the rating system formula.
FIDE title, Federation, Current Rating, ID Number, Number of games rated in the rating period, Date of Birth, Gender and the current value of K for the player.
-
-
- The closing date for tournaments for a list is 3 days before the date of the list; tournaments ending before or on that day may be rated on the list. Official FIDE events may be rated on the list even if they end on the last day before the list date.
- A rating for a player new to the list shall be published only if it meets the following criteria:
-
7.14a If based on results obtained under 6.3, a minimum of 5 games. 7.14b If based on results obtained under 6.4, a minimum of 5 games played against rated opponents.
7.14c The condition of a minimum of 5 games need not be met in one tournament. Results from other tournaments played within consecutive rating periods of not more than 26 months are pooled to obtain the initial rating.
7.14d The rating is at least 1000.
7.14e The rating is calculated using all his results as if they were played in one tournament (it is not published until he has played at least 5 games) by using all the rating data available.
-
- Players who are not to be included on the list:
-
-
- Players whose ratings drop below 1000 are listed on the next list as 'delisted'. Thereafter they are treated in the same manner as any other unrated player.
- Titled players who are unrated are published in a separate list concurrently with the list of rated players.
- Inactive players are considered rated at their most recent published rating for purposes of rating and title results.
-
7.23a A player is considered to commence inactivity if he plays no rated games in a one year period.
7.23b A player regains his activity if he plays at least one rated game in a period and he is then listed on the next list.
The FIDE Rating system is a numerical system in which fractional scores are converted to rating differences and vice versa. Its function is to produce scientific measurement information of the best statistical quality.
-
- The rating scale is an arbitrary one with a class interval set at 200 points. The tables that follow show the conversion of fractional score 'p' into rating difference 'dp'. For a zero or 1.0 score dp is necessarily indeterminate but is shown notionally as 800. The second table shows conversion of difference in rating 'D' into scoring probability 'PD' for the higher 'H' and the lower 'L' rated player respectively. Thus the two tables are effectively mirror-images.
| p - dp | p - dp | p - dp | p - dp | p - dp | p - dp |
| 1.0 - 800 | .83 - 273 | .66 - 117 | .49 - 7 | .32 -133 | .15 - 296 |
| .99 - 677 | .82 - 262 | .65 - 110 | .48 - 14 | .31 - 141 | .14 - 309 |
| .98 - 589 | .81 - 251 | .64 - 102 | .47 - 21 | 30 - 149 | .13 - 322 |
| .97 - 538 | .80 - 240 | .63 - 95 | .46 - 29 | .29 - 158 | .12 - 336 |
| .96 - 501 | .79 - 230 | .62 - 87 | .45 - 36 | .28 - 166 | .11 - 351 |
| .95 - 470 | .78 - 220 | .61 - 80 | .44 - 43 | .27 - 175 | .10 - 366 |
| .94 - 444 | .77 - 211 | .60 - 72 | .43 - 50 | .26 - 184 | .09 - 383 |
| .93 - 422 | .76 - 202 | .59 - 65 | .42 - 57 | .25 - 193 | .08 - 401 |
| .92 - 401 | .75 - 193 | .58 - 57 | .41 - 65 | .24 - 202 | .07 - 422 |
| .91 - 383 | .74 - 184 | .57 - 50 | .40 - 72 | .23 - 211 | .06 - 444 |
| .90 - 366 | .73 - 175 | .56 - 43 | .39 - 80 | .22 - 220 | .05 - 470 |
| .89 - 351 | .72 - 166 | .55 - 36 | .38 - 87 | .21 - 230 | .04 - 501 |
| .88 - 336 | .71 - 158 | .54 - 29 | .37 - 95 | .20 - 240 | .03 - 538 |
| .87 - 322 | .70 - 149 | .53 - 21 | .36 - 102 | .19 - 251 | .02 - 589 |
| .86 - 309 | .69 - 141 | .52 - 14 | .35 - 110 | .18 - 262 | .01 - 677 |
| .85 - 296 | .68 - 133 | .51 - 7 | .34 - 117 | .17 - 273 | .00 - 800 |
| .84 - 284 | .67 - 125 | .50 - 0 | .33 - 125 | .16 - 284 |
8.1b Table of conversion of difference in rating, D, into scoring probability PD, for the higher, H, and the lower, L, rated player respectively.
0-3 .50 .50 92-98 .63 .37 198-206 .76 .24 345-357 .89 .11
4-10 .51 .49 99-106 .64 .36 207-215 .77 .23 358-374 .90 .10
11-17 .52 .48 107-113 .65 .35 216-225 .78 .22 375-391 .91 .09
18-25 .53 .47 114-121 .66 .34 226-235 .79 .21 392-411 .92 .08
26-32 .54 .46 122-129 .67 .33 236-245 .80 .20 412-432 .93 .07
33-39 .55 .45 130-137 .68 .32 246-256 .81 .19 433-456 .94 .06
40-46 .56 .44 138-145 .69 .31 257-267 .82 .18 457-484 .95 .05
47-53 .57 .43 146-153 .70 .30 268-278 .83 .17 485-517 .96 .04
54-61 .58 .42 154-162 .71 .29 279-290 .84 .16 518-559 .97 .03
62-68 .59 .41 163-170 .72 .28 291-302 .85 .15 560-619 .98 .02
69-76 .60 .40 171-179 .73 .27 303-315 .86 .14 620-735 .99 .01
77-83 .61 .39 180-188 .74 .26 316-328 .87 .13 > 735 1.0 .00
 84-91 .62 .38 189-197 .75 .25 329-344 .88 .12
84-91 .62 .38 189-197 .75 .25 329-344 .88 .12
-
- Determining the Rating 'Ru' in a given event of a previously unrated player.
- If an unrated player scores zero in his first event his score is disregarded. First determine the average rating of his competition 'Rc'.
- Determining the Rating 'Ru' in a given event of a previously unrated player.
- In a Swiss or Team tournament: this is simply the average rating of his opponents.
- The results of both rated and unrated players in a round-robin tournament are taken into account. For unrated players, the average rating of the competition 'Rc' is also the tournament average 'Ra' determined as follows:
- Determine the average rating of the rated players 'Rar'.
- Determine p for each of the rated players against all their opponents. Then determine dp for each of these players.
Then determine the average of these dp = 'dpa'.
-
- 'n' is the number of opponents. Ra = Rar - dpa x n/(n+1)
- If he scores 50%, then Ru = Ra
- 8.23 If he scores more than 50%, then Ru = Ra + 20 for each half point scored over 50%
- 8.24 If he scores less than 50% in a Swiss or team tournament: Ru = Ra
- 'n' is the number of opponents. Ra = Rar - dpa x n/(n+1)
+ dp
-
-
- If he scores less than 50% in a round-robin: Ru = Ra + dp x n/(n+1).
-
-
- The Rating Rn which is to be published for a previously unrated player is then determined as if the new player had played all his games so far in one tournament. The initial rating is calculated using the total score against all opponents. It is rounded to the nearest whole number.
- If an unrated player receives a published rating before a particular tournament in which he has played is rated, then he is rated as a rated player with his current rating, but in the rating of his opponents he is counted as an unrated player.
- Determining the rating change for a rated player
- For each game played against a rated player, determine the difference in rating between the player and his opponent, D.
- If the opponent is unrated, then the rating is determined at the end of the event. This applies only to round-robin tournaments. In other tournaments games against unrated opponents are not rated.
- The provisional ratings of unrated players obtained from earlier tournaments are ignored.
- A difference in rating of more than 400 points shall be counted for rating purposes as though it were a difference of 400 points.
- (a) Use table 8.1(b) to determine the player’s score probability PD
- ΔR = score – PD. For each game, the score is 1, 0.5 or 0.
- ΣΔR x K = the Rating Change for a given tournament, or Rating period.
-
- K is the development coefficient.
-
K = 40 for a player new to the rating list until he has completed events with at least 30 games.
K = 20 as long as a player's rating remains under 2400.
K = 10 once a player's published rating has reached 2400 and remains at that level subsequently, even if the rating drops below 2400.
K = 40 for all players until their 18th birthday, as long as their rating remains under 2300.
If the number of games (n) for a player on any list for a rating period multiplied by K (as defined above) exceeds 700, then K shall be the largest whole number such that K x n does not exceed 700.
-
-
- The Rating Change is rounded to the nearest whole number. 0.5 is rounded up (whether the change is positive or negative).
- Determining the Ratings in a round-robin tournament.
-
Where unrated players take part, their ratings are determined by a process of iteration. These new ratings are then used to determine the rating change for the rated players.
Then the ΔR for each of the rated players for each game is determined using Ru(new) as if an established rating.
- Reporting Procedures
- The Chief Arbiter of a FIDE registered tournament has to provide the tournament report (TRF file) within 7 days after the end of the tournament to the Rating Officer of the federation where the tournament took place. The Rating Officer shall be responsible for uploading the TRF file to the FIDE Rating Server not later than 30 days after the end of the tournament.
- Results of all international competitions must be submitted for rating unless the original invitations have made it clear the event was not to be FIDE rated. The chief arbiter must also announce this to the players before the tournament starts.
- Each national federation shall designate an official to coordinate and expedite qualification and rating matters. His name and details must be given to the FIDE Secretariat.
- Monitoring the Operation of the Rating System
- One of the functions of Congress is to establish the policies under which FIDE titles and ratings are awarded. The function of the rating system is to produce scientific measurement information of the best statistical quality to enable Congress to award equal titles for equal proficiencies of players. Thus the rating system must be properly scientifically maintained and adjusted on both a short and long term basis.
- The rating scale is arbitrary and open ended. Thus only differences in ratings have any statistical significance in terms of probability. Thus if the composition of the FIDE Rating pool were to change, the rating scale could drift with respect to the true proficiency of the players. It is a major objective to ensure the integrity of the system so that ratings of the same value from year to year represent the same proficiency of play.
- Part of the responsibilities of the Rating System Administrator is to detect any drift in the rating scale.
- The requirements for the FIDE Rating System Administrator
- A sufficient knowledge of statistical probability theory as it applies to measurements in the physical and behavioural sciences.
- Ability to design the surveys described under 12.3; to interpret the results of the surveys; and to recommend the Qualification Commission whatever measures are needed to preserve the integrity of the rating system.
- To be able to advise and assist any FIDE member federation in the establishment of a national rating system.
- To display a level of objectivity comparable to that of a FIDE Arbiter.
- Some comments on the Rating system
- The following formula gives a close approximation to tables 8.1a and 8.1b.
P = 1/(1 + 10 -D/400). However the tables are used as shown.
-
- Tables 8.1a and 8.1b are used precisely as shown, no extrapolations are made to establish a third significant figure.
- K is used as a stabilising influence in the system. When K = 10, the rating turns over in approximately 70 games; K = 20, it is 35 games; K = 40, it is 18 games.
- The system has been devised to enable players to verify their ratings readily.
- Inclusion in the Rating list
- To be included in the FRL or FIDE Rapid/Blitz Rating Lists, a player must be registered through a national chess federation which is a member of FIDE. The Federation must not be temporarily or permanently excluded from membership.
- It is the responsibility of national Federations to inform FIDE if players should not be included in the FRL.
- Any player excluded from the rating list because he is unable to obtain membership of a national federation, may apply to FIDE for special dispensation to be included in the list.
Example for the ratings calculations
In a 9 round Swiss System Tournament a player with a FIDE Rating 2212 and less than 30 games played in his chess career, played against the following opponents with the relevant ratings and had the following results :
1. (1926) 1
2. (2011) 1
3. (2318) 0
4. (2067) 0.5
5. (2219) 0.5
6. (2585) 0
7. (2659) 1
8. (2464) 0.5
9. (2652) 0.5
Calculate his new rating after the end of the tournament.
We calculate the rating difference for every opponent, using the table 8.1 (b): 1. 2212‐1926=286, result 1, p(H)=0.84, dR=1‐0.84 = +0.16
2. 2212‐2011=201, result 1, p(H)=0.76, dR=1‐0.76 = +0.24
3. 2318‐2212=106, result 0, p(L)= 0.36, dR=0‐0.36 = ‐0.36
4. 2212‐2067=145, result 0.5, p(H)=0.69, dR=0.5‐0.69 = ‐0.19
5. 2219‐2212=7, result 0.5, p(L)= 0.49, dR=0.5‐0.49 = +0.01
6. 2585‐2212=373, result 0, p(L)=0.10, dR=0‐0.10 = ‐0.10
7. 2659‐2212=447 we consider max. 400, result 1, p(L)=0.08, dR =1‐0.08= +0.92
8. 2464‐2212=252, result 0.5, p(L)=0.19, dR=0.5‐0.19= +0.31
9. 2652‐2212=440, we consider max. 400, result 0.5, p(L)=0.08, dR=0.5‐0.08=
+0.42
ΣdR= 0.16+0.24‐0.36‐0.19+0.01‐0.10+0.92+0.31+0.42 = +1.41
So his Rating Change will be: K X ΣdR=40 X 1.41 = +56.4 His New Rating will be 2212 + 56.4 = 2268.4 ‐‐‐‐‐‐‐ 2268
REGULATIONS FOR THE TITLES OF ARBITERS
As approved by GA 1982, amended by GA 1984 to 2016.
- Introduction
- The following regulations can only be altered by the General Assembly following recommendation by the Arbiters’ Commission.
- Changes to the regulations shall only be made every four year, commencing from 2004 (unless the Commission agrees urgent action is required).
- Any such changes shall take effect from 1 July of the year following the decision by the General Assembly.
- The titles for award are International Arbiter (IA) and FIDE Arbiter (FA).
- The titles are valid for life from the date awarded or registered.
- The judging unit is The FIDE Arbiters’ Commission.
- The Arbiter Commission is appointed by the General Assembly for the same period of office as the FIDE President. The Commission shall include a Chairman, appointed by the FIDE President, a Secretary, appointed by the Chairman in consultation with the FIDE President and not more than 11 experts, maximum one per federation, who shall have voting rights in the Commission. No federation shall have more than one representative in the Commission.
- The Presidential Board or Executive Board may confirm the titles under
- The following regulations can only be altered by the General Assembly following recommendation by the Arbiters’ Commission.
1.1.3 in clear cases only, after consultation with the Arbiters Commission chairman.
-
-
- The Commission usually makes its decisions in the sessions immediately preceding the opening of the General Assemblies.
- In exceptional circumstances, the Commission may recommend a title by correspondence voting.
- National federations may register their Arbiters of National level(s) with FIDE after approval by the FIDE Arbiters’ Commission.
-
Level: World, Continental, National championships Type: Individual or Team
Certificates: number of norm certificates to be issued Norms: number of norms that can be used in application
| Format | Level of Event | Type | Certificates | Norms |
| All kind | World | any kind | no limit | no limit |
| All kind | Continental | any kind | no limit | no limit |
| Swiss | International | any kind | 1 per 50 players | no limit |
| Round Robin | International | any kind | maximum 2 | no limit |
| Swiss | National Championships | Individual / Team (adult) | 1 per 50 players | maximum 2 |
| Round Robin | National Championship | Individual / Team (adult) | maximum 2 | maximum 2 |
| Rapid Chess | World / Continental | any kind | no limit | maximum 1 |
-
-
- An arbiter in the highest division of the National Team Championship; whereby the following requirements are met:
- a minimum of four boards per team;
- a minimum of ten teams and six teams, in case of a Double Round Robin tournament;
- at least 60% of the players are FIDE rated;
- at least five rounds.
- Two (2) different formats of tournaments shall be included as norms for the applications for both FA and IA title (i.e. Swiss or Round Robin or Team Tournaments). Only Swiss System Tournaments may be accepted in case that at least one (1) of them is an international FIDE rated chess event with at least 100 players, at least 30% FIDE rated players, and at least 7 rounds.
- An arbiter in the highest division of the National Team Championship; whereby the following requirements are met:
-
|
In order an International FIDE rated chess event to be valid as a norm for the FA title, it must have participants from at least two Federations. |
-
-
- Applicants for the title of IA/FA must be at least 21 years old.
- Arbiters of national Level must be at least 16 years old.
-
All of the following:
-
- Thorough knowledge of the Laws of Chess, the FIDE Regulations for chess competitions and the Swiss Pairing Systems.
- Absolute objectivity, demonstrated at all times during his activity as an arbiter.
- Sufficient knowledge of the at least one official FIDE language.
- Skills to operate electronic clocks of different types and for different systems.
- Experience as Arbiter in at least three (3) FIDE rated events (these can be either national or international) and attendance of at least one (1) FIDE Arbiters’
Seminar and successful passing (at least 80%) an examination test set up by the Arbiters Commission.
FIDE rated event valid for a norm is considered any tournament with minimum 10 players in case that it is played with Round Robin system, with minimum 6 players in case that it is played with Double Round Robin system and with minimum 20 players in case that it is played with Swiss system.
-
- The title of the FIDE Arbiter for each of the IBCA, ICCD, IPCA shall each be equivalent to one FA norm.
- For a candidate, being a match arbiter in an Olympiad is equivalent to one FA norm. No more than one such norm will be considered for the title.
- Being Arbiter in any FIDE rated Rapid or Blitz events, with minimum thirty
(30) players and nine (9) rounds, shall be equivalent to one (1) FA norm. No more than one such norm from Rapid or Blitz tournaments will be considered for the title.
-
- Attendance of one (1) FIDE Arbiters Seminar and successful passing (at least 80%) an examination test set by the Arbiters Commission, shall be equivalent to one (1) FA norm. Not more than one (1) such norm will be considered for the title.
- Applicants from federations which are unable to organize any tournaments valid for titles or rating, may be awarded the title on passing an examination set by the Arbiters’ Commission.
All of the following:
-
- Thorough knowledge of the Laws of Chess, the FIDE Regulations for chess competitions, the Swiss Pairing Systems, the FIDE Regulations regarding achievement of title norms and the FIDE Rating System.
- Absolute objectivity, demonstrated at all times during his activity as an arbiter.
- Obligatory knowledge of English language, minimum at conversation level; and of chess terms in other official FIDE languages.
- Minimum skills at user level to work on a personal computer. Knowledge of pairing programs endorsed by the FIDE, Word, Excel and email.
- Skills to operate electronic clocks of different types and for different systems.
- Experience as Arbiter in at least four (4) FIDE rated events such as the following:
- The final of the National Individual (adult) Championship (maximum two norms).
- All official FIDE tournaments and matches.
- International tournaments where FIDE title norms for players are possible.
- International FIDE rated chess events with at least 100 players, at least 30% FIDE rated players, and at least seven rounds (maximum one norm).
- All official World and Continental Rapid and Blitz Championships for adult and juniors (maximum one (1) norm).
- The title of the International Arbiter for each of the IBCA, ICCD, IPCA shall each be equivalent to one IA norm.
-
- Being a match arbiter in an Olympiad is equivalent to one IA norm. No more than one such norm will be considered for the title.
- The title of International Arbiter can be awarded only to applicants who have already been awarded the title of FIDE Arbiter.
- All the norms for the IA title must be different from the norms already used for the FA title and must have been achieved after the FA title has been awarded.
- At least two (2) of the submitted norms shall be signed by different Chief Arbiters.
- Application Procedure
- Application forms for the titles listed in 1.1.3 are annexed hereto. They are: Tournament Report Form with cross-table and decisions on appeals – IT3 (one for each norm)
Arbiter Norm Report Form – IA1 or FA1 (one for each norm) Arbiter Title Application Form – IA2 or FA2.
-
- For the FIDE Arbiter title the norms must include tournaments (according to 3.5) with at least seven (7) rounds. Only one (1) tournament with five (5) or six
(6) rounds shall be accepted.
For the International Arbiter title the norms must include tournaments (according to 4.6) with at least nine (9) rounds. Only one (1) tournament with seven (7) or eight (8) rounds shall be accepted.
In case of norms from Team Tournaments the number of rounds where the applicant was an Arbiter must be at least five (5) and it must be indicated in the FA1/IA1 form.
All the certificates have to be signed by the Chief Arbiter and the federation responsible for the tournament.
In case the applicant is the Chief Arbiter of the event, then the Organizer or the Federation Official may sign the certificate.
If the Chief Arbiter is Arbiter of National Level, he cannot sign any certificate for International Arbiter title.
-
- All norms included in the applications must have been achieved in events with starting dates that fall within a six-year period. The application must be submitted not later than the second FIDE Congress after the date of the latest event listed. Norms from Seminars are valid for a period of four (4) years.
- Applications must be submitted to the FIDE Secretariat by the federation of the applicant. The National federation is responsible for the fee. If the applicant’s federation refuses to apply, the applicant can bring his case to the Arbiters’ Commission, who will investigate it. If it is found that there is no sufficient reason for the refusal, the applicant can appeal to FIDE and apply (and pay) for the title himself.
- There is a 60-day deadline in order for the applications to be considered properly. There is a 50% surcharge for applications to be considered in a shorter time-scale than this. Those arriving during the Presidential Board, Executive Board or General Assembly shall be charged a 100% supplement.
Exception: the surcharge may be waived, if the last norm was achieved so late that the time limit could not be observed.
-
- All applications together with full details must be posted on the FIDE website for a minimum of 60 days prior to finalization. This is in order for any objections to be lodged.
- Arbiters’ Licence.
- A titled active Arbiter (International Arbiter or FIDE Arbiter) and Arbiters of National levels working in a FIDE rated tournament shall be charged with a “license fee”.
- The license will be valid for life, on the condition the Arbiter remains an active arbiter, and will be in effect from the day after FIDE has received the fee.
- The license fee for Arbiters of National levels is valid for life.
- If an Arbiter of National level is awarded the title of “FIDE Arbiter” the license fee for this title has to be paid to FIDE.
- If an Arbiter upgrades his/her category only the difference between the category fees has to be paid to FIDE.
- If a “FIDE Arbiter” achieves the title of “International Arbiter”, the fee for the new title has to be paid to FIDE.
- A titled active Arbiter (International Arbiter or FIDE Arbiter) and Arbiters of National levels working in a FIDE rated tournament shall be charged with a “license fee”.
-
- The license fee will be:
- for A’ Category Arbiters (only IAs): 300 €
- for B’ Category Arbiters (only IAs): 200 €
- for C’ Category Arbiters: IAs 160 €
FAs 120 €
- for D’ Category Arbiters: IAs 100 €
FAs 80 €
- for Arbiters of National Levels (NA): 20 €
- Failure to pay the license fee will lead to exclusion from the FIDE Arbiters’ list.
- The Arbiters’ license will come into effect from 01. 01. 2013.
- From 01. 01. 2013 all arbiters of FIDE rated tournaments shall be licensed.
- An arbiter who has become inactive (see annex 2, articles 1.3 and 1.4) is considered not to be licensed any more.
- In order to be active again the arbiter has to pay for a new license, according to 6.3.
-
- If the article 6.6 is not fulfilled, the tournaments shall not be rated and any Arbiters’ norms shall not be accepted.
- From 01. 01. 2013 the license fee will be charged together with the application fee for all awarded arbiter titles.
ANTI-CHEATING GUIDELINES
(These guidelines shall be included in the subjects and will be taught in all Workshops, FIDE Arbiters’ Seminars and Courses for International and FIDE Arbiters).
The FIDE Laws of Chess have introduced provisions against cheating. Specifically:
-
- The arbiter shall:
- ensure fair play.
- The arbiter shall:
12.2.7 follow the Anti-Cheating Rules or Guidelines
It means that it is the Arbiter’s duty to avoid the cheating by the players. The Laws also explicitly forbid electronic devices:
11.3.1 During play the players are forbidden to use any notes, sources of information or advice, or analyse any game on another chessboard.
-
-
-
- During a game, a player is forbidden to have any electronic device not specifically approved by the arbiter in the playing venue.
-
-
However, the regulations of an event may allow such devices to be stored in a player’s bag, provided the device is completely switched off. This bag must be placed as agreed with the arbiter. Both players are forbidden to use this bag without permission of the arbiter.
-
-
-
- If it is evident that a player has such a device on their person in the playing venue, the player shall lose the game. The opponent shall win. The regulations of an event may specify a different, less severe, penalty.
- The arbiter may require the player to allow his clothes, bags, other items or body to be inspected, in private. The arbiter or person authorised by the arbiter shall inspect the player, and shall be of the same gender as the player. If a player refuses to cooperate with these obligations, the arbiter shall take measures in accordance with Article 12.9.
-
-
Tournament organizers are also free to introduce their own regulations and conditions for events, provided they are in accord with the Laws of Chess.
Recommendations for Arbiters
The adoption of ACC regulations will require a substantial effort to FIDE and its arbiters. In par- ticular, the role of arbiters in chess will need to be rethought, and the resulting shall be the product of close interaction between the ACC and the Arbiters Commission.
-
-
-
- Continuous Training
-
-
The ACC feels that Continuous Training is desirable for all FIDE-Titled arbiters. In the medium term, the ACC, together with the Arbiters Commission, should organize special Anti-Cheating
Training Sessions aimed at FIDE Arbiters The ACC recommends that the Arbiters Commission only raise the classification of Arbiters that have completed Anti-Cheating Training, as part of regular Arbiter Training or through special courses Such training is vital for successful application of AC regulations.
-
-
-
- Initial Recommendations
-
-
While waiting for the new training system to be developed, the ACC wishes to issue the following initial recommendations for arbiters It should be understood that if an arbiter feels a need for support from the ACC, he/she is strongly encouraged to contact the Commission to obtain con- sultancy about a particular situation
- How breaches of AC regulations may occur during the game:
- An arbiter should know how to recognize behaviors and devices involved in cases in the past. The definition of cheating according to Article 11. 3. a of the Laws of Chess includes
-
- accepting information by another person (spectator, captain, co-player, etc.); and ii) getting information from any source of information or communication (such as books, notes, etc., or any electronic device) It the arbiter’s duty to take care of situations that may yield suspicions of cheating during the entire duration of the round.
- The use of a mobile phone hidden in a pocket is forbidden according to Article 11. 3. b of the Laws of Chess To find hidden mobile phones and other electronic devices the use of hand-held metal detectors and other equipment (see Annex D) is highly recommended in all tournaments Arbiters should exercise caution and delicateness in asking for and carrying out a check with hand-held metal detectors. If a metal detector gives a signal it is important to clarify the reason, if necessary by an inspection of the player and his/her belongings as described in Article 11. 3. b of the Laws of Chess.
2 . Applicable precautions:
- The arbiter must have a discreet control of the players that are leaving the playing area very often, for their contact with other players, spectators and other persons, according to Article
12 of the Laws of Chess.
- The arbiter should be aware that in some cases a player can get information from a third party. The arbiter should prevent any contact between players and spectators such as talking and/or giving/receiving signals
- The arbiter should never tolerate the use of chess programs in the playing venue Finding that a player or spectator is using a chess program in the playing area calls for immediate action in conjunction with the Chief Arbiter.
- Organizers are free to assign extra arbiters to the specific task of preventing cheating
- During a tournament, the arbiter is encouraged to use the FIDE screening tool with PGN games, since that tool can identify cases needing further attention or, more likely, show that a player is not to be considered suspicious based on his or her games
- . Screening games for precaution and information:
- During a tournament, the arbiter is encouraged to compile games in PGN format and submit
them to the FIDE screening tool. As emphasized above, the screening test is not a cheating test and gives no statistical judgment, but its information is useful to have beforehand in case any suspicions are voiced or situations may be developing
- In early rounds (such as 1-3 of a 9-game event) there will always be outliers because the total number of relevant moves is small, but any cheating player will likely be among them.
-
- However, in later rounds, a persistent outlier may be grounds for contacting ACC, calling for a full statistical test, and for “unobtrusive” actions such as increased watchfulness of the player See Appendix C for more on screening and full-test procedures and interpretation
4 . How to deal with suspicious behavior:
- In case of a suspicious behavior, the Arbiter must always follow the player on his or her way out of the playing venue (to the bar, toilets, smoking area etc), in order to avoid any contact of the player with other persons and any use of external sources of information or communication
- In multiple cases, there has been use of mobile phones in the toilet. Therefore, the arbiter should note how often a player leaves the playing area and if this is significant take appro- priate measures trying to find out the reason. In addition, the arbiter should consider im- plementing procedures described next in sub-head 5
- How to deal with the new Article 11. 3 of the Laws of Chess:
- The arbiter may require the player to allow his/her clothes, bags, or other items to be in- spected, in private. The arbiter, or a person authorized by the arbiter, shall inspect the player and shall be of the same gender as the player.
- Usually the arbiter will inspect a player as described in Article 11. 3. b of the Laws of Chess only in case of suspected breach of AC regulations or after receiving an official In-Tourna- ment Complaint (see Section 3), but only after coming to the conclusion that the complaint is not evidently unfounded. If the arbiter decides to make an inspection on whatever grounds, it is not necessary to give the player a special reason. The arbiter, and any other person conducting the inspection, should be calm, polite and discreet The inspection of a player should be carried out in a separate room, again by a person of the same gender. Only this person, the player, and one witness (also of the same gender) may have access to this room during the inspection. The player is entitled to select a second witness of his own choice (of either gender).If there is no matter of urgency, the inspection of a player and his/her belongings should generally be carried out before or immediately after the end of the game Still, the arbiter should be aware that it is possible to hide electronic devices somewhere in or near to the playing venue, or to give them to a third party shortly before the end of the game The arbiter also has the right to check a player who has left the playing venue during a game, or upon request of a player who filed an In-Tournament Complaint, but only once during the round
- If a player refuses to be inspected, it is advised that the arbiter shall explain the rules calmly. If the player still refuses, he/she shall get a warning If the player still refuses to submit to an inspection, he/she shall lose the game and be precluded from further play in the tournament
- If random inspections are considered, they must be announced in the rules of the competition in advance
- . How to deal with accusations:
-
- The procedure for handling accusations is described under Section 3, Part A. If any FIDE- identified person presents an accusation of breach of AC regulations, the arbiter should ask him/her to make an official In-Tournament Complaint In case of refusal, the arbiter shall make a remark in the tournament report and annotate the person’s name as having presented an accusation of breach of AC regulations. In this case the accused player shall not be informed by the arbiter An arbiter who receives an In-Tournament Complaint may inform the accused player after the end of his/her game, and ask him/her for comment.
- The arbiter should describe in the tournament report any In-Tournament Complaints and inspections, stating the result of each action.
7 . How to deal with false accusations:
- In case of a false accusation by a player, the Arbiter shall penalize him/her according to Article 12. 2 of the Laws of Chess. For further procedures, see Section 3, Part A
THE ROLE OF THE ARBITERS AND THEIR DUTIES
The Arbiters are the connecting link between the organizer and the players of a tournament.
They have not only to control the games, but also to ensure the best conditions, for the players not to be disturbed and will be able to play without any problem. So they have to take care about the playing area, the equipment, the environment and the whole playing venue.
Finally they have to avoid any cheating by the players.
The general duties of the Arbiters in a competition are described in the Laws of Chess (art. 12) and are:
- They shall see that the Laws of Chess are observed.
- They shall ensure fair play and they have to follow the Anti cheating regulations. It means that they also must take care so that avoid any cheating by the players.
- They shall act in the best interest of the competition. They should ensure that a good playing environment is maintained and that the players are not disturbed. They shall supervise the progress of the competition
- They shall observe the games, especially when the players are short of time, enforce decisions they have made and impose penalties on players where appropriate.
In order to do all these, the Arbiters shall have the necessary competence, sound judgment and absolute objectivity (Preface of the Laws of Chess).
The number of the required Arbiters in a competition varies, depending on the kind of event
(Individual, Team), on the system of the games (Round Robin, Swiss System, Knock Out, Matches), on the number of participants and on the importance of the event.
Normally one Chief Arbiter, one Deputy Chief Arbiter and a number of Arbiters (approximately one for every 20 to 25 players) are appointed for a competition. In special cases (i.e. tiebreak games with adequate supervision), Assistant Arbiters may be appointed.
Additionally we can consider the following requirements as very important for the Arbiters in a competition:
-
- To show proper behavior to the players, captains and spectators and to be respectful and dignified.
They shall avoid any dispute during the games and take care of the good image of the tournament.
-
- To observe of as many games as possible during every round of the competition. They have to take care of the games that they are responsible, to observe and
to check the games’ progress (especially when there is time trouble).
It is not acceptable for the Arbiters to leave the playing area every 10 or 15 minutes for smoking or for any discussions with friends, spectators, officials, or other persons, or to leave their sector unattended in order to go and watch other games in another part of the playing hall. It is not acceptable for the Arbiters to stay seated in their chairs reading newspapers or books (even chess books!), or to sit in front of a computer, surfing on Internet, etc., leaving their games without observation. It is also not acceptable for the Arbiters to speak on their mobiles in the playing hall during the games. The Law of Chess regarding the mobile phones is valid not only for the players, captains and spectators, but for the Arbiters as well.
It is sure that the biggest problems during the games are caused because of the absence or the lack of attention of the Arbiters and thus the ignorance of what actually happened in case of an incident. How an absent Arbiter will take a fair decision in a dispute between two players caused because of a touched piece (i.e. the opponents do not agree that the player said “j’adoube” in advance)? Without knowing what actually happened, the Arbiter has 50% possibilities to take a correct decision and 50% to take a wrong one, losing by this way his credibility and the trust of the players.
Of course the Arbiters are human beings and they may make mistakes, but they have to try as much as they can to avoid such problems.
-
- To show responsibility in executing their duties.
The correct time of arriving in the playing hall before the start of the round and the following of the Chief Arbiter’s instructions are parameters that help the smooth running of the tournament.
-
- To show team spirit and cooperate in the best way with the other Arbiters of the competition. An Arbiter’s job in a competition is mainly a team work and the Arbiters shall help and cover each other in any case, so that to avoid, if possible, any problem that arises during the games. The Arbiter has to ask for consultation by the Chief Arbiter, in any case when he does not feel ready to take an important decision regarding the game he observes.
- To study the regulations and be updated for any changes of the laws of chess and the tournament rules.
The Arbiter has to know the Laws of Chess and the Regulations of the tournament, as he has to take a decision immediately when it is needed. The players cannot wait for a long time and the game has to be continued.
-
- To have excellent knowledge of handling the electronic clocks.
It is not acceptable for an Arbiter to let the players waiting for a long time, while trying to fix an electronic clock with wrong time indications during a game.
-
- To follow the dress code.
The Arbiters of a competition shall be dressed properly, helping to the increase of the image of chess as a sport.
Summary of the general duties of an Arbiter
The following general duties are referred to the Arbiters who are acting in Individual or Team Tournaments of any importance and any level, independently of the number of participants:
-
-
- Before the start of the game
-
- An Arbiter should arrive at the playing hall at least thirty (30) minutes before the start of the round. For the first round of the tournament it is advisable to arrive at least one (1) hour before the start of the round.
In very important events the Chief Arbiter may ask for the presence of the Arbiters even earlier before the start of the round.
- The whole playing venue (playing hall, toilets, smoking area, analysis room, bar) and the technical conditions (light, ventilation, air‐condition, enough space for the players, etc.) has to be checked carefully before arrival of players or spectators.
- Check of the equipment (chessboards, pieces, score sheets, pens).
- Arrangement of the tables, chairs, ropes for the playing area, name plates for the players and flags of federations, if needed, or table numbers.
- Check of the electronic clocks, the correct setting of the time control, check of batteries and the correct placement of the clocks.
- For team competitions it is very important to check before the start of the games if the team compositions follow the basic list of players.
-
-
- During the games
-
- Define the unplayed games (if players didn’t arrive on time for their games and have to be forfeited) and inform the Chief Arbiter.
- Regular check of the electronic clocks by using the time control sheets (every thirty minutes) and of the score sheets and the number of moves written.
- Discrete control of the players, if leaving the playing area for an unusual number of times, for their contact with other players, spectators and other persons,
- Observation of all the games, especially when there is time trouble, with the help of an assistant, if needed.
- Carefully check of claims by the players, together with the Chief Arbiter, if needed, before taking any decision.
- At the end of the game check of the recorded result by both players and check of the score sheets to be signed by both players.
- Update the results sheet by recording the result of every finished game.
-
-
- After the end of the round
-
- Thorough check of the results of all the games, by counterchecking of the score sheets and the results sheet or the game protocols (in team events) and forward it to the Chief Arbiter.
- Arrangement of all chess boards and the other equipment (pieces, score sheets, pens, clocks), to be ready for the next round.
The Chief Arbiter is responsible for the full control of the competition and for the correct application of the Laws of Chess and the Tournament Regulations. He shall take care of all technical matters and ensure the best conditions for the players. He has to manage the available arbiters and assigns their duties and responsibilities. He is responsible for the smooth running of the competition and he has the responsibility of taking decisions in every case or incident during the games. He has to try to settle all arising disputes before they are forwarded to the Appeals Commission.
Only in his absence these responsibilities go to the Deputy Chief Arbiter.
After the end of the competition the Chief Arbiter submits in due course his report to the organizing body (FIDE, Continental Federations, National Federation, etc.), in which he includes
- the list of participants
- all pairings and results
- the final standings
- the list of arbiters
- any norm reports and certificates
- a report about any incident that happened during the games
- any appeal that was submitted and the decision taken
- and everything else important for the future organization of the event.
The successful arbitration during the games plays a very significant role in the success of the event.
Example of a “Time Control Sheet”:
|
games started at: |
Check at: |
|
games started at: |
Check at: |
||||||||
|
board |
Time 1 |
Time 2 |
+ |
move |
dif |
|
board |
Time 1 |
Time 2 |
+ |
move |
dif |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Explanation
Example: the games are played with time control: 90 min/40 moves + 30 min/rest of game +30sec/move increment from move 1
Games started at 18:00 and at 18:30 we check clocks. Time game started 18:00 so 90+90 =180 mins;
30 min passed from the beginning: 180-30=150
|
games started at: |
18:00 |
check at: |
18:30 |
||
|
board |
Time 1 |
Time 2 |
+ |
move |
dif |
|
1 |
80 |
85 |
165 |
15 |
150 |
|
2 |
90 |
70 |
160 |
10 |
150 |
|
3 |
87 |
79 |
166 |
16 |
150 |
|
4 |
65 |
90 |
155 |
5 |
150 |
|
|
|
|
|
|
|
In the column “board” write the board’s number
In the column “Time 1” write the white colour player’s time (in minutes) In the column “Time 2” write the black colour player’s time (in minutes) In the column “+” write the sum of “Time 1” + ”Time 2”
In the column “move” the number of the last move that has been played last on this board
In the column “dif” write the difference of column “+” minus “move”
The “dif” values should be equal to: (initial time) minus (time passed until the time of check). In our example is: 90+90–30 = 150. If we find a different value of ± 2 we have to check thoroughly: if players missed of pressing the clock for some moves
- if players have missed pressing the clock for some moves
- if clocks settings are correct
- if there is a clock malfunction.
Our Commitee
Consectetur adipisicing elit sed do ei usmod tempor incididunt.enim ad minim veniam, quis nostrud exer citation ullamco laboris nisi ut aliquip ex ea commodo

Mr. Muzad
Advisor

Robert Khaled
Member




